A stream of a positively charged particles and velocity is deflected by an electric field .Theelectric field exists in a region of 10 cm along x direction. Due to the electric field, the deflection of the charge particles in the y direction is _____mm.
The correct Answer is:B
To solve the problem, we need to find the deflection of positively charged particles in the y-direction due to an electric field. Let's break down the solution step by step.
Step 1: Identify the Given Values
- Charge-to-mass ratio: qm=2×1011C/kg
- Velocity of particles: →v=3×107^im/s
- Electric field: →E=1.8^jkV/m=1.8×103^jV/m
- Length of the region with electric field: x=10cm=0.1m
Step 2: Calculate the Time of Flight
The time t taken to travel through the electric field can be calculated using the formula:
t=xvx
Substituting the values:
t=0.1m3×107m/s=13×108s
Step 3: Calculate the Acceleration in the y-direction
The acceleration ay experienced by the charged particles due to the electric field can be calculated using:
ay=qEm
Using the charge-to-mass ratio:
ay=qmE=(2×1011C/kg)(1.8×103V/m)
Calculating this gives:
ay=3.6×1014m/s2
Step 4: Calculate the Deflection in the y-direction
Using the kinematic equation for displacement in the y-direction:
y=uit+12ayt2
Since the initial velocity in the y-direction ui=0:
y=12ayt2
Substituting the values of ay and t:
y=12(3.6×1014m/s2)(13×108s)2
Calculating t2:
t2=(13×108)2=19×1016s2
Now substituting this back into the equation for y:
y=12(3.6×1014)(19×1016)
y=3.6×101418×1016=2×10−2m=0.02m=20mm
Final Answer
The deflection of the charged particles in the y-direction is 20 mm.
- Charge-to-mass ratio: qm=2×1011C/kg
- Velocity of particles: →v=3×107^im/s
- Electric field: →E=1.8^jkV/m=1.8×103^jV/m
- Length of the region with electric field: x=10cm=0.1m
The time t taken to travel through the electric field can be calculated using the formula:
t=xvx
Substituting the values:
t=0.1m3×107m/s=13×108s
The acceleration ay experienced by the charged particles due to the electric field can be calculated using:
ay=qEm
Using the charge-to-mass ratio:
ay=qmE=(2×1011C/kg)(1.8×103V/m)
Calculating this gives:
ay=3.6×1014m/s2
Using the kinematic equation for displacement in the y-direction:
y=uit+12ayt2
Since the initial velocity in the y-direction ui=0:
y=12ayt2
Substituting the values of ay and t:
y=12(3.6×1014m/s2)(13×108s)2
Calculating t2:
t2=(13×108)2=19×1016s2
Now substituting this back into the equation for y:
y=12(3.6×1014)(19×1016)
y=3.6×101418×1016=2×10−2m=0.02m=20mm
The deflection of the charged particles in the y-direction is 20 mm.
Topper's Solved these Questions
Similar Questions
A mass of 10kg is suspended vertically by a rope of length 5 m from the roof. A force of 30 N is applied at the middle point of rope in horizontal direction .The angle made by upper half of the rope with vertical is θ=tan−1(x×10−1). The value of x is _______
(Given , g =10m/s2)
View SolutionThe figure shows the path of a positively charged particle 1 through a rectangualr region of unifrom electric field as shown in the figure. What is the direction of electric field and the direction of deflection of particles 2,3 and 4 ?
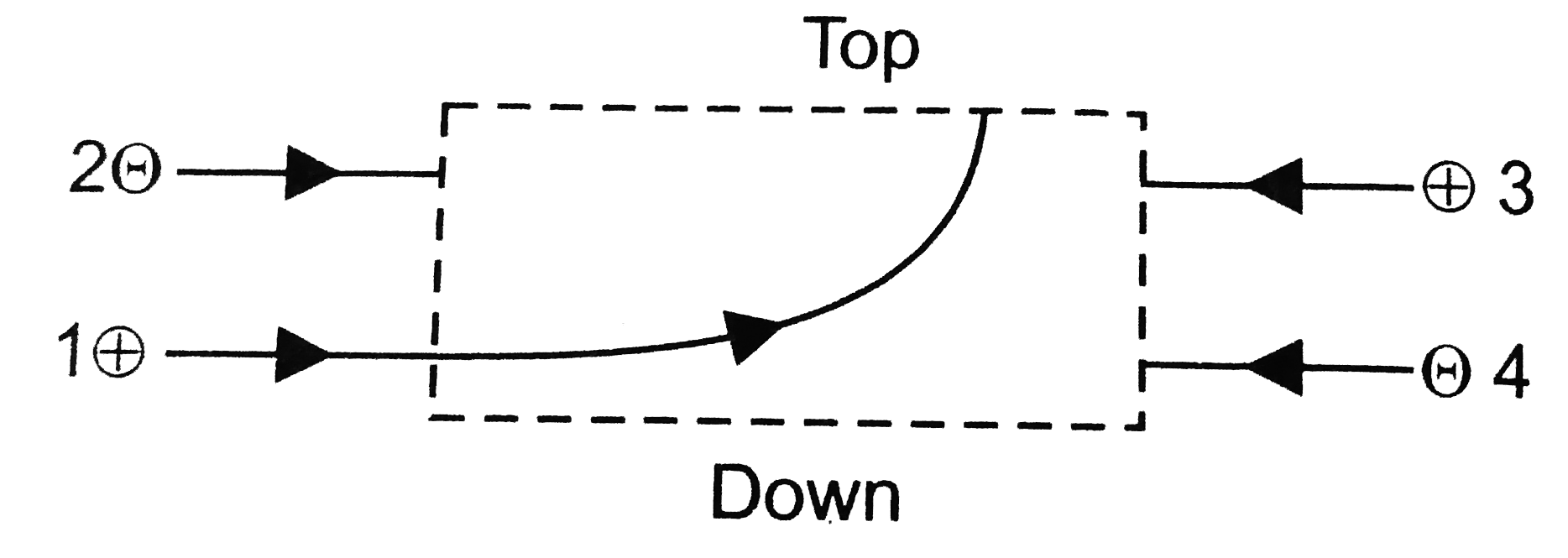
View Solutionएक इलेक्ट्रान धारामें इलेक्ट्रान को वेना 2.0×107 मी/से, 1.6×103 वोल्ट/मी के वैद्युत क्षेत्र के लंबवत दिशा में 10 सेमी चलने में 3.4 मिमी विक्षेपित को जाता है । इलेक्ट्रान का विशिष्ट आवेश (e /m ) ज्ञात कीजिए ।
View SolutionAn electron having mass 9.1×10−11 kg, charge 1.6×10−19 C and moving with the velocity of 106 m/s enters a region where magnetic field exists. If it describes a circle of radius 0.2 m then the intensity of magnetic field must be _________ ×10−5T
View SolutionA charged particle of mass 3 xx 10^(-6) kg is held stationary in spacing in an electric field of strength 10^(6) NC^(-1) directed vertically downwards. The charge on the particle is ( g = 10 ms^(-2))
View SolutionA proton is moving with a velocity of 5 xx 10^(5) m//s along the Y-direction. It is acted upon by an electric field of intensity 10^(5) V/m along the X-direction and a magnetic field of 1 Wb//m^(2) along the Z-direction. Then the Lorentz force on the particle is :
View SolutionThe electric field due to a proton of charge 1.6×10−19C at a distance 1 A (10−10m) from it is
View SolutionA charged particle with a velocity 2 xx 10^(3) m s^(-1) passes undeflected through electric field and magnetic fields in the mutually perpendicular directions. The magnetic field is 1.5 T. The magnitude of electric field will be
View SolutionIf particle of charge 10^(-12) coulomb moving along the hatx - direction with a velocity 10^(5)m//s experiences a force of 10^(-10) newton in haty - direction due to magnetic field, then the minimum magnetic field is
View SolutionTwo charged particles are attached to an x axis: Particle 1 of charge -4.00 xx 10^(-7) C is at position x = -5.00 cm and particle 2 of charge +4.00 xx 10^(-7)C is at position x = 10.cm. Midway between the particles, what is their net electric field in unit-vector notation ?
View Solutionविद्युत क्षेत्र 3×10−10Vm−1 में अनुगमन वेग 7.5×10−4ms−1 वाले आवेशित कण की चलायमानता (Mobility) है
View Solution32×10−19 कूलॉम आवेश वाला एक α- कण 500 न्यूटन/एम्पियर-मी तीव्रता वाले चुाजकीय क्षेत्र में 100 मी/से के वेग से क्षेत्र की दिशा में प्रवेश करता है। कण पर लगने वाला बल है
View SolutionThe figure shows the path of a positively charged particle 1 through a rectangualr region of unifrom electric field as shown in the figure. What is the direction of electric field and the direction of deflection of particles 2,3 and 4 ?

View SolutionA particle of mass 2 xx 10^(-3) kg, charge 4 xx 10^(-3)C enters in an electric field of 5 V//m , then its kinetic energy after 10 s is
View SolutionIn a region, an electric field →E=5×103ˆjNC−1 and a magnetic field of →B=0.1ˆKT are applied. A beam of charged particles are projected along X-direction. Find the velocity of charged particles which move an deflected in this crossed fields.
View SolutionA charged particle with a velocity 2×103ms−1 passes undeflected through electric field and magnetic fields in mutually perpendicular directions. The magnetic field is 1.5 T. The magnitude of electric field will be
View Solution
