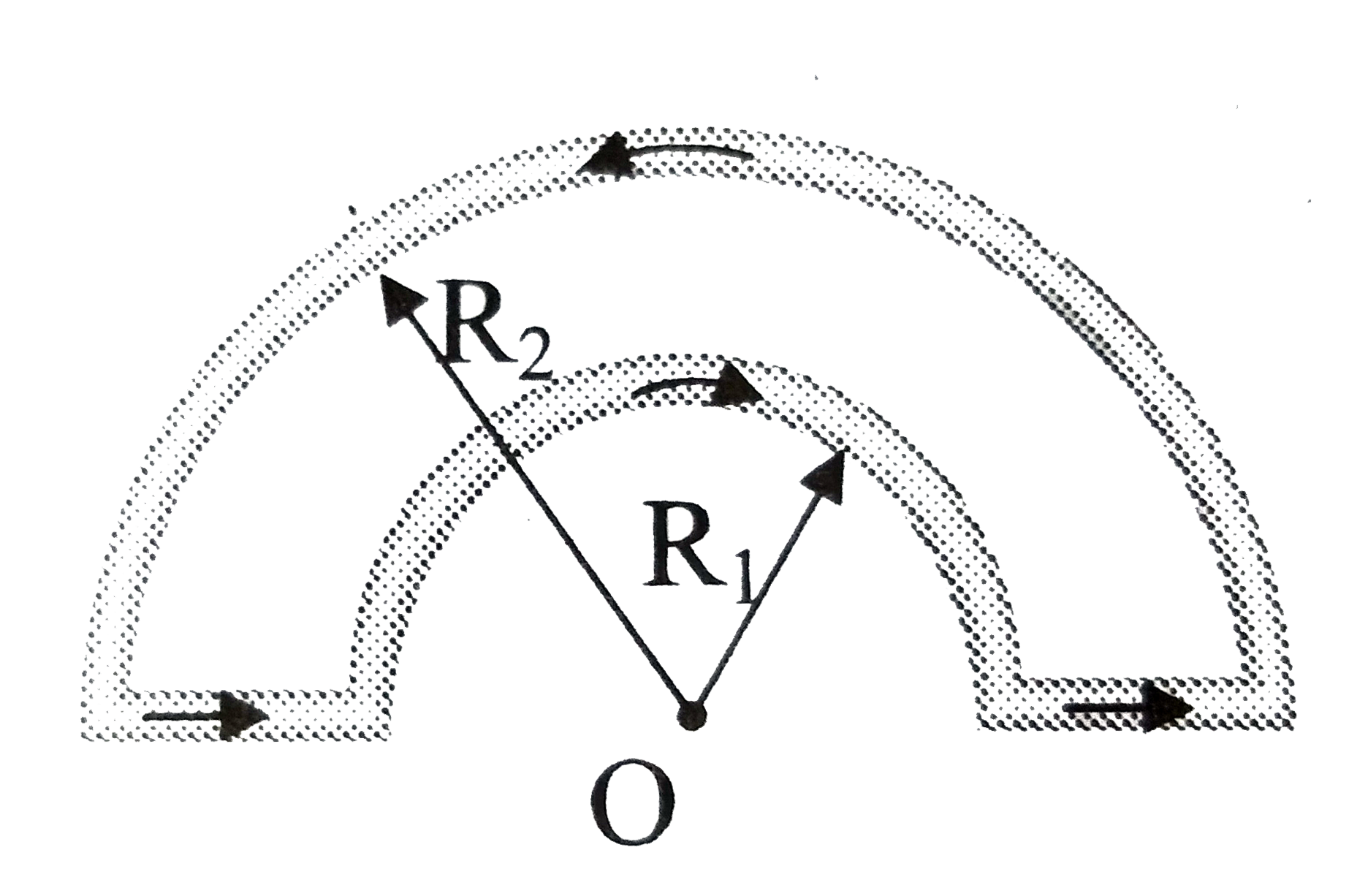
The magnetic induction at the centre O in the figure shown in
A
μ0I4(1R1−1R2)
B
μ0I4(1R1+1R2)
C
μ0I4(R1−R2)
D
μ0I4(R1+R2)
Video Solution
Text Solution
The correct Answer is:A
B due to outer semi circular ring -
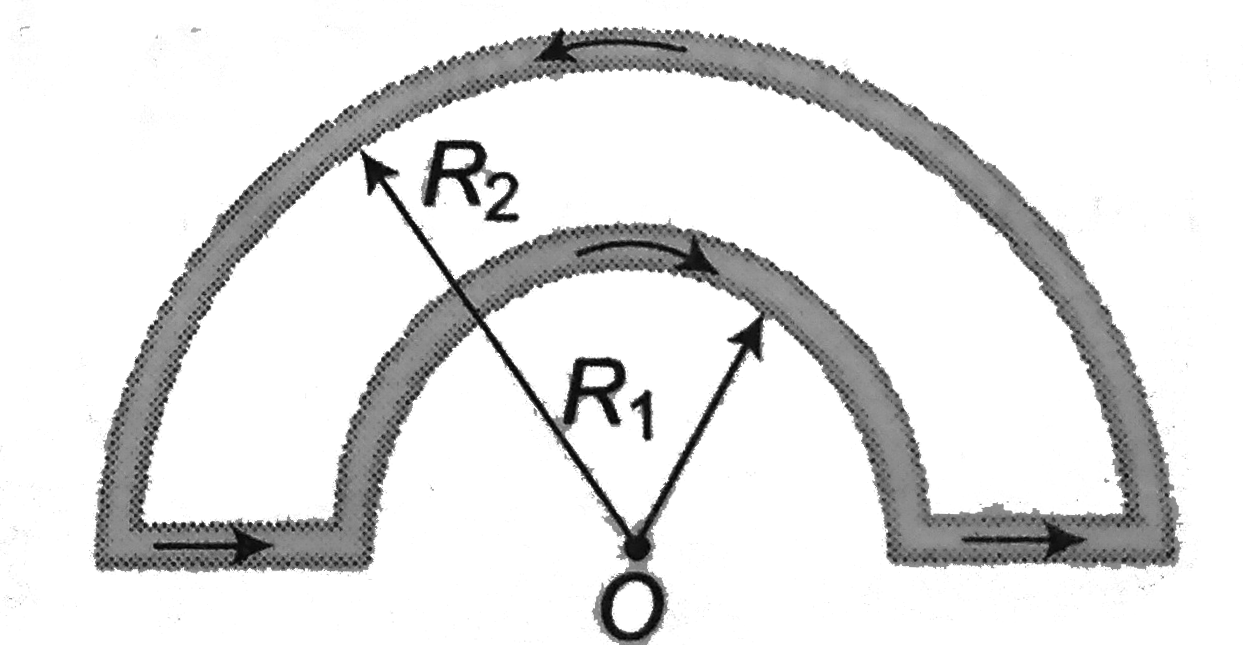
B1=μ0I4π.θR=μ0I4R2π×π=μ0I4R2
B due to inner semi circular ring -
B2=μ0I4R1 (inside)
Total field B−B2−B1=μ0I4(1R1−1R2) (inside)
|
Similar Questions
Knowledge Check
The magnetic induction at centre O Fig.
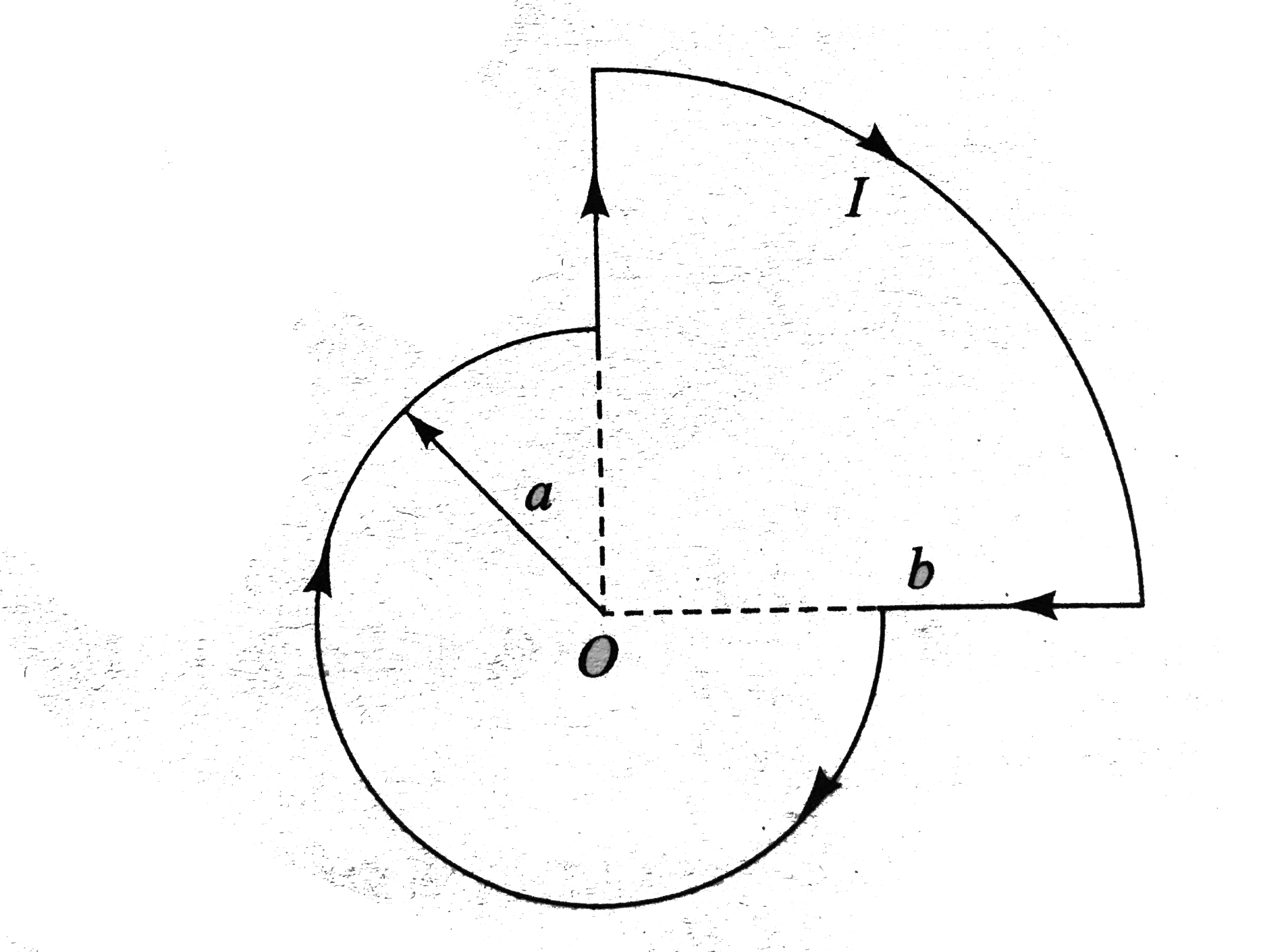 Aμ0I2a+μ0I2b⊗B3μ0I8a+μ0I8b⊗C3μ0I8a−μ0I8b⊗D3μ0I8a+μ0I8b⊙
Aμ0I2a+μ0I2b⊗B3μ0I8a+μ0I8b⊗C3μ0I8a−μ0I8b⊗D3μ0I8a+μ0I8b⊙The magnetic induction at center O as shown in the figure is
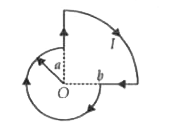 Aμ0I2a+μ0I2b⊗B3μ0I8a+μ0I8b⊗C3μ0I8a−μ0I8b⊗D3μ0I8a+μ0I8b⊙
Aμ0I2a+μ0I2b⊗B3μ0I8a+μ0I8b⊗C3μ0I8a−μ0I8b⊗D3μ0I8a+μ0I8b⊙Find the magnetic induction at the origin in the figure shown.
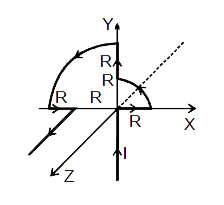 Aμ0I2R(34ˆk+1πˆj)Bμ0I4R(34ˆk+1πˆj)Cμ0I3R(34ˆk+1πˆj)Dμ0I4R(12ˆk+1πˆj)
Aμ0I2R(34ˆk+1πˆj)Bμ0I4R(34ˆk+1πˆj)Cμ0I3R(34ˆk+1πˆj)Dμ0I4R(12ˆk+1πˆj)
The magnetic induction at centre O in the following figure will be
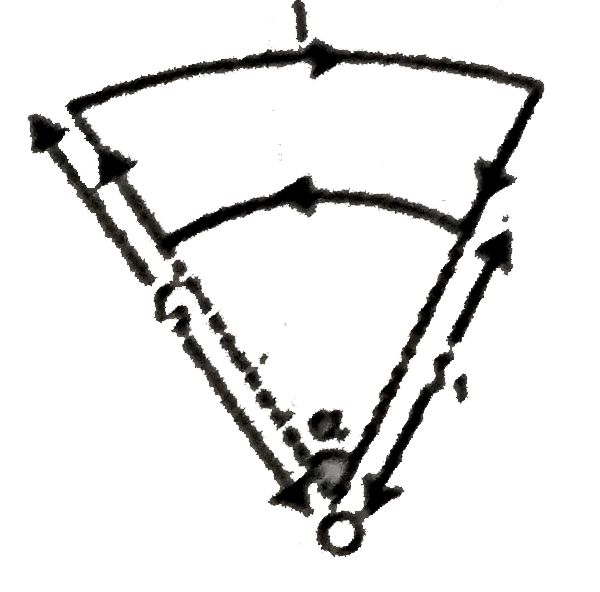
View SolutionThe magnetic field at the centre O of the are shown in the figure is

View SolutionMagnetic induction at the point O in the adjacent figure is
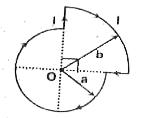
View SolutionA current I is flowing in a hexgonal coil of side a figure. The magnetic field induction at the centre O of the coil will be
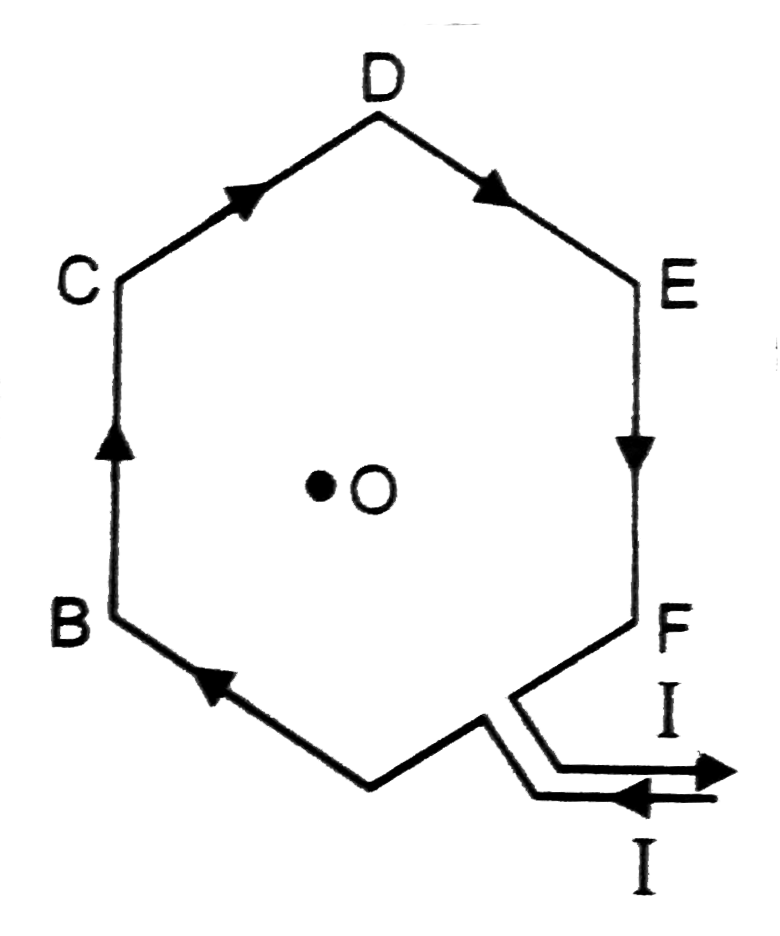
View SolutionA current I is flowing in an octagonal coil of side a figure. The magnetic field induction at the centre O of the coil will be
View Solution