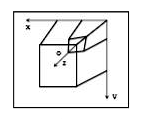
A smaller cube with side b (depicted by dashed lines) is excised from a bigger uniform cube with side a as shown below such that both cubes have a common vertex P. Let X = a/b. If the centre of mass of the remaining solid is at the vertex O of smaller cube then X satisfies.

A

$x^{3} - x^{2} - x - 1 = 0$

B

$x^{2} - x - 1 = 0$

C

$x^{3} + x^{2} - x - 1 = 0$

D

$x^{3} - x^{2} - x + 1 = 0$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

$X_{C M} = \frac{ρ (a^{3}) (\frac{a}{2}) - ρ (b^{3}) (\frac{b}{2})}{ρ (a^{3}) - ρ (b^{3})}$
We will consider removed mass as a negative mass
$b = \frac{ρ (\frac{a^{4}}{2}) - ρ (\frac{b^{4}}{2})}{ρ (a^{3}) - ρ (b^{3})}$
$a^{3} b - b^{4} = \frac{a^{4}}{2} - \frac{b^{4}}{2}$
$2 a^{3} b - 2 b^{4} = a^{4} - b^{4}$
Put a= bx,
$2 b^{4} x^{3} - 2 b^{4} = b^{4} x^{4} - b^{4}$
$2 x^{3} - 1 = x^{4}$
$2 x^{3} - 2 + 1 = x^{4}$
$2 (x^{3} - 1) = (x^{2} + 1) (x^{2} - 1)$
$2 (x - 1) (x^{2} + 1 + x) = (x - 1) (x + 1) (x^{2} + 1)$
$2 x^{2} + 2 + 2 x = x^{3} + x + x^{2} + 1$
$x^{3} - x^{2} - x - 1 = 0$

Updated on:21/07/2023

|

Class 11 PHYSICS RIGID BODY DYNAMICS

Similar Questions

Recommended Questions

Home
Ask Doubt
Profile