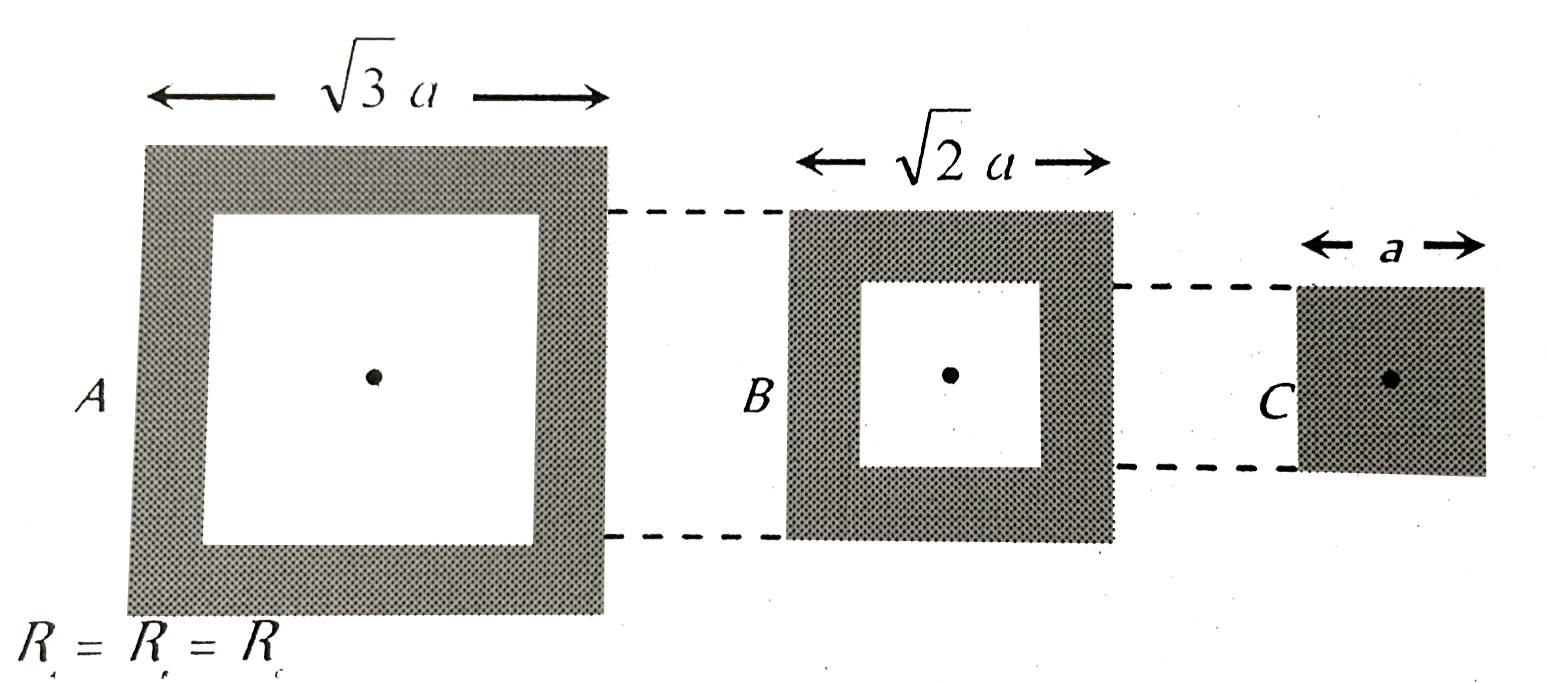
Following figure shows cross-section through three long conductors of the same length and material, with square cross-section of edge lengths as shown. Conductor $B$ will snugly within conductor $B$ . Relationship between their end to end resistance is

$R_{A} = R_{B} = R_{C}$

$R_{A} > R_{B} > R_{C}$

$R_{A} < R_{B} < R_{c}$

Information is not sufficient

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Updated on:21/07/2023

Following figure shows cross-sections through three long conductors of the same length and material, with square cross-section of edge lengths as shown. Conductor B will fit snugly within conductor A , and conductor C will fit snugly within conductor B . Relationship between their end to end resistance is

A $R = R = R$
B $R > R > R$
C $R < R < R$
DInformation is not sufficient
Following figure shows cross-sections through three long conductors of the same length and material, with square cross-section of edge lengths as shown. Conductor B will fit snugly within conductor A, and conductor C will fit snugly within conductor B. Relationship between their end to end resistance is

A $R_{A} = R_{B} = R_{C}$
B $R_{A} > R_{b} > R_{C}$
C $R_{A} < R_{B} < R$
DInformation is not sufficient
How is electrical conductance of a conductor related with length and area a cross section of the conductor
A $G = l . a . k^{- 1}$
B $G = k . i . a^{- 1}$
C $G = k . a . i^{- 1}$
D $G = k . i . a^{- 2}$