In order to increase the resistance of a given wire of uniform cross section to four times its value, a fraction of its length is stretched uniformly till the full length of the wire becoes times the original length. What is the value of this fraction?
A
B
C
D
Video Solution
Text Solution
The correct Answer is:A
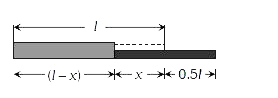
Since, new Resistance = 4R
where
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - ERRORLESSCHAPTER - CURRENT ELECTRICITYEXERCISE - NCERT BASED QUESTIONS (Grouping of Resistances)69videos
CURRENT ELECTRICITY
- BOOK - ERRORLESSCHAPTER - CURRENT ELECTRICITYEXERCISE - NCERT BASED QUESTIONS (KIRCHHOFF.S LAW, CELLS)53videos
CURRENT ELECTRICITY
- BOOK - ERRORLESSCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - Assertion and Reason23videos
ATOMIC AND NUCLEAR PHYSICS
- BOOK - ERRORLESSCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSERTION & REASON20videos
ELECTROMAGNETIC INDUCTION
Similar Questions
Knowledge Check
- Question 1 - Select One
In order to increase the resistance of a given wire of unknown of uniform cross section to four times its value, a fraction of its length is stretched uniformly till the full length of the wire becoes 32 times the original length. What is the value of this fraction?
A14B18C116D16 - Question 2 - Select One
A wire of resistance 12Ω is stretched uniformly till its length becomes three times original length. The change in resistance of wire is
A96ΩB108ΩC150ΩD208Ω - Question 3 - Select One
In order to quadruple the resistance of a uniform wire, a part of its length was uniformly stretched till the final length of the entire wire was 1.5 times the original length, the part of the wire was fraction equal to
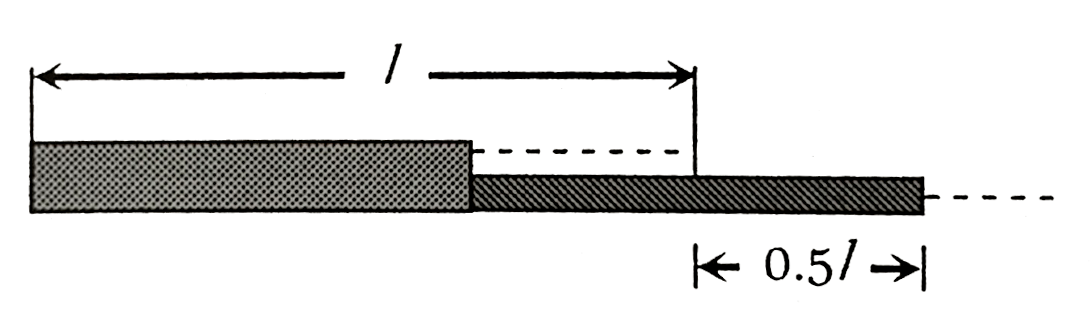 A1/8B1/6C1/10D1/4
A1/8B1/6C1/10D1/4
A copper wire of resistance R0 is strerched till its length is increased to n times of its original length. What will be its new resistance?
View SolutionIf a wire of resistance 25Ω is uniformly stretched until its length becomes three times its original length then its new resistance is
View SolutionIf length of a metallic wire becomes n times, its resistance becomes
View SolutionThe resistance of a wire is r ohm. If it is melted and stretched to n times its original length , its new resistance will be
View SolutionThe resistance of wire of length l and area of cross-section a is x ohm. If the wire is stretched to double its length, its resistance would become:
View Solution
