Assertion: The graph of total energy of a particle in SHM with respect to position is a straight line with zero slope.
Reason: Total energy of particle in SHM remains constant throughout its motion.
A
If both assertion and reason are true and the reason is the correct explanation of the assertion.
B
If both assertion and reason are true but reason is not the correct explanation of the assertion.
C
If assertion is true but reason is false.
D
If the assertion and reason both are false
Video Solution
Text Solution
The correct Answer is:A
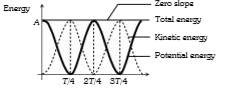
The total energy of S.H.M.=Kinetic energy of particle + potential energy of particle.
The variation of total energy of the particle in SHM with time is shown in a graph.
|
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - ERRORLESSCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - PAST YEARS QUESTIONS
28 Videos
- ROTATIONAL MOTIONBOOK - ERRORLESSCHAPTER - ROTATIONAL MOTIONEXERCISE - Assertion & Reason
25 Videos
- SURFACE TENSIONBOOK - ERRORLESSCHAPTER - SURFACE TENSIONEXERCISE - ASSERTION & REASON
11 Videos
Similar Questions
Knowledge Check
Assertion : The graph of total energy of a particle in SHM w.r.t. position is a line with zero slope
Reason : Total energy of particle in SHM remain constant throughout its motionAIf both assertion and reason are true and the reasopn is a true explanation of the assertionBIf both assertion and reason are true and but the reason is not the correct explanation of assertionCIf the assertion is true but reason is falseDIf both the assertion and reason are falseSTATEMENT-1 : The graph of total energy of a particle in S.H.M w.r.t. position is a straight line with zero slope. STATEMENT-2 : Total graph of total energy of a particle in S.H.M. remains constant throughout its motion.
AStatement-1 is True, Statement-2 is True, Statement-2 is a correct explanation for Statement-1.BStatement-1 is True, Statement-2 is True, Statement-2 is NOT a correct explanation for Statement-1.CStatement-1 is True, Statement-2 is False.DStatement-1 is False, Statement-2 is True.The potential energy of a particle performing S.H.M. at extreme position is
AminimumBmaximumCremains constantD1/2mω2x2
The value of total mechanical energy of a particle is SHM is
View SolutionThe total energy of particle executing SHM is proportional to
View SolutionThe total energy of particle executing SHM is proportional to
View SolutionThe potential energy of a particle performing S.H.M. at mean position is
View SolutionThe potential energy of a particle performing S.H.M. at mean position is
View Solution
