A metre stick of mass 400 g is pivoted at one end and displaced through an angle . The increase in its potential energy is
2 J
3 J
0 J
1 J
The correct Answer is:D
To solve the problem, we need to calculate the increase in potential energy of a meter stick that is pivoted at one end and displaced through an angle of 60 degrees. Here’s a step-by-step solution:
Step 1: Understand the System
The meter stick has a mass of 400 g (which is 0.4 kg) and is pivoted at one end. When it is displaced to an angle of 60 degrees, the center of mass of the stick will rise to a new height.
Step 2: Calculate the Initial Height of the Center of Mass
The center of mass of a uniform meter stick is located at its midpoint, which is at a distance of L2 from the pivot point. For a meter stick (L = 1 m):
- Initial height of the center of mass (h_initial) = 12 m = 0.5 m.
Step 3: Calculate the Final Height of the Center of Mass
When the stick is tilted at an angle of 60 degrees, the height of the center of mass can be calculated using trigonometry:
- The height of the center of mass after displacement (h_final) = L2×cos(60∘).
- Since cos(60∘)=12:
hfinal=12×12=14m=0.25m.
Step 4: Calculate the Increase in Height
The increase in height (Δh) of the center of mass when the stick is tilted is:
Δh=hfinal−hinitial=0.25m−0.5m=−0.25m.
(Note: The height decreases, indicating a negative change, but we need the absolute value for potential energy calculation.)
Step 5: Calculate the Change in Potential Energy
Potential energy (PE) is given by the formula:
PE=mgh,
where:
- m = mass of the stick = 0.4 kg,
- g = acceleration due to gravity = 10 m/s²,
- h = change in height.
The initial potential energy (PE_initial):
PEinitial=mghinitial=0.4×10×0.5=2J.
The final potential energy (PE_final):
PEfinal=mghfinal=0.4×10×0.25=1J.
Step 6: Calculate the Increase in Potential Energy
The increase in potential energy (ΔPE) is:
ΔPE=PEfinal−PEinitial=1J−2J=−1J.
Since we are interested in the increase, we take the absolute value:
ΔPE=1J.
Final Answer
The increase in potential energy is 1 Joule.
---
The meter stick has a mass of 400 g (which is 0.4 kg) and is pivoted at one end. When it is displaced to an angle of 60 degrees, the center of mass of the stick will rise to a new height.
The center of mass of a uniform meter stick is located at its midpoint, which is at a distance of L2 from the pivot point. For a meter stick (L = 1 m):
- Initial height of the center of mass (h_initial) = 12 m = 0.5 m.
When the stick is tilted at an angle of 60 degrees, the height of the center of mass can be calculated using trigonometry:
- The height of the center of mass after displacement (h_final) = L2×cos(60∘).
- Since cos(60∘)=12:
hfinal=12×12=14m=0.25m.
The increase in height (Δh) of the center of mass when the stick is tilted is:
Δh=hfinal−hinitial=0.25m−0.5m=−0.25m.
(Note: The height decreases, indicating a negative change, but we need the absolute value for potential energy calculation.)
Potential energy (PE) is given by the formula:
PE=mgh,
where:
- m = mass of the stick = 0.4 kg,
- g = acceleration due to gravity = 10 m/s²,
- h = change in height.
PEinitial=mghinitial=0.4×10×0.5=2J.
PEfinal=mghfinal=0.4×10×0.25=1J.
The increase in potential energy (ΔPE) is:
ΔPE=PEfinal−PEinitial=1J−2J=−1J.
Since we are interested in the increase, we take the absolute value:
ΔPE=1J.
The increase in potential energy is 1 Joule.
Topper's Solved these Questions
- ROTATIONAL MOTIONBOOK - ERRORLESSCHAPTER - ROTATIONAL MOTIONEXERCISE - NCERT BASED QUESTIONS (Rolling on Inclined Plane)
54 Videos
- ROTATIONAL MOTIONBOOK - ERRORLESSCHAPTER - ROTATIONAL MOTIONEXERCISE - PAST YEARS QUESTIONS
70 Videos
- ROTATIONAL MOTIONBOOK - ERRORLESSCHAPTER - ROTATIONAL MOTIONEXERCISE - NCERT BASED QUESTIONS (Angular Momentum)
52 Videos
- NEWTON'S LAWS OF MOTIONBOOK - ERRORLESSCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - ASSERTION & REASON
18 Videos
- SIMPLE HARMONIC MOTIONBOOK - ERRORLESSCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Assertion & Reason
15 Videos
Similar Questions
A uniform meter scale of mass 2kg is suspended from one end.If it is displaced through an angle of 600 from the vertical,the increase in its potential energy is ?
View SolutionA metre stick weighing 240 g is pivoted at its upper end in such a way that it can freely rotate in a vertical plane through this end figure. A particle of mass 100 g is attached to the upper end of the stick through a light sting of length 1 m. Initially the rod is kept veritcal and the string horizontal when the system is released from rest. The particle colides with the lower end of the stick and sticks there. Find the maximum angle through which the stick will rise.
View Solution
Knowledge Check
A stick of mass m and length l is pivoted at one end and is displaced through an angle θ. The increase in potential energy is
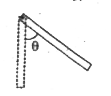 Amg(l)2(1−cosθ)Bmg(l)2(1+cosθ)Cmg(l)2(1−sinθ)Dmg(l)2(1+sinθ)
Amg(l)2(1−cosθ)Bmg(l)2(1+cosθ)Cmg(l)2(1−sinθ)Dmg(l)2(1+sinθ)A metre stick weighing 600 g, is displaced through an angle of 60∘ in vertical plane as shown. The change in its potential energy is (g=10ms−2)
 A1.5 JB15 JC30 JD45 J
A1.5 JB15 JC30 JD45 JA long wire hangs vertically with its upper end clamped, when a torque of 2 Nm is applied to the free end, it is twisted through an angle of 30∘. Then the potential energy of the twisted wire is
Aπ joulesBπ/3 joulesCπ/6 joulesDπ/4 joules
A uniform rod pivoted at its upper end hangs vertically. It is displaced through anangle of 60∘ and then released. Find the magnitude of the force acting on a particle of mass dm at the tip of the rod when the rod makes an angle of 37∘ with the vertical.
View Solution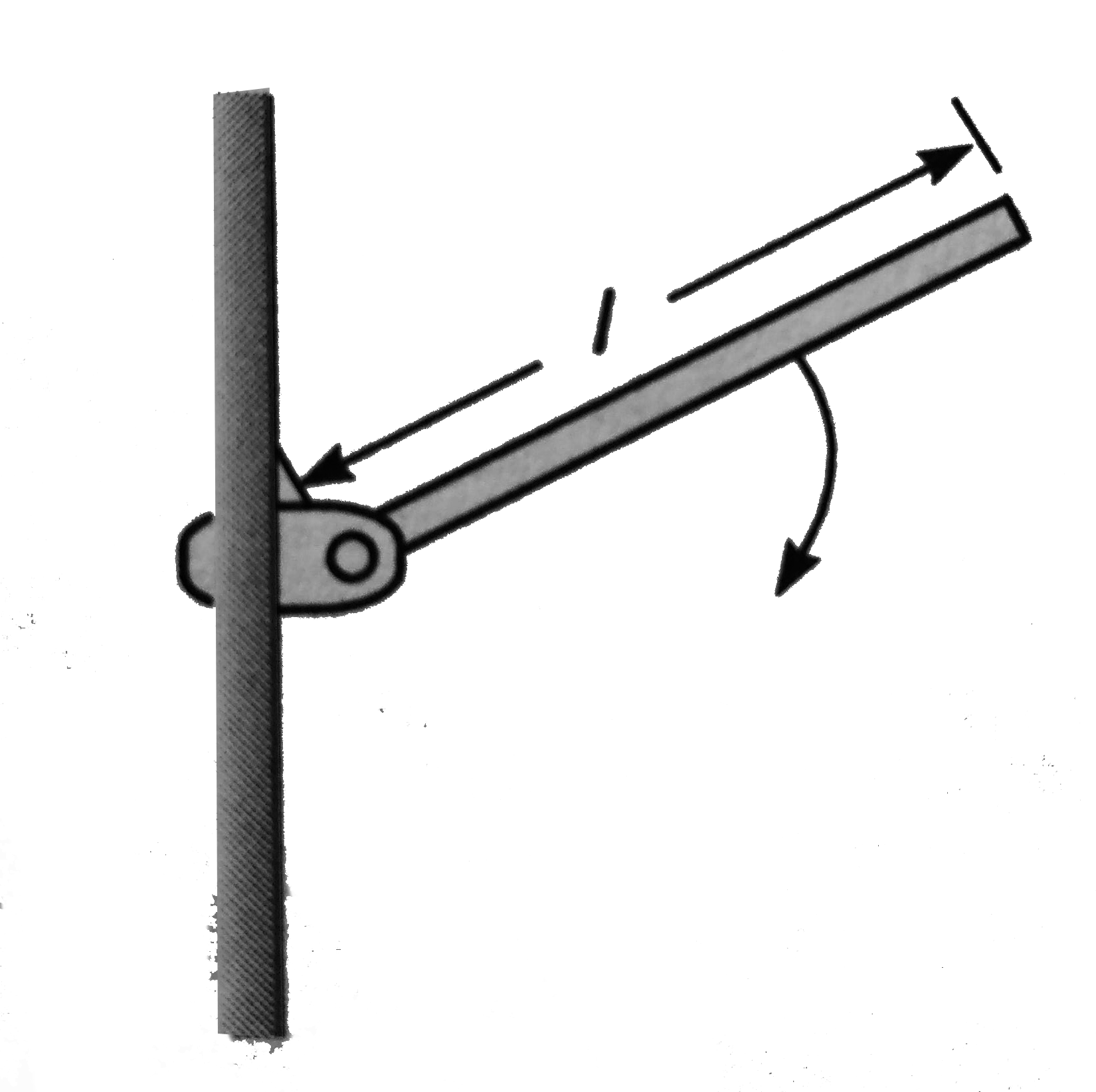
A thin plank of mass M and length l is pivoted at one end. The plank is releaed at 60∘ from the vertical. What is the magnitude and direction of the force on the pivot when the plank is horizontal?
View SolutionThe upper end of a wire of radius 4mm and lengh 100cm is clamped and its other end if twiced through an angle of 60∘ the angle of shear si
View SolutionA ball of mass 0.1 kg is suspended by a string. It is displaced through an angle of 60∘ and left. When the ball passes through the mean position, the tension in the string is
View SolutionAn α− particle is accelerated through a potential difference of 200V. The increase in its kinetic energy is
View Solution
