A uniform thin rod of length 2L and mass m lies on a horizontal table. A horizontal impulse J is given to the rod at one red. There is no friction. The total kinetic energy of the rod just after the impulse will be

$\frac{J^{2}}{2 m}$

$\frac{J^{2}}{m}$

$\frac{2 J^{2}}{m}$

$\frac{6 J^{2}}{m}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

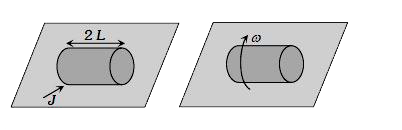
$J = m v ... (i)$
When v is the velocity of centre of mass after impulse rod get angular velocity $ω$
Angular impulse $= I ω$
$\Rightarrow J \times L = \frac{m {(2 L)}^{2}}{12} \times ω$
$\Rightarrow J = \frac{m L ω}{3} \Rightarrow ω = \frac{3 J}{m L}$
From equation (i) $v = \frac{J}{m}$
Kinetic energy = KE = $\frac{1}{2} m v^{2} + \frac{1}{2} I ω^{2}$
$\Rightarrow K E = \frac{1}{2} m \frac{J^{2}}{m^{2}} + \frac{1}{2} \times \frac{m \times 4 L^{2}}{12} \times \frac{9 J^{2}}{m^{2} L^{2}}$
$\Rightarrow K E = \frac{2 J^{2}}{m}$

Updated on:21/07/2023

Class 11 PHYSICS WORK, ENERGY AND POWER

Topper's Solved these Questions

Knowledge Check

A uniform rod of length $L$ and mass $M$ is lying on a frictionless horizontal plane and is pivoted at one of its ends as shown in Fig. There is no friction at the pivot. An inelastic ball of mass $m$ is fixed with the rod at a distance $L / 3$ from $O$ . A horizontal impulse $J$ is given to the rod at a distance $2 L / 3$ from $O$ in a direction perpendicular to the rod. Assume that the ball remains in contact with the rod after the collision and impulse $J$ acts for a small time interval $△ t$ . Now answer the following questions:

Find the resulting instantaneous angular velocity of the rod after the impulse.
A $\frac{3 J}{(m + 3 M) L}$
B $\frac{6 J}{(m + 3 M) L}$
C $\frac{3 J}{(3 m + M) L}$
D $\frac{6 J}{(3 m + M) L}$
A uniform rod of length $L$ and mass $M$ is lying on a frictionless horizontal plane and is pivoted at one of its ends as shown in Fig. There is no friction at the pivot. An inelastic ball of mass $m$ is fixed with the rod at a distance $L / 3$ from $O$ . A horizontal impulse $J$ is given to the rod at a distance $2 L / 3$ from $O$ in a direction perpendicular to the rod. Assume that the ball remains in contact with the rod after the collision and impulse $J$ acts for a small time interval $△ t$ . Now answer the following questions:

Find the magnitude of the impulse applied by the during the time interval $△ t$
A $\frac{m J}{(m + 3 M)}$
B $\frac{m J}{(3 m + M)}$
C $\frac{M J}{(m + 3 M)}$
D $\frac{M J}{(3 m + M)}$
A uniform rod of length $L$ and mass $M$ is lying on a frictionless horizontal plane and is pivoted at one of its ends as shown in Fig. There is no friction at the pivot. An inelastic ball of mass $m$ is fixed with the rod at a distance $L / 3$ from $O$ . A horizontal impulse $J$ is given to the rod at a distance $2 L / 3$ from $O$ in a direction perpendicular to the rod. Assume that the ball remains in contact with the rod after the collision and impulse $J$ acts for a small time interval $△ t$ . Now answer the following questions:

Find the impulse acted on the ball during the time $△ t$
A $\frac{2 M J}{(3 m + M)}$
B $\frac{2 M J}{(m + 3 M)}$
C $\frac{2 m J}{(3 m + M)}$
D $\frac{2 m J}{(m + 3 M)}$

ERRORLESS-WORK, ENERGY POWER AND COLLISION-NCERT BASED QUESTIONS (Conservation of Energy and Momentum)

Home
Ask Doubt
Profile

A uniform thin rod of length 2L and mass m lies on a horizontal table. A horizontal impulse J is given to the rod at one red. There is no friction. The total kinetic energy of the rod just after the impulse will be

The correct Answer is:C

J=mv ...(i) When v is the velocity of centre of mass after impulse rod get angular velocity ω Angular impulse =Iω ⇒J×L=m(2L)212×ω ⇒J=mLω3⇒ω=3JmL From equation (i) v=Jm Kinetic energy = KE = 12mv2+12Iω2 ⇒KE=12mJ2m2+12×m×4L212×9J2m2L2 ⇒KE=2J2m

Topper's Solved these Questions

Similar Questions

Knowledge Check

ERRORLESS-WORK, ENERGY POWER AND COLLISION-NCERT BASED QUESTIONS (Conservation of Energy and Momentum)