If pt ne qs, then the pair of equations px + qy = r, sx + ty = u

Shown below are the graphs of the lines $y - 2 x = 0, x + y = 6 and p x + q y = r$

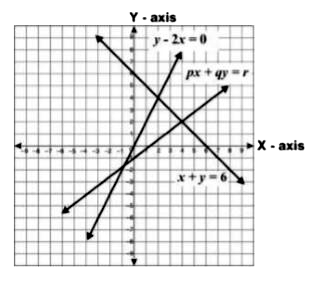
Which of these is the solution for the pair of equations x+y=6 and px+qy=r
Ax=2,y=4
Bx=4, y=2
Cx=3, y=2
DWe cannot say for sure as the values of p and q are not known.
Obtain the condition for the following system of linear equations to has a unique solution
$p x + q y = r$ and $l x + m y = n$ .
Apm = ql
Bpm - ql = 0
Cpm $\neq$ ql
Dnone of these
If the roots of the quadratic equation $p x^{2} + q x + r = 0$ are reciprocal to each other ,then the relation between p and r is
A $r \neq p$
B $r = p$
C $r = q$
DNone of these

If pt $\neq$ qs, then the pair of equations px + qy = r, sx + ty = u