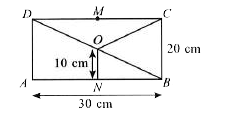
ABCD is a rectangle.If N,O and M are the mid points of AB, BD and CD respectively , then find
(i) area of (δCOD)
(ii) area of (CBNO): area of (ABCD)
i ii250cm2 2:1
i ii150cm2 1:4
i ii100cm2 3:2
i ii150cm2 3:8
The correct Answer is:D
Step by step video, text & image solution for ABCD is a rectangle.If N,O and M are the mid points of AB, BD and CD respectively , then find (i) area of (delta COD) (ii) area of (CBNO): area of (ABCD) by Maths experts to help you in doubts & scoring excellent marks in Class 7 exams.
Updated on:21/07/2023Topper's Solved these Questions
- IMO QUESTION PAPER 2019-20 SET ABOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - IMO QUESTION PAPER 2019-20 SET AEXERCISE - EVERYDAY MATHEMATICS
10 Videos
- IMO QUESTION PAPER 2018-19 SET ABOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - IMO QUESTION PAPER 2018-19 SET AEXERCISE - Logical Reasoning
20 Videos
- IMO QUESTION PAPER 2019-20 SET BBOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - IMO QUESTION PAPER 2019-20 SET BEXERCISE - Achievers section
5 Videos
Similar Questions
Knowledge Check
If ABCD be a rectangle and P, Q, R, S be the mid-points of AB, BC, CD and DA respectively, then the area of the quadrilateral PQRS is equal to
A13 are (ABCD)B34 area (ABCD)C12 area (ABCD)Darea (ABCD)In a rectangle ABCD , E is the mid -point of AB . If AB= 16 m and AD = 6 m , then find ED .
A15 cmB10 cmC12 mD14 mArea of ΔABC=30cm2. D and E are the mid - points of BC and AB respectively. Find A(ΔBDE) :
A10cm2B7.5cm2C15cm2Dnone of these
ABCD is a rhombus and P,Q,R,S are the mid-points of AB,BC,CD,DA respectively. Prove that PQRS is a rectangle.
View SolutionIn a figure, ABCD is a rectangle and P & Q are mid-points of CD and BC respectively. Find ratio of area of shadow region and area of un-shad-owed region?

View SolutionLet ABCD be a parallelogram of area 124cm2. If E and F are the mid-points of sides AB and CD respectively, then find the area of parallelogram AEFD.
View SolutionABCD is a rectangle and M is a point on CD . AC and BM meet at X .

It is given that CM =3MD. Find
(i) area of ΔCXM : area of ΔAXB
(ii) area of ΔBXC : area of rectangle ABCD
View SolutionABCD is a parallelogram, E and F are the mid-points of BC and CD. Find the ratio of area of parallelogram ABCD and ΔAEF
View Solution
