Question

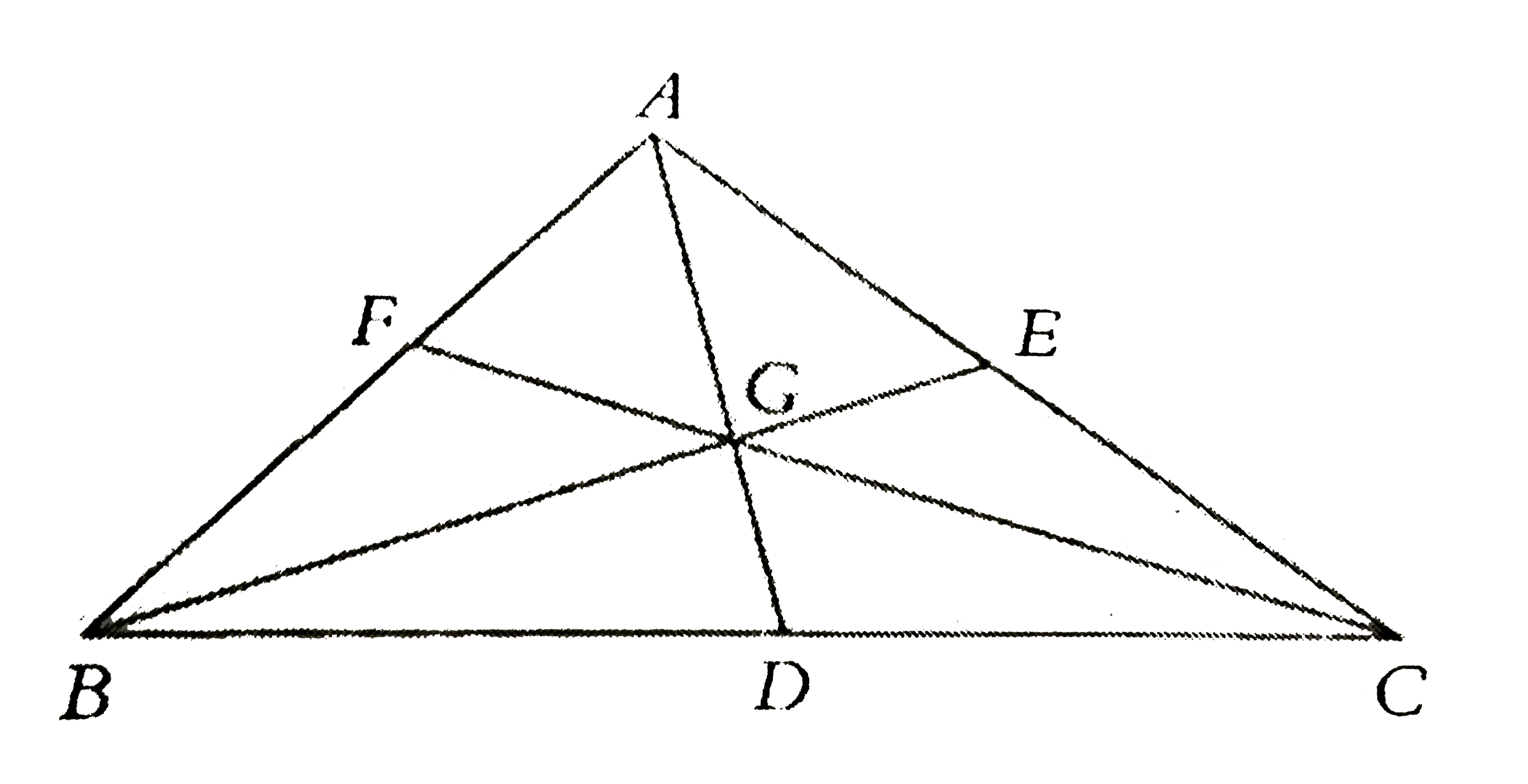
In a $\Delta ABC,AD,BE$ and CF are three medians. The perimeter of $\Delta ABC$ is always.

Options

A. equal to `(bar(AB) + bar(BE) + bar(CF))`
B. greater than `(bar(AD) + bar(BE) + bar(CF))`
C. less than `(bar(AD) + bar(BE)+bar(CF))`
D. None of these

Answer

B

Explanation

To find the relationship between the perimeter of triangle ABC and the lengths of its medians AD, BE, and CF, we can follow these steps:

Step 1: Understand the Medians
In triangle ABC, the medians AD, BE, and CF are the segments that connect each vertex of the triangle to the midpoint of the opposite side.

Step 2: Define the Perimeter
The perimeter of triangle ABC is defined as the sum of the lengths of its sides:
\( P = AB + BC + CA \)

Step 3: Use the Median Length Formula
The length of a median can be calculated using the formula:
\( m_a = \frac{1}{2} \sqrt{2b^2 + 2c^2 - a^2} \)
where \( m_a \) is the median from vertex A to side BC, and \( a, b, c \) are the lengths of sides opposite to vertices A, B, and C respectively.

Step 4: Establish Relationships
Using the triangle inequality, we know that the sum of the lengths of any two sides of a triangle is greater than the length of the third side. Therefore:
1. \( AB + AC > BC \)
2. \( AB + BC > AC \)
3. \( AC + BC > AB \)

Step 5: Relate Medians to Sides
For any triangle, it is known that:
- \( AB + AC > 2AD \)
- \( AB + BC > 2BE \)
- \( AC + BC > 2CF \)

Step 6: Combine the Inequalities
Adding the above inequalities gives:
\( (AB + AC) + (AB + BC) + (AC + BC) > 2(AD + BE + CF) \)
This simplifies to:
\( 2(AB + AC + BC) > 2(AD + BE + CF) \)

Step 7: Divide by 2
Dividing both sides by 2, we get:
\( AB + AC + BC > AD + BE + CF \)

Conclusion
Thus, the perimeter of triangle ABC is always greater than the sum of its medians:
\( P > AD + BE + CF \)