Which apparatus is used to select the charged particles of a particular velocity concerning both electric and magnetic fields ?
Cyclotron
Velocity Filter
Galvanometer
Wooden block
The correct Answer is:B
To solve the question, "Which apparatus is used to select the charged particles of a particular velocity concerning both electric and magnetic fields?", we can follow these steps:
1. Understanding the Question: The question asks for an apparatus that can select charged particles based on their velocity when subjected to both electric and magnetic fields.
2. Identifying the Options: The options provided are:
- Cyclotron
- Velocity filter
- Galvanometer
- Wooden block
3. Analyzing Each Option:
- Cyclotron: This device accelerates charged particles using a magnetic field, but it does not specifically filter particles based on velocity.
- Galvanometer: This is used to detect electric current, not specifically for selecting charged particles based on velocity.
- Wooden Block: This is not relevant to the selection of charged particles.
- Velocity Filter: This apparatus is specifically designed to allow only charged particles of a certain velocity to pass through by using both electric and magnetic fields.
4. Conclusion: The correct answer is the Velocity Filter (or Velocity Selector). This device uses the principles of electric and magnetic fields to filter out charged particles based on their velocity.
Final Answer:
The apparatus used to select charged particles of a particular velocity concerning both electric and magnetic fields is the Velocity Filter.
---
- Cyclotron
- Velocity filter
- Galvanometer
- Wooden block
- Cyclotron: This device accelerates charged particles using a magnetic field, but it does not specifically filter particles based on velocity.
- Galvanometer: This is used to detect electric current, not specifically for selecting charged particles based on velocity.
- Wooden Block: This is not relevant to the selection of charged particles.
- Velocity Filter: This apparatus is specifically designed to allow only charged particles of a certain velocity to pass through by using both electric and magnetic fields.
The apparatus used to select charged particles of a particular velocity concerning both electric and magnetic fields is the Velocity Filter.
Similar Questions
If a charged particle goes unaccelerated in a region containing electric and magnetic fields,
View SolutionIf a charged particle goes unaccelerated in a region containing electric and magnetic fields,
View SolutionA charged particle goes undeflected in a region containing electric and magnetic field. It is possible that
View SolutionFind the condition under which the charged particles moving with different speeds in the presence of electric and magnetic field vectors can be used to select charged particles of a particular speed.
View SolutionA charged particle enters a uniform magnetic field perpendicular to it. The magnetic field
View Solution(a) Write the expression for the force, →F, acting on a charged particle of charge 'q', moving with a velocity →V in the presence of both electric field →E and magnetic field →B. Obtain the condition under which the particle moves undeflected through the fields.
(b) A rectangular loop of size l×b carrying a steady current I is placed in a uniform magnetic field →B. Prove that the torque →τ acting on the loop is given by
→τ=→m×→B, where →m is the magnetic moment of the loop.View SolutionIf the velocity of charged particle has both perpendicular and parallel components while moving through a magnetic field ,then what is the path following by a charged particle?
View SolutionA charged particle passes through a region that could have uniform electric field only or uniform magnetic field only or both electric and magnetic fields or none of the fields. Some possible paths of motion are mentioned in Coliumn-I with the related information in Column-II
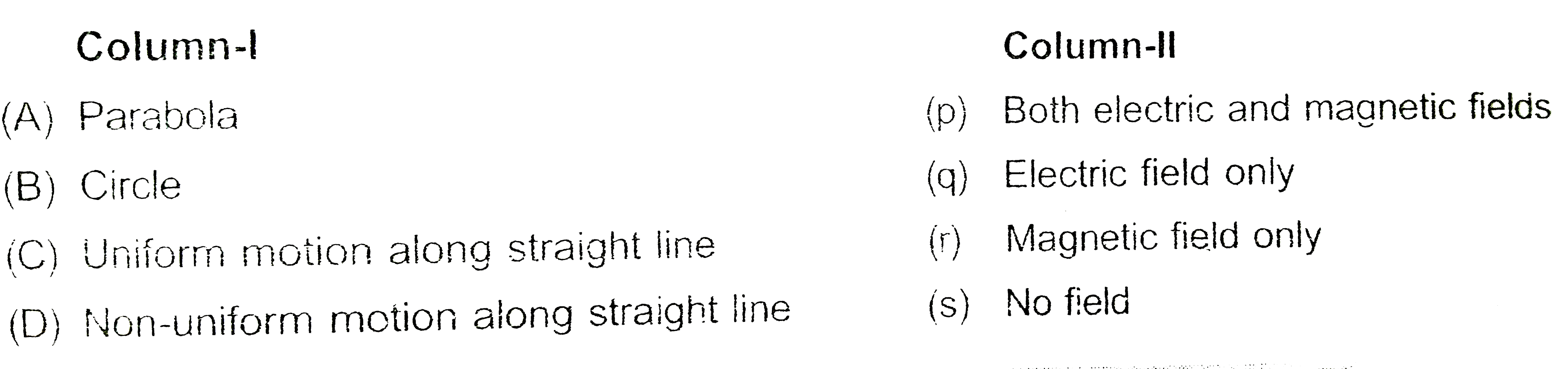 View Solution
View SolutionIf a charged particle enters perpendicular in the uniform magnetic field then
View SolutionA particle having charge q moves with a velocity v through a region in which both an electric field →E and a magnetic field B are present. The force on the particle is
View Solution
