Question

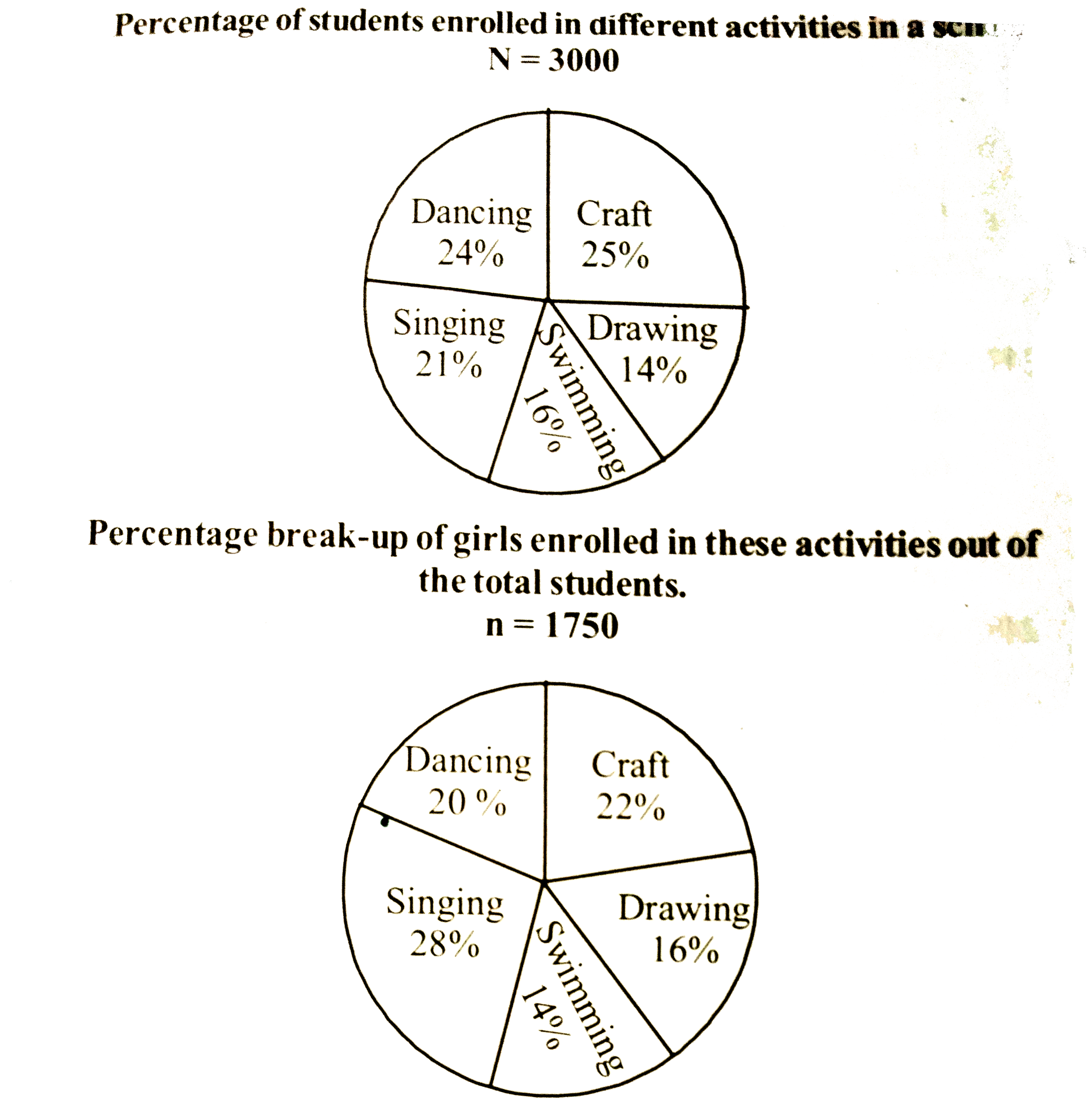
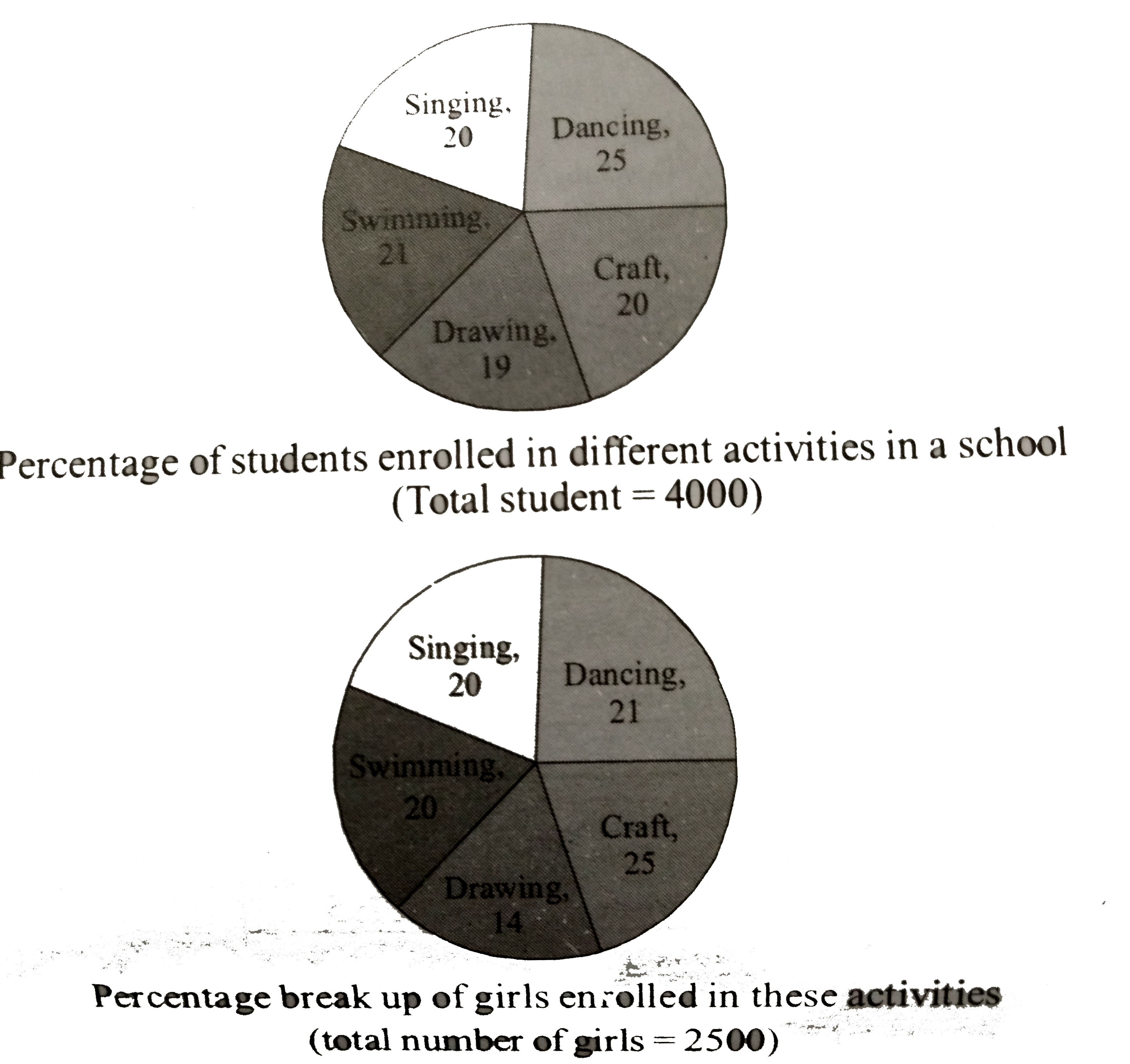
In a school, out of 900 students, $85\%$ of the girls and $70\%$ of the boys passed. How many boys appeared in the examination if the pass percentage of the school was $80\%$?

Options

A. 300
B. 450
C. 430
D. 200

Answer

A

Explanation

To solve the problem step by step, we can follow these instructions:

Step 1: Define Variables
Let:
- \( x \) = number of boys
- \( y \) = number of girls

From the problem, we know that the total number of students is 900. Therefore, we can write the equation:
\( x + y = 900 \)

Step 2: Express Passing Students
According to the problem:
- 85% of the girls passed, which can be expressed as \( 0.85y \).
- 70% of the boys passed, which can be expressed as \( 0.70x \).

Step 3: Total Passed Students
The total number of students who passed is given as 80% of the total students:
\( 0.80 \times 900 = 720 \)

Step 4: Set Up the Equation
Now, we can set up the equation based on the total number of students who passed:
\( 0.85y + 0.70x = 720 \)

Step 5: Substitute \( y \)
From the first equation \( x + y = 900 \), we can express \( y \) in terms of \( x \):
\( y = 900 - x \)

Step 6: Substitute \( y \) in the Passing Equation
Now, substitute \( y \) in the passing equation:
\( 0.85(900 - x) + 0.70x = 720 \)

Step 7: Simplify the Equation
Expanding the equation:
\( 765 - 0.85x + 0.70x = 720 \)
Combine like terms:
\( 765 - 0.15x = 720 \)

Step 8: Solve for \( x \)
Now, isolate \( x \):
\( -0.15x = 720 - 765 \)
\( -0.15x = -45 \)
Dividing both sides by -0.15:
\( x = \frac{-45}{-0.15} = 300 \)

Step 9: Conclusion
Thus, the number of boys who appeared in the examination is \( x = 300 \).