Directions: Given data shows total male and female doctors in three hospital in a seminar. Read data carefully and answer the question:
In annual seminar of three hospitals, A, B and C some male and female doctors represent their hospitals. Average number of female doctors who represent A and B is 210. Total male doctors in A and B is 810. Number of female doctors is 23rd and 25th of male doctor in A and B repectively. Total female doctor who represent C are 25% more than total female doctor who represent A and total male doctor who represent C are 3313% more than female doctor who represent B.
Find average number females in B and C?
250
210
260
240
The correct Answer is:D
To solve the problem step by step, let's break down the information given and calculate the required values.
Step 1: Define Variables
Let:
- MA = Number of male doctors in Hospital A
- MB = Number of male doctors in Hospital B
- FA = Number of female doctors in Hospital A
- FB = Number of female doctors in Hospital B
- FC = Number of female doctors in Hospital C
- MC = Number of male doctors in Hospital C
Step 2: Set Up Equations
From the problem, we have the following information:
1. The average number of female doctors in A and B is 210:
FA+FB2=210⟹FA+FB=420
2. The total number of male doctors in A and B is 810:
MA+MB=810
3. The number of female doctors in A is 23 of the male doctors in A:
FA=23MA
4. The number of female doctors in B is 25 of the male doctors in B:
FB=25MB
Step 3: Substitute and Solve
Substituting FA and FB into the equation FA+FB=420:
23MA+25MB=420
To eliminate the fractions, we can multiply through by 15 (the least common multiple of 3 and 5):
10MA+6MB=6300(Equation 1)
Now we have two equations:
1. MA+MB=810 (Equation 2)
2. 10MA+6MB=6300 (Equation 1)
Step 4: Solve the System of Equations
From Equation 2, we can express MB in terms of MA:
MB=810−MA
Substituting this into Equation 1:
10MA+6(810−MA)=6300
10MA+4860−6MA=6300
4MA=6300−4860
4MA=1440⟹MA=360
Now substituting MA back to find MB:
MB=810−360=450
Step 5: Find Female Doctors in A and B
Now we can find FA and FB:
FA=23MA=23×360=240
FB=25MB=25×450=180
Step 6: Calculate Female Doctors in C
According to the problem, the total female doctors who represent C are 25% more than total female doctors who represent A:
FC=FA+0.25FA=240+60=300
Step 7: Calculate Average Number of Females in B and C
Now we can find the average number of female doctors in B and C:
Average=FB+FC2=180+3002=4802=240
Final Answer
The average number of female doctors in B and C is 240.
---
Let:
- MA = Number of male doctors in Hospital A
- MB = Number of male doctors in Hospital B
- FA = Number of female doctors in Hospital A
- FB = Number of female doctors in Hospital B
- FC = Number of female doctors in Hospital C
- MC = Number of male doctors in Hospital C
From the problem, we have the following information:
1. The average number of female doctors in A and B is 210:
FA+FB2=210⟹FA+FB=420
2. The total number of male doctors in A and B is 810:
MA+MB=810
FA=23MA
FB=25MB
Substituting FA and FB into the equation FA+FB=420:
23MA+25MB=420
To eliminate the fractions, we can multiply through by 15 (the least common multiple of 3 and 5):
10MA+6MB=6300(Equation 1)
1. MA+MB=810 (Equation 2)
2. 10MA+6MB=6300 (Equation 1)
From Equation 2, we can express MB in terms of MA:
MB=810−MA
10MA+6(810−MA)=6300
10MA+4860−6MA=6300
4MA=6300−4860
4MA=1440⟹MA=360
MB=810−360=450
Now we can find FA and FB:
FA=23MA=23×360=240
FB=25MB=25×450=180
According to the problem, the total female doctors who represent C are 25% more than total female doctors who represent A:
FC=FA+0.25FA=240+60=300
Now we can find the average number of female doctors in B and C:
Average=FB+FC2=180+3002=4802=240
The average number of female doctors in B and C is 240.
Similar Questions
Number of females/ males in India
View SolutionDoctor: Hospital
View SolutionDoctor : Hospital : : Chef : ?
View SolutionRead the following information carefully and answer the questions given below.
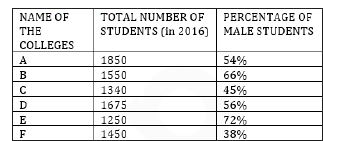
Find the average difference between the number of male and female students in all the colleges?
View SolutionThe following bar-diagram shows total number of males and females in five different organisations. Study it carefully to answer the questions.
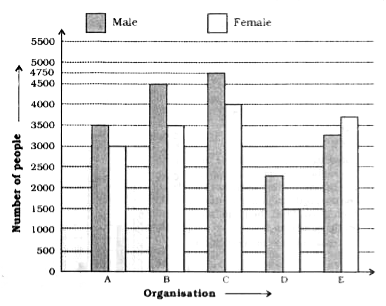
What is the difference between the total number of females and the total number of males from all the organisations together?
View SolutionDoctor : Hospital :: Teacher: _?_
View SolutionChoose the correct figure that represents the given relation:
Blue eyed, females, doctors
View SolutionThe following bar-diagram shows total number of males and females in five different organisations. Study it carefully to answer the questions.
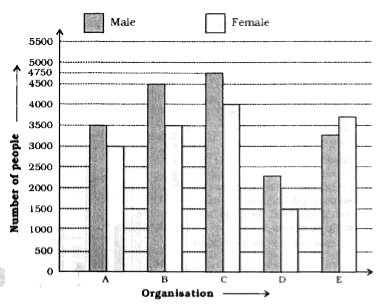
What is the ratio of average number of females from the organisations A, B and C to the average number of males from the organisations C, D and E?
View SolutionThe following bar-diagram shows total number of males and females in five different organisations. Study it carefully to answer the questions.
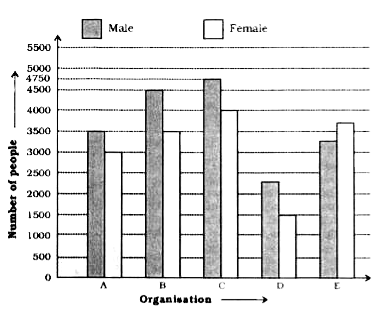
What is the ratio of the number of females from the organisations B and C to the number of males from the organisations D and E?
View SolutionThe following bar-diagram shows total number of males and females in five different organisations. Study it carefully to answer the questions.
Males from organisations A and B together form what per cent of total number of males from organisations C, D and E together?
View Solution
