the moment of inertia ofa uniform rod of mass 0.50 kg and length 1 m is 0.10 kg m^2 about a line perpendicular to the rod. Find the distance of this line from the middle point of the rod.Solution in Gujrati
The correct Answer is:C, D
Length of the rod=1m, Mass of the rod
=0.5kg
Let at distance d from the centre the rod is moving.
Applying parallel axis therom, the moment of inertia about that point
→(ml212)+md2=0.10
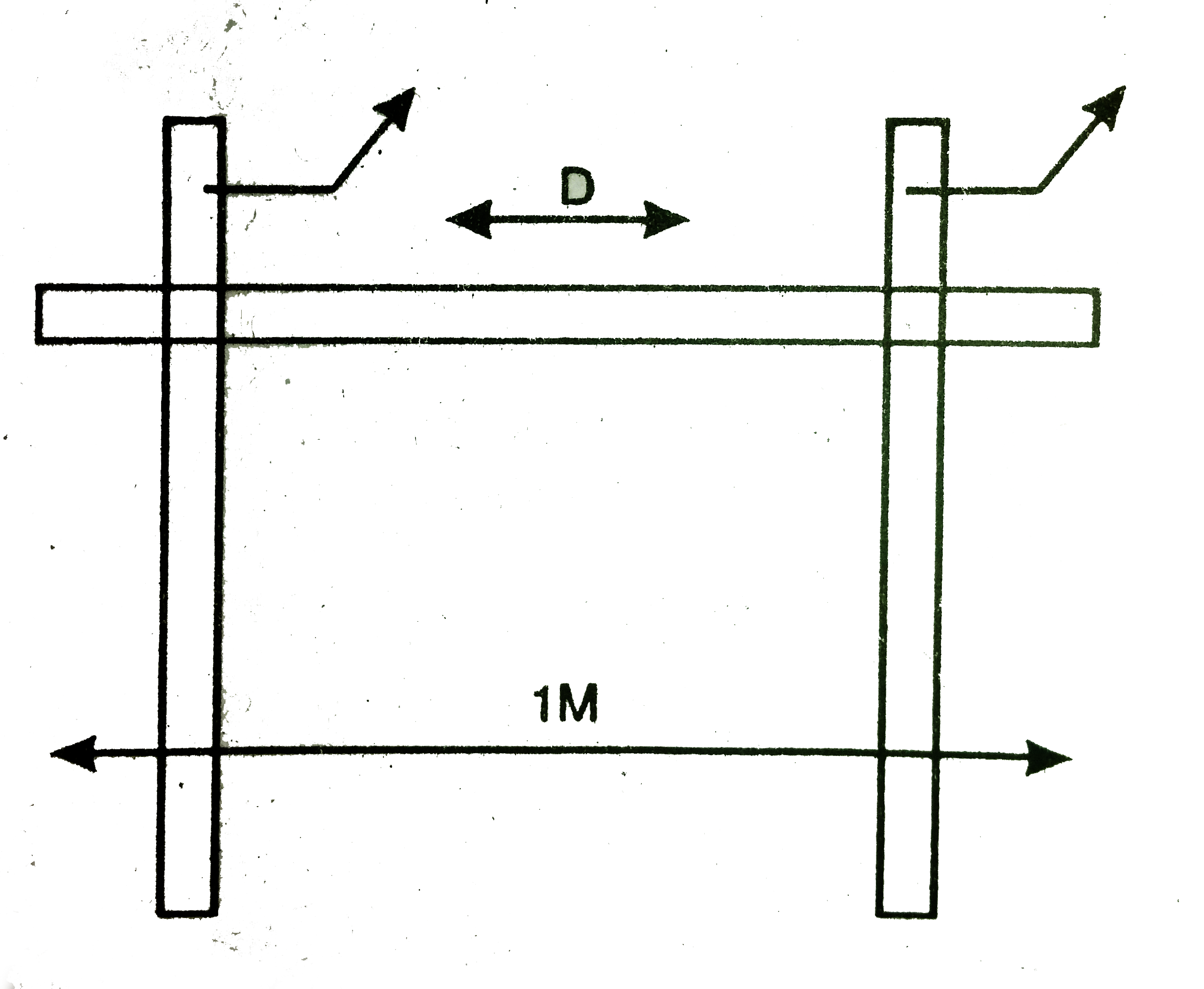
→0.5×l212+0.5×d2=0.10 ltbr> →112+d2=0.2
→d2=0.118
d=0.342m from the centre
Similar Questions
एक छड़ (लम्बाई l और द्रव्यमान m) का जड़त्व आघूर्ण उस अक्ष के सापेक्ष, जो छड़ की लम्बाई के लम्बवत् है तथा इस छड़ के मध्य बिन्दु और एक सिरे से बराबर दूरी पर स्थित बिन्दु से गुजरता है, है
03:17View SolutionA uniform thin rod of 120 cm length and 1600 g mass is bent as shown in the figure. The moment of inertia of the bent rod about and axis passing through the point 'O' and perpendicular to the plane of the paper is . . . . . . kg -m^(2) .
03:45View SolutionThe moment of inertia of a uniform rod of mass 0.50 kg and length 1 m is 0.10 kg m^2 about a line perpendicular to the rod. Find the distance of this line from the middle point of the rod.
04:01View SolutionFind the moment of inertia and radius of gyration of uniform cross-section rod of mass M and length l about an axis through a point at distance l3 from one end and perpendicular to the length of rod.
03:31View SolutionThe moment of inertia of a thin uniform rod of mass M and length l about an axis perpendicular to the rod through its centre is I. The moment of inertia of the rod through its end point is
View SolutionMoment of inertia of a uniform rod of mass m and length l is 712ml2 about a line perpendicular to the rod. Find the distance of this line from the middle point of the rod.
02:24View SolutionCalculate the moment of inertia of a uniform rod of mass M and length l about an axis passing through an end and perpendicular to the rod. The rod can be divided into a number of mass elements along the length of the rod.
01:28View SolutionTwo uniform solid of masses m_(1) and m_(2) and radii r_(1) and r_(2) respectively, are connected at the ends of a uniform rod of length l and mass m . Find the moment of inertia of the system about an axis perpendicular to the rod and passing through a point at a distance of a from the centre of mass of the rod as shown in figure.
02:26View Solution
