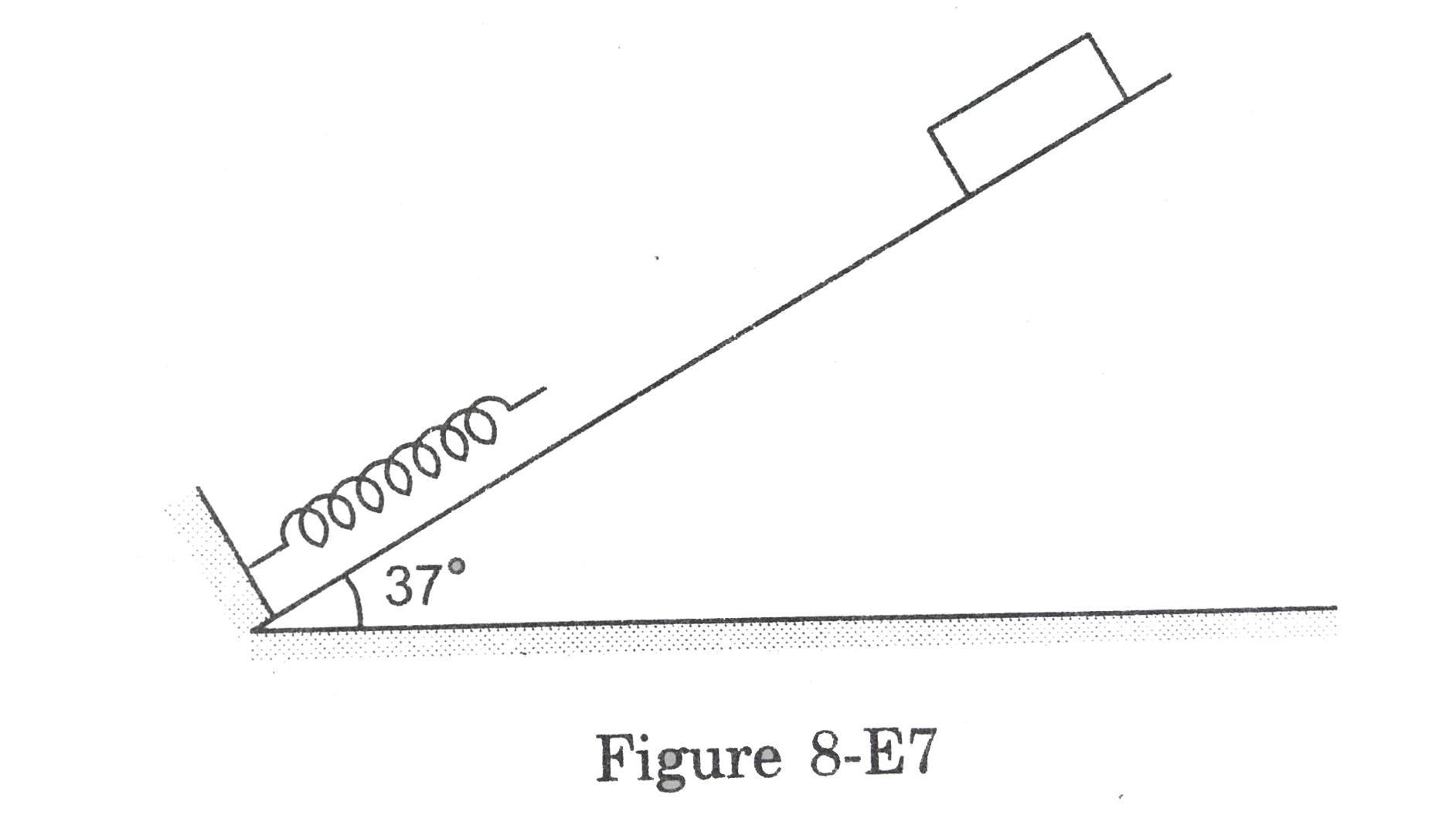
Figure shows a spring fixed at the bottom end of an incline of inclination 370. A small block of mass 2 kg starts slipping down the incline from a point 4.8 m away from the spring. The block compresses the spring by 20 cm, stops momentarily and then rebounds through a distance of 1 m up the incline. Find a. the frictioin coefficient between the plane and the block and b. the spring constant of the spring. Take g=10ms2.
 Solution in Gujrati
Solution in Gujrati
The correct Answer is:A, B
m=2kg,S1=4.8m,x=20cm=0.2m
S2=1m
sin370=0.60=35
θ=370
cos 37^0=0.80=4/5=10m/sec^2<br><imga≤ocr_imageload∈g=lazysrc=https://d10lpgp6xz60nq.cloudfront.net/physics_images/HCV_VOL1_C08_E01_080_S01.pngwh=80%sty≤=background:white;><br>Apply∈gworke≠rgypr∈cip≤fordownwardmotionofthebody<br>0-0=mgsin37^0(x+4.8)-muRx5-1/2kx62<br>20xx(0.80)xx5-1/2k(0.2)^2=0<br>rarr 60-80mu-0.02k=0<br>→80mu+0.02k=60<br>Similarlyfortheupwardmotionofthebodytheequationis<br>0-0=(-mgsin37^0)-muRx1+1/(2k)(-2)^2<br>rarr 20xx(0.06)xx1-muxx20xx(0.80)xx1-1/2k(0.2)^2<br>rarr 12-16mu+0.02k=0<br>Adding equation i and equation ii we get
96μ=48
→μ=0.5
Now putting the value of μ in equation i,
k=1000Nm
Similar Questions
In figure a spring with k = 168 N//m is in its relaxed length and is at the top of a frictionless incline of angle theta = 37^(@) . The lower end of the incline is at distance D = 1 m from the end of the spring. A small blcok of mass 2 kg is pushed against the spring until the spring is compressed by 0.2 m and released from rest. What is the speed of the block at the instant the spring returns to its relaxed length (which is when the block loses contact with the spring)?
View SolutionIn figure a spring with k = 168 N//m is in its relaxed length and is at the top of a frictionless incline of angle theta = 37^(@) . The lower end of the incline is at distance D = 1 m from the end of the spring. A small blcok of mass 2 kg is pushed against the spring until the spring is compressed by 0.2 m and released from rest. What is the speed of the block when it reaches the lower end of the incline?
View SolutionA block of mass m slips down an inclined plane as shown in the figure. When it reaches the bottom it presses the spring by a length (spring length ⟨h and spring constant = K)-
View SolutionA spring with constant k = 78 N/m is at the base of a frictionless, 30.0^(@) inclined plane. A 0.50 kg block is pressed against the spring, compressing it 0.20 m from its equilibrium position. The block is then released. If the block is not attached to the spring, how far up the incline will it travel before it stops ?
View Solutiona. A 2−kg situated on a smooth fixed incline is connected to a spring of negligible mass, with spring constant k=100Nm−1, via a frictionless pulley. The block is released from rest when the spring is unstretched. How far does the block moves down the incline before coming (momentarily) to rest? What is its acceleration at its lower point?
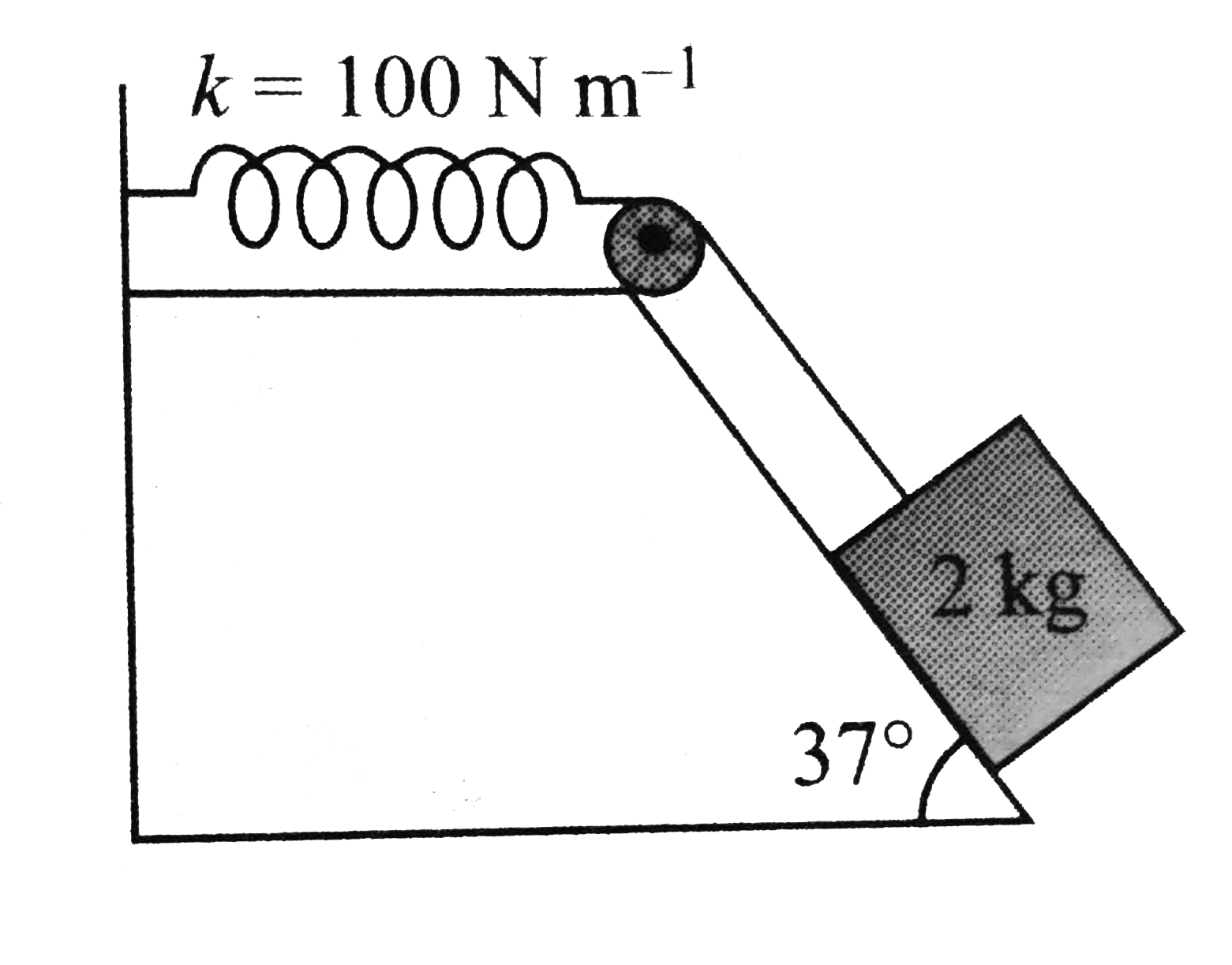
b. The experiment is repeated on a rough incline. If the block is observed to move 0.20m down along the incline before it comes to instantaneous rest, calculate the coefficient of kinetic friction.
View SolutionA block of mass 4 kg is released from the top of an inclined smooth surface as shown in figure If spring constant of spring is 100N/m and block comes to rest after compressing spring by 1 m, find the distance travelled by the block before it comes t o rest.

View SolutionShown in the figure a spring fixed at the bottomm end of all incline of inclination 37^@ . A small block of mass 2 kg starts slipping down the incline form a point 4.8 m aaway from the spring. The block compresses the spring by 20 cm, stops momentarily and then rebounds through a distance of 1 m up the incline. Find the friction coefficient between the palne
View SolutionShown in the figure a spring fixed at the bottomm end of all incline of inclination 37^@ . A small block of mass 2 kg starts slipping down the incline form a point 4.8 m aaway from the spring. The block compresses the spring by 20 cm, stops momentarily and then rebounds through a distance of 1 m up the incline. Find the spring constant of the spring Take g = 10 ms^-2
View Solution
