Question

Study the following information carefully and answer the questions given below:
Six cars i.e. M, S, R, T, Q and P which are parked in a parking area in a straight path such that the distance between a car and its adjacent cars is same for all the cars at the instant when they all are parked in a straight line. (i.e. Distance between car Mand car Pis equal to the distance between car Pand car Sif Mand Sare adjacent to P). All cars front side are facing in the north direction. Car Pis parked third to the left of car Q. Car R is parked third to the right of car M. Car P is placed between car S and car R. Car P takes a U turn and started moving and covers distance of 8 unit to reach point A. From point A Car P takes ${135}^{\circ }$ right turn and then again covers 10 unit to reach at the point where car Mis parked. Car Pagain covers 4 unit in the north direction from the point Where car M is parked to reach point K. CarQ starts moving in the north direction and covers a certain distance to reach point L.
What is the shortest distance between point K and car Q final position (if the point K and point L lies in a straight line)?

Options

A. 14 unit
B. 15 unit
C. 17 unit
D. 13 unit

Answer

B

Explanation

Step 1:- From the given statements, the distance between a car and its adjacent cars is same for all the cars at the instant when they all are parked in a straight line. All cars front side are facing in the north direction. Car R is parked third to the right of car M. Car P is placed between car S and car R so there will be two possible cases

Step II :- Now from the given condition Car Pis parked third to the left of car Q case 1 will be eliminated and we get the final positions of cars in the parking area.

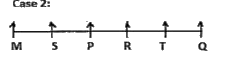
Step III :- Now we will determine the distance between the adjacent cars,
Car P moved 8 unit towards south direction (as it takes U turn from its initial position) to reach point A From point A Car P takes 135o right turn and then again covers 10 unit to reach at the point where car M is parked. Car P again covers 4 unit in the north direction from the last position to reach point K. Car Q starts moving in the north direction and covers a certain distance to reach point L.
Shortest distance between point A and car M initial) is 10 unit.
By using Pythagoras theorem,
The distance between Car M and initial position of car `P =sqrt((100-64))=sqrt((36))=6` unit
So 2x = 6 unit
(Where x, is distance between two adjacent cars)
x= 3 unit,
So, we have

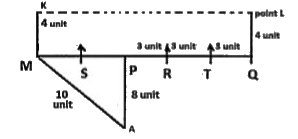
As it is given that Point K and point L lies in a straight line then the distance between both the point is equal to the distance between Car M and Car Q.s initial positions.