A boat moves relative to water with a velocity with a velocity v is n times less than the river flow u. At what angle to the stream direction must the boat move to minimize drifting ?

Video Solution

Text Solution

Verified by Experts

In this problem, one thing should be carefully noted that the velocity of boat is less than the river flow velocity. In such a case, boat cannot reach the point directly opposite to its starting point , i.e,. Drift can never be zero. Thus, to minimize the drift , boat starts at an angle $θ$ from the normal direction up stream as shown.

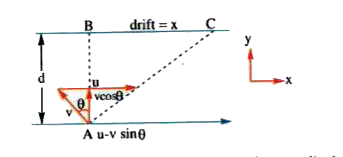
now, again if we find the components of velocity of boat along and perpendicular to the flow , these are, velocity along the river, $V_{x} = u - v sin θ$
and velocity perpendicular to the river, $V_{y} = v cos θ$ .
time taken to cross the river is $t = \frac{d}{v_{y}} = \frac{d}{v cos θ}$
In this time, drift $x = (v_{x}) t = (u - v sin θ) \frac{d}{v cos θ} (or) x = \frac{u d}{v} sec θ - d tan θ$ .
The drift x is minimum , when $\frac{d x}{d t h η} = 0 (or) (\frac{u d}{v}) (sec θ . tan θ) - d {sec}^{2} θ = 0$
(or) $\frac{u}{v} sin θ = 1 or sin θ = \frac{v}{u} = \frac{1}{n} (a s v = \frac{u}{n})$
So, for minimum drift , the boat must move at an angle $θ = {sin}^{- 1} (\frac{v}{u})$ from normal direction or an angle $\frac{π}{2} + {sin}^{- 1} (\frac{v}{u})$ from stream direction.

Updated on:21/07/2023

|

Class 11 PHYSICS VECTOR

Similar Questions

Recommended Questions

Home
Ask Doubt
Profile