A vessel whose bottom has round holes with diameter , is filled with water. The maximum height up to which water can be filled without leakage is
20 cm
40 cm
30 cm
60 cm
The correct Answer is:C
Step by step video solution for A vessel whose bottom has round holes with diameter 0.1 mm, is filled with water. The maximum height up to which water can be filled without leakage is by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
A vessel, whose bottom has round holes with diameter of 0.1 mm , is filled with water. The maximum height to which the water can be filled without leakage is (S.T. of water =75 dyne/cm , g=1000 cm/s)
View SolutionA vessel whose bottom has round holes with a diameter of d=0.1mm is filled with water. The maximum height of the water level h at which the water does not flow out, will be (The water does not wet the bottom of the vessel). [STofwater=70dyne/cm]
View SolutionA vessel whose bottom has round holes with diameter of 1 mm is filled with water Assuming that surface tension acts only at holes, then the maximum height to which the water can be filled in vessel without leakage is (given surface tension of water is 75×10−3N/m) and g=10m/s2
View SolutionA cylindrical tank has a hole of 1cm2 in its bottom. If the water is allowed to flow into the tank from a tube above it at the rate of 70cm3/sec, then the maximum height up to which water can rise in the tank is
View SolutionA cylindrical tank has a hole of 1cm2 in its bottom. If the water is allowed to flow into the tank from a tube above it at the rate of 70cm3/sec, then the maximum height up to which water can rise in the tank is
View SolutionA container of height 10 cm is filled with water. There is hole at bottom. Find the pressure difference between point A & B .
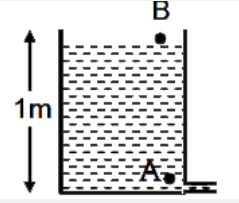
View SolutionA wide vessel with a small hole in the bottom is filled with water and kerosene. Find the velocity of water flow if the thickness of water layer is h1=30cm and that of kerosene is h2=20cm brgt

View SolutionA cylinderical tank of height 0.4 m is open at the top and has a diameter 0.16m. Water is filled in it up to height of 0.16 m. Find the time taken to empty the tank through a hole of radius 5×10−3m in its bottom.
View SolutionA cylindrical tank has a hole of diameter 2r in its bottom. The hole is covered wooden cylindrical block of diameter 4r, height h and density ρ/3.

Situation I: Initially, the tank is filled with water of density ρ to a height such that the height of water above the top of the block is h1 (measured from the top of the block).
Situation II: The water is removed from the tank to a height h2 (measured from the bottom of the block), as shown in the figure. The height h2 is smaller than h (height of the block) and thus the block is exposed to the atmosphere.
Find the minimum value of height h1 (in situation 1), for which the block just starts to move up?
View SolutionA cylindrical tank has a hole of diameter 2r in its bottom. The hole is covered wooden cylindrical block of diameter 4r, height h and density ρ/3.

Situation I: Initially, the tank is filled with water of density ρ to a height such that the height of water above the top of the block is h1 (measured from the top of the block).
Situation II: The water is removed from the tank to a height h2 (measured from the bottom of the block), as shown in the figure. The height h2 is smaller than h (height of the block) and thus the block is exposed to the atmosphere.
Find the minimum value of height h1 (in situation 1), for which the block just starts to move up?
View Solution
