Find the PE of three objects of masses 1 kg, 2 kg and 3 kg placed at the three vertices of an equilateral triangle of side 20 cm.
50G J
−55GJ
5G J
60 G J
The correct Answer is:B
To find the potential energy (PE) of three objects of masses 1 kg, 2 kg, and 3 kg placed at the vertices of an equilateral triangle with a side length of 20 cm, we can follow these steps:
1. Identify the Masses and Distance:
- Let the masses be m1=1kg, m2=2kg, and m3=3kg.
- The side length of the equilateral triangle is s=20cm=0.2m.
2. Formula for Gravitational Potential Energy:
- The gravitational potential energy U between two masses m1 and m2 separated by a distance r is given by:
U=−Gm1m2r
- Here, G is the gravitational constant, approximately 6.674×10−11N m2/kg2.
3. Calculate the Potential Energy for Each Pair:
- The potential energy between the pairs of masses at the vertices of the triangle:
- Between m1 and m2:
U12=−Gm1m2s=−G⋅1⋅20.2
- Between m2 and m3:
U23=−Gm2m3s=−G⋅2⋅30.2
- Between m3 and m1:
U31=−Gm3m1s=−G⋅3⋅10.2
4. Sum the Potential Energies:
- The total potential energy Utotal of the system is the sum of the potential energies calculated above:
Utotal=U12+U23+U31
- Substituting the values:
Utotal=−G⋅1⋅20.2−G⋅2⋅30.2−G⋅3⋅10.2
=−G0.2(2+6+3)=−G0.2⋅11
5. Final Expression:
- Thus, the total potential energy becomes:
Utotal=−11G0.2=−55GJoules
Final Answer:
The potential energy of the system is −55GJoules.
- Let the masses be m1=1kg, m2=2kg, and m3=3kg.
- The side length of the equilateral triangle is s=20cm=0.2m.
- The gravitational potential energy U between two masses m1 and m2 separated by a distance r is given by:
U=−Gm1m2r
- Here, G is the gravitational constant, approximately 6.674×10−11N m2/kg2.
- The potential energy between the pairs of masses at the vertices of the triangle:
- Between m1 and m2:
U12=−Gm1m2s=−G⋅1⋅20.2
- Between m2 and m3:
U23=−Gm2m3s=−G⋅2⋅30.2
- Between m3 and m1:
U31=−Gm3m1s=−G⋅3⋅10.2
- The total potential energy Utotal of the system is the sum of the potential energies calculated above:
Utotal=U12+U23+U31
- Substituting the values:
Utotal=−G⋅1⋅20.2−G⋅2⋅30.2−G⋅3⋅10.2
=−G0.2(2+6+3)=−G0.2⋅11
- Thus, the total potential energy becomes:
Utotal=−11G0.2=−55GJoules
The potential energy of the system is −55GJoules.
Similar Questions
Three masses of 2 kg, 3 kg and 4 kg are located at the three vertices of an equilateral triangle of side 1 m. What is the M.I. of the system about an axis along the altitude of the triangle and passing through the 3 kg mass?
View SolutionLocate the centre of mass of a system of three particles 1.0 kg, 2.0 kg and 3.0 kg placed at the corners of an equilateral traingular of side 1m.
View Solution
Knowledge Check
Three particles of masses 1kg,32kg,and2kg are located the vertices of an equilateral triangle of side a. The x,y coordinates of the centre of mass are.
Aa. 5a9,2a3√3Bb. 2a3√3,5a9Cc. 5a9,2a√3Dd. 2a√3,5a9
Three point masses m1=2Kg,m2=4kgandm3=6kg are kept at the three corners of an equilateral triangle of side 1 m. Find the locationo of their center of mass.
View SolutionThree masses of 1kg, 2kg, and 3kg, are placed at the vertices of an equilateral triangle of side 1m. Find the gravitational potential energy of this system.
Take G=6.67×10−11N−m2/kg2.
View SolutionThree balls of masses 1kg,2kgand3kg are arranged at the corners of an equilateral triangle of side 1m. What will be the moment of inertia of the system and perpendicular to the plane of the triangle.
View SolutionThree charges 2 μC , -4 μC and 8 μC are places at the three vertices of an equilateral triangle of side 10 cm. The potential at the centre of triangle is
View SolutionThree point particles of masses 1.0 kg 2 kg and 3 kg are placed at three comers of a right angle triangle of sides 4.0 cm , 3.0 cm and 5.0 cm as shown in the figure . The centre of mass of the system is at point :
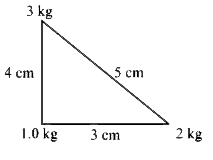
View SolutionThree point particles of masses 1.0 kg 2 kg and 3 kg are placed at three comers of a right angle triangle of sides 4.0 cm , 3.0 cm and 5.0 cm as shown in the figure . The centre of mass of the system is at point :

View SolutionThree particles of masses 1.0 kg 2.0 kg and 3.0 kg are placed at the corners A,B and C respectively of an equilateral triangle ABC of edge 1m. Locate the centre of mass of the system.
View Solution
