Four identical balls A,B,C and D are placed in a line on frictionless horizontal surface. A and D are moed with the same speed u towards the middle as shown. Assuming elastic collisions, find the final velocities.

The correct Answer is:VA=u(→),VB=0,VC=0andVD=u(→)
Step by step video solution for Four identical balls A,B, C and D are placed in a line on frictionless horizontal surface. A and D are moed with the same speed u towards the middle as shown. Assuming elastic collisions, find the final velocities. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Three balls A,B and C of same mass m are placed on a frictionless horizontal plane in a straight line as shown. Ball A is moved with velocity u towards the middle ball B. If all the collisions are elastic, find the final velocities of all the balls.

View SolutionIn the figure shown, the two identical balls of mass M and radius R each, are placed in contact with each other on the frictionless horizontal surface. The third ball of mass M and radius R/2, is coming down vertically and has a velocity =v0 when it simultaneously hits the two balls and itself comes to rest. Then, each of the two bigger balls will move after collision with a speed equal to

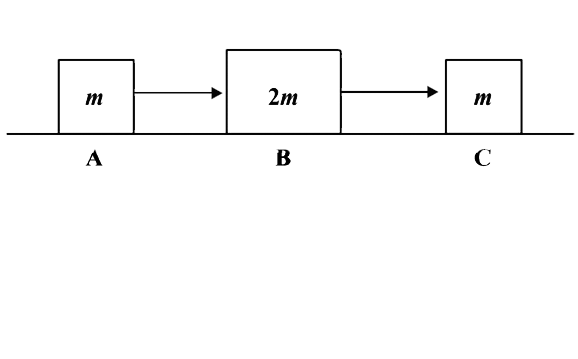
View SolutionThere object A,B and C are kept is a straing line a frictionless horizontal surface . These have masses have increase on 2m and m respectively . The object A move toward B with a speed 9 m//s and makes as elastic collision with a there after B makes completely inclesis with C . All motion over on the same straight line . Find the first speed of the object C
View SolutionTwo identical blocks A and B connected by massless string, are placed on a frictionless horizontal plane. A bullet havig the same mass, moving with speed u strickes block B from behind as shown. If the bullet gets embedded into block B then find

a. the velocity of A,B,C after collision.
b. impulse on A due to tension in the string,
c. impulse on C due to normal force of collision,
d. impulse on B due to normal force of collision.
View SolutionThree balls A,B and C each of mass m and same size,are placed along same line on smooth horizontal surface.A is given a velocity u towards B as shown.If coefficient of restitution for collision between A and B is 12 and between B and C is 13.Assuming all the collisions to be head on choose the correct options: (A) Final velocity of A is u4, (B) Final velocity of B is u4 (C) Final velocity of C is u2, (D) Total energy loss is 516mu2
View SolutionThree identical block A, B and C are placed on a horizontal frictionless surface. The blocks B and C are at rest but A is approaching towards B with a speed 10ms−1. The coefficient of restitution for all collisions is 0.5. The speed of the block C just after the collision is

View SolutionThree identical blocks A, B and C are placed on horizontal frictionless surface. The blocks B and C are at rest. But A is approaching towards B with a speed of 10ms−1
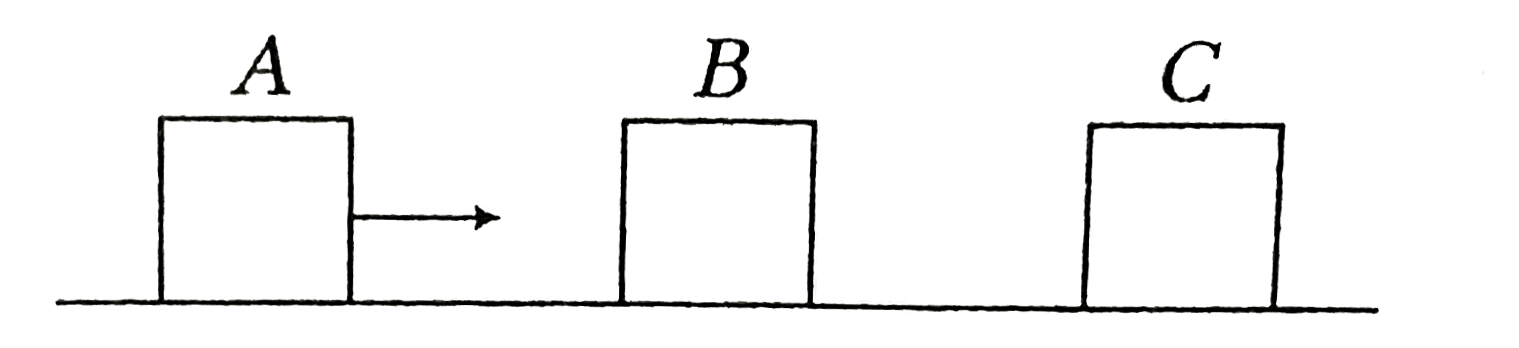
The coefficient of restitution for all collision is 0.5. The speed of the block C just after collision is
View Solution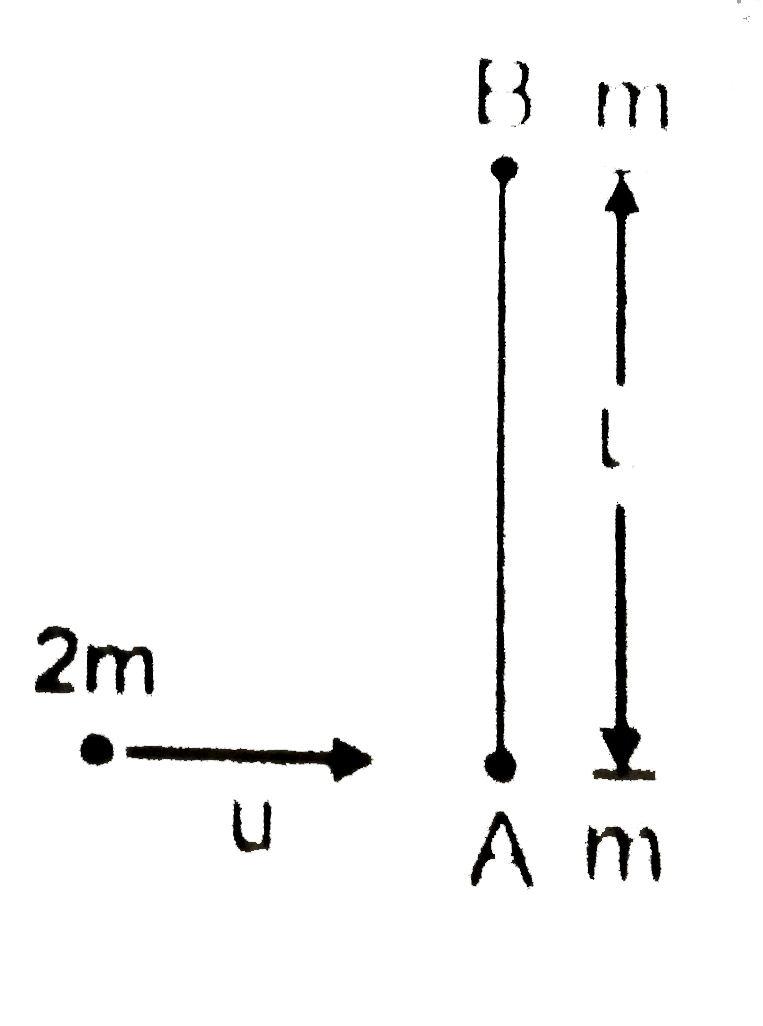
Two small balls A and B, each of mass m, are joined rigidly at the ends of a light rof of length L. they are placed on a frictionless horizontal surface another ball of mass 2 m moving with speed u towards one of the ball and perpendicular to the length of the rod on the horizontal frictionless surface as shown int he figure. if the coefficient of restitution is 1/2 then the angular speed of the rod after the collision will be
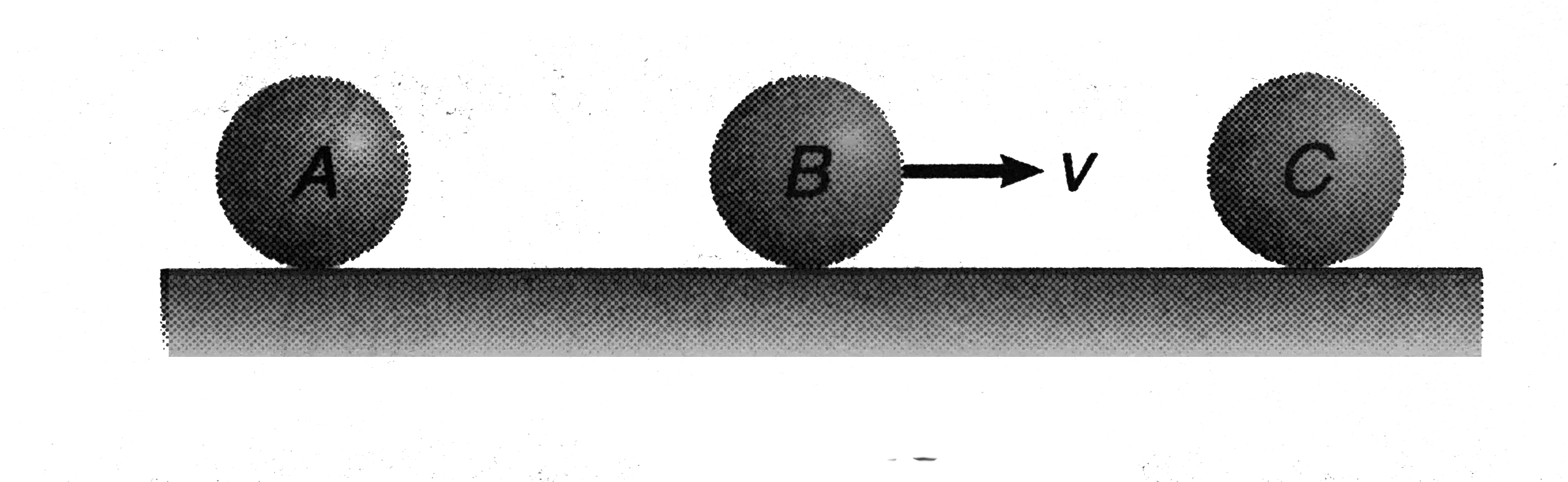
View SolutionThree balls A, B and C are placed on a smooth horizontal surface. Given that mA=mC=4mB. Ball B collides with ball C with an initial velocity v as shown in figure. Find the total number of collisions between the balls. All collisions are elastic.
View SolutionTwo identical balls, each of mass m, are tied with a string and kept on a frictionless surface. Initially, the string is slack. They are given velocities 2u and u. in the same direction. Collision between the balls is perfectly elastic.

What is the final loss in kinetic energy of the balls?
View Solution
