Current i flows through a long wire. A square loop made of conducting wire held below the wire in the same vertical plane is released and allowed to fall under gravity. If acceleration of the loop is a, then :
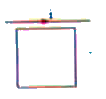
a current is induced in anticlockwise direction and a gt g
a current is induced in the clockwise direction and a lt g
a current is induced in anticlockwise direction and a lt g
no current is induced in the loop and a = g
The correct Answer is:B
Step by step video & image solution for Current i flows through a long wire. A square loop made of conducting wire held below the wire in the same vertical plane is released and allowed to fall under gravity. If acceleration of the loop is a, then : by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE - I (ASSERTION AND REASON)
14 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE - II (MAGNETIC FLUX, FARADAY.S LAW, LENZ.S LAW , AC GENERATOR)
10 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE - I (MAGNETIC FLUX, FARADAY.S LAW, LENZ.S LAW, AC GENERATOR)
26 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - AAKASH SERIESCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - PROBLEMS (LEVEL-II)
26 Videos
- ELECTROMAGNETIC WAVESBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - EXERCISE -II
22 Videos
Similar Questions
Two fixed long straight wires carry the same current i in opposite directions as shown in . A square loop of side b is fixed in the plane of the wires with its length parallel to one wire at a distance a shown in the figure.
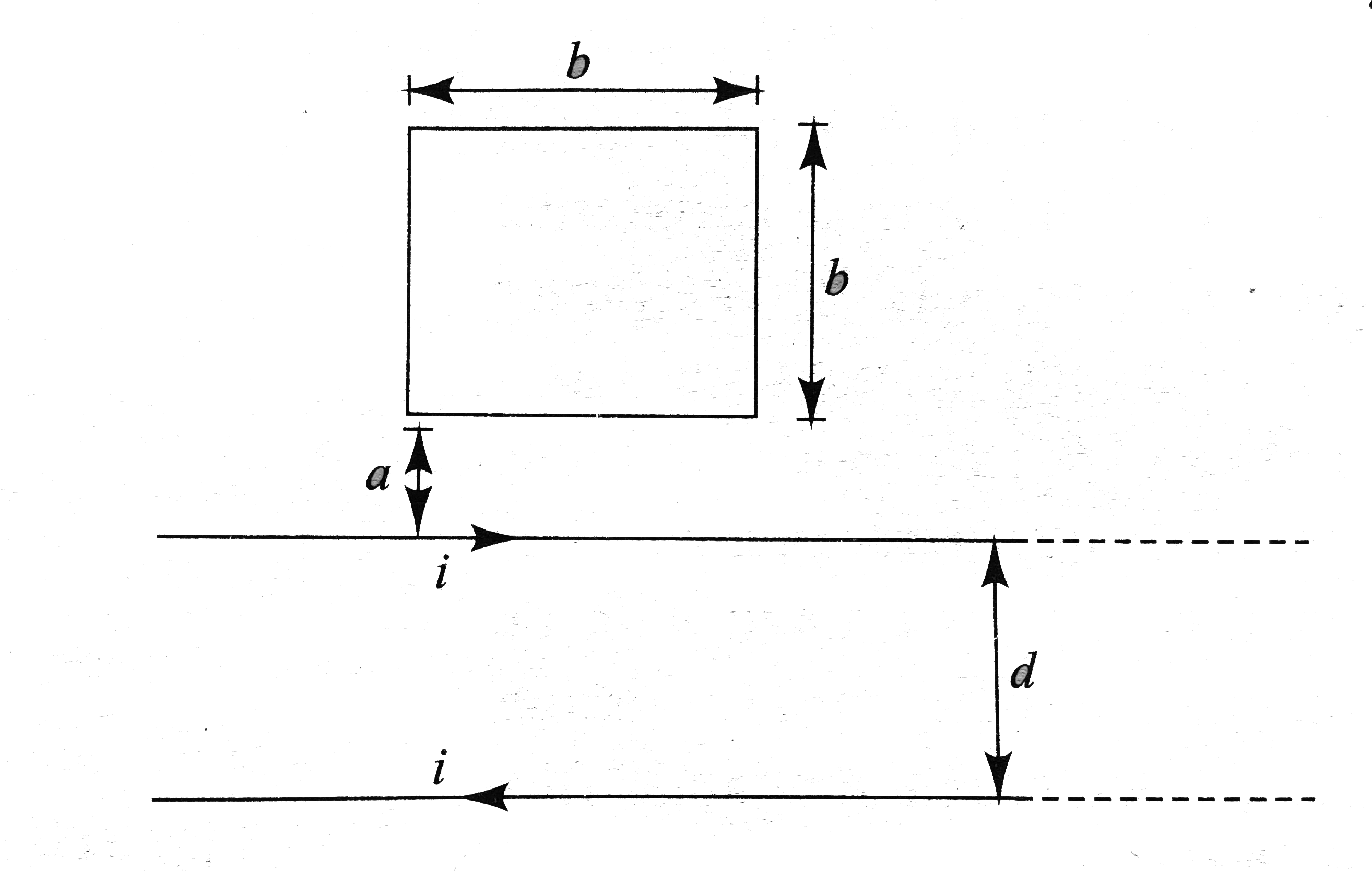
(a) alculate at the induced emf in the loop if the current in both the wires is changing at the rate di/dt.
(b) What is the direction of force on the loop if di/dt is positive?
View SolutionA constant current I flows through a long straight wire as shown in figure. A square loop starts moving towards righ with as constant speed v.

a Find induced emf produced i the loop as a function x
b. If total resistance of the loop is R, then find induced current in the loop,
View SolutionA circular loop and a square loop are formed from the same wire and the same current is passed through them . Find the ratio of their dipole moments.
View SolutionA long wire carrying a steady current is bent into a circular loop of one turn. The magnetic field at the centre of the loop is B. it is then bent into a circular coil of n turns. The magnetic field at the centre of this coil of n turns will be
View SolutionThe straight infinite long wire carries a constant current i to the right. The conducting circular loop in the same plane is being pulled upward by the external force. What is the direction of the magnetic force on the loop ?

View SolutionTwo different wire loops are concentric and lie in the same plane. The current in the outer loop is clockwise and increasing with time. The induced current in the inner loop then is
View SolutionA square metallic loop of side l is placed near a fixed long wire carrying a current i(figure).The loop is moved towards right perpendicular to the wire with a speed v in the plane containing the wire and the loop.The emf induced in the loop when the rear end of the loop is at a distance a=2l from the wire is μ0ivxπ.Find out value of x.

View SolutionA rectangular loop of wire with dimensions shown in figure is coplanar with a long wire carrying current 'I'. The distance between the wire and the left side of the loop is r. The loop is pulled to the right as indicated. What are the directions of the induced current in the loop and the magnetic forces on the left and right sides of the loop when the loop is pulled ?
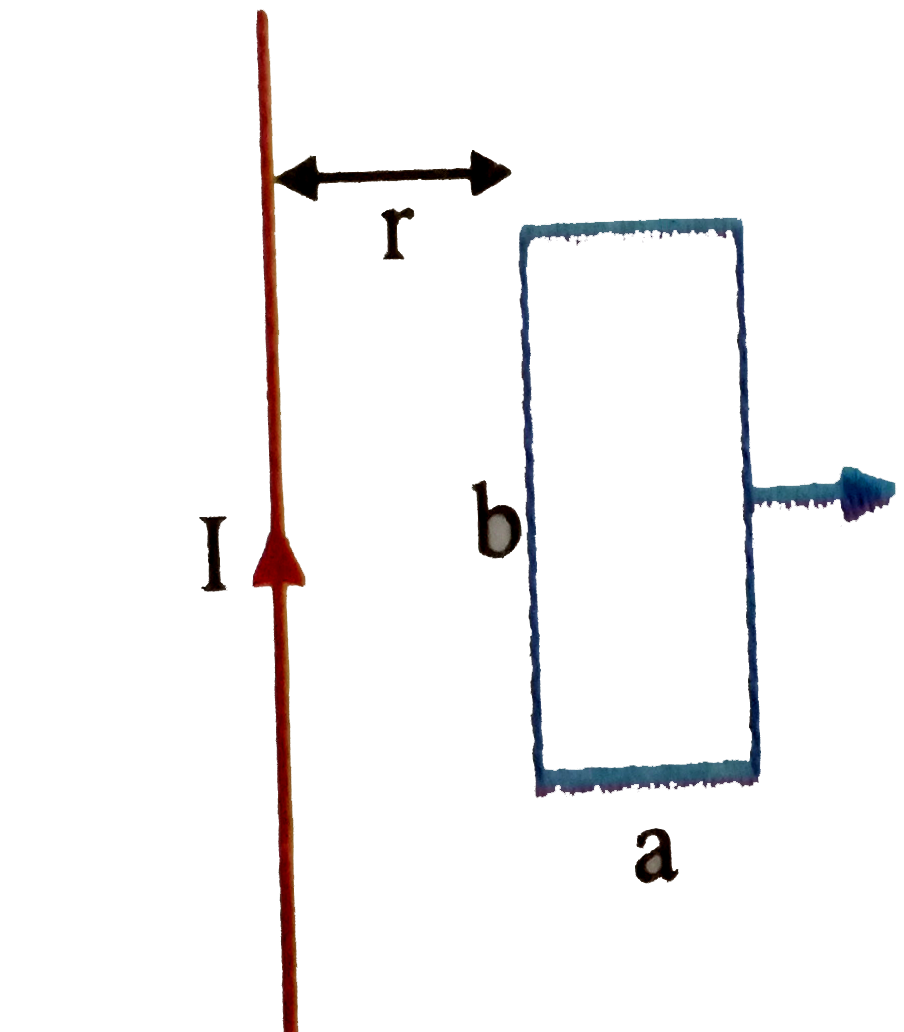
View SolutionA square loop is placed near a long current carrying straight wire as shown in the figure . Match the following table


View SolutionA long wire having a semi-circular loop of radius r carries a current I, as shown in Fig. Find the magnetic field due to entire wire.

View Solution
