A block of mass M slides along the sides of bowl as shown in the figure. The walls of the bowl are frictionless and the base has coefficient of friction 0.1, and length 0.5m. The block is released from the point A which is 0.2 m high as shown in figure. Then the block comes to rest
To solve the problem step by step, we will use the principles of conservation of energy and the work done against friction.
Step 1: Determine the initial potential energy
The block is released from a height of 0.2 m. The potential energy (PE) at this height can be calculated using the formula:
PE=mgh
where:
- m = mass of the block (we can keep it as m since it will cancel out later),
- g = acceleration due to gravity (approximately 9.81m/s2),
- h = height = 0.2 m.
So,
PE=mg⋅0.2
Step 2: Calculate the kinetic energy at the bottom
As the block slides down, the potential energy converts into kinetic energy (KE) at the bottom of the bowl. The kinetic energy can be expressed as:
KE=12mv2
At the bottom, all potential energy converts to kinetic energy:
mg⋅0.2=12mv2
Step 3: Solve for velocity
We can cancel m from both sides (assuming m≠0):
g⋅0.2=12v2
Rearranging gives:
v2=2g⋅0.2
v2=0.4g
Step 4: Calculate the work done against friction
When the block moves along the base of the bowl, it encounters friction. The work done against friction (W_f) can be calculated using:
Wf=friction force×distance
The friction force is given by:
f=μmg
where μ=0.1 (the coefficient of friction) and the distance is 0.5m.
So,
Wf=μmg⋅d=0.1mg⋅0.5
Wf=0.05mg
Step 5: Set up the energy conservation equation
The initial potential energy must equal the kinetic energy at the bottom minus the work done against friction:
mg⋅0.2=12mv2+Wf
Substituting Wf:
mg⋅0.2=12mv2+0.05mg
Step 6: Substitute v2 into the equation
Now substitute v2=0.4g into the equation:
mg⋅0.2=12m(0.4g)+0.05mg
Step 7: Simplify the equation
Cancelling m from both sides:
g⋅0.2=12(0.4g)+0.05g
g⋅0.2=0.2g+0.05g
g⋅0.2=0.25g
Step 8: Solve for height
Now, we can find the height to which the block rises after sliding on the base. The remaining energy after overcoming friction will be converted back into potential energy:
Remaining energy=mg⋅0.2−0.05mg=0.15mg
Setting this equal to the potential energy at height h:
0.15mg=mgh
Cancelling m gives:
0.15g=gh
Thus,
h=0.15m
Conclusion
The block comes to rest after rising to a height of 0.15 m.
The block is released from a height of 0.2 m. The potential energy (PE) at this height can be calculated using the formula:
PE=mgh
where:
- m = mass of the block (we can keep it as m since it will cancel out later),
- g = acceleration due to gravity (approximately 9.81m/s2),
- h = height = 0.2 m.
PE=mg⋅0.2
As the block slides down, the potential energy converts into kinetic energy (KE) at the bottom of the bowl. The kinetic energy can be expressed as:
KE=12mv2
mg⋅0.2=12mv2
We can cancel m from both sides (assuming m≠0):
g⋅0.2=12v2
v2=2g⋅0.2
v2=0.4g
When the block moves along the base of the bowl, it encounters friction. The work done against friction (W_f) can be calculated using:
Wf=friction force×distance
The friction force is given by:
f=μmg
where μ=0.1 (the coefficient of friction) and the distance is 0.5m.
Wf=μmg⋅d=0.1mg⋅0.5
Wf=0.05mg
The initial potential energy must equal the kinetic energy at the bottom minus the work done against friction:
mg⋅0.2=12mv2+Wf
mg⋅0.2=12mv2+0.05mg
Now substitute v2=0.4g into the equation:
mg⋅0.2=12m(0.4g)+0.05mg
Cancelling m from both sides:
g⋅0.2=12(0.4g)+0.05g
g⋅0.2=0.2g+0.05g
g⋅0.2=0.25g
Now, we can find the height to which the block rises after sliding on the base. The remaining energy after overcoming friction will be converted back into potential energy:
Remaining energy=mg⋅0.2−0.05mg=0.15mg
0.15mg=mgh
Cancelling m gives:
0.15g=gh
Thus,
h=0.15m
The block comes to rest after rising to a height of 0.15 m.
Similar Questions
Describe the motion of the mass m shown in figure. The walls and the block are elastic.
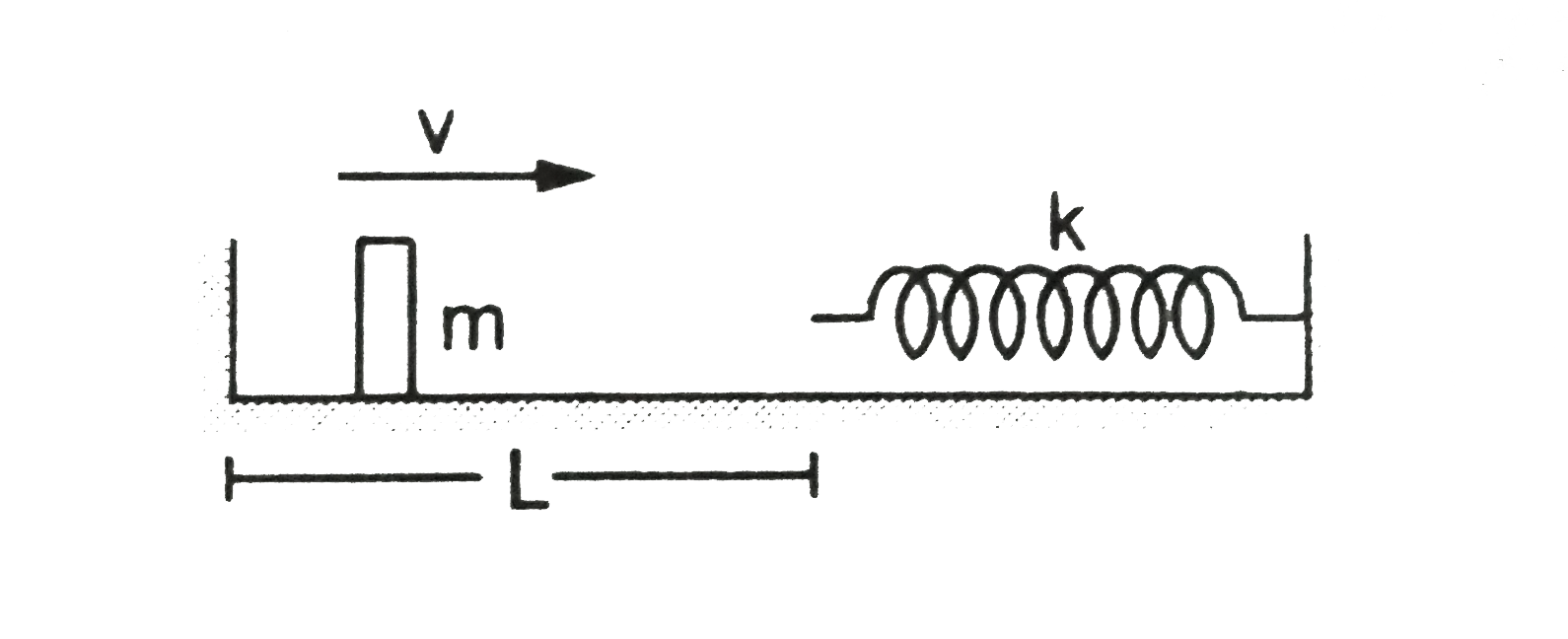
View SolutionA block of mass m slides along a frictionless surface as shown in the figure. If it is releases from rest from A what is its speed at B?
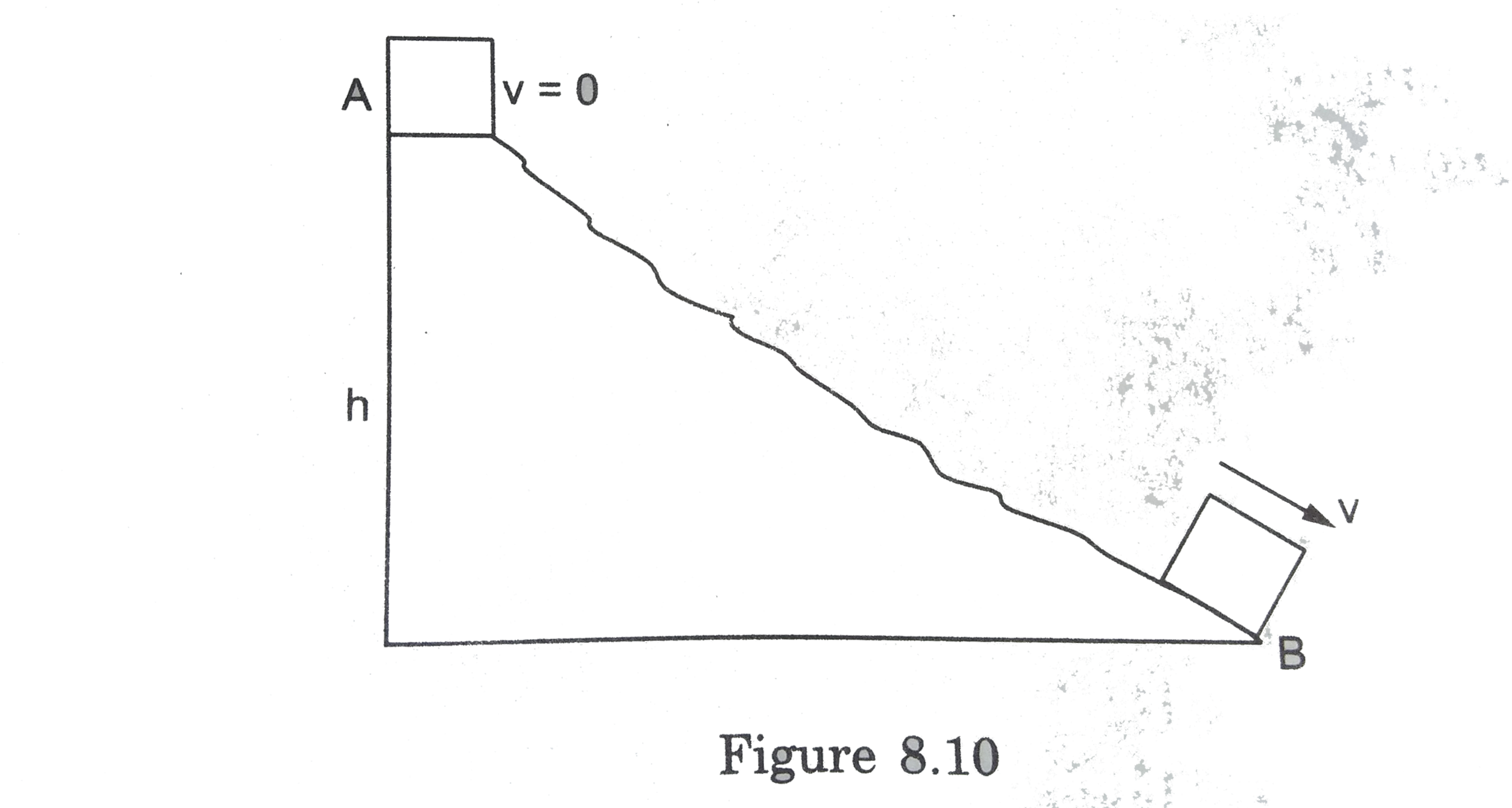
View SolutionA block of mass 'm' is released on the top of a frictionless incline as shown in the figure. The time period of the oscillation of the block is

View Solution
Two blocks of masses m1 and m2 are connected as shown in the figure. The acceleration of the block m2 is :
View SolutionTwo block of masses m1 and m2 are connected as shown in the figure. The acceleration of the block m2 is:

View SolutionA block of mass m is at rest on a rought wedge as shown in figure. What is the force exerted by the wedge on the block?

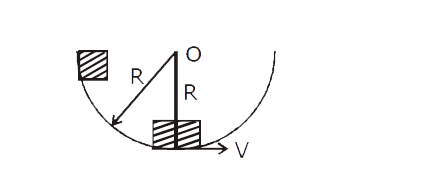
View SolutionA block of mass m slides down along the surface of the bowl from the rim to the bottom as shown in fig. The velocity of the block at the bottom will be-
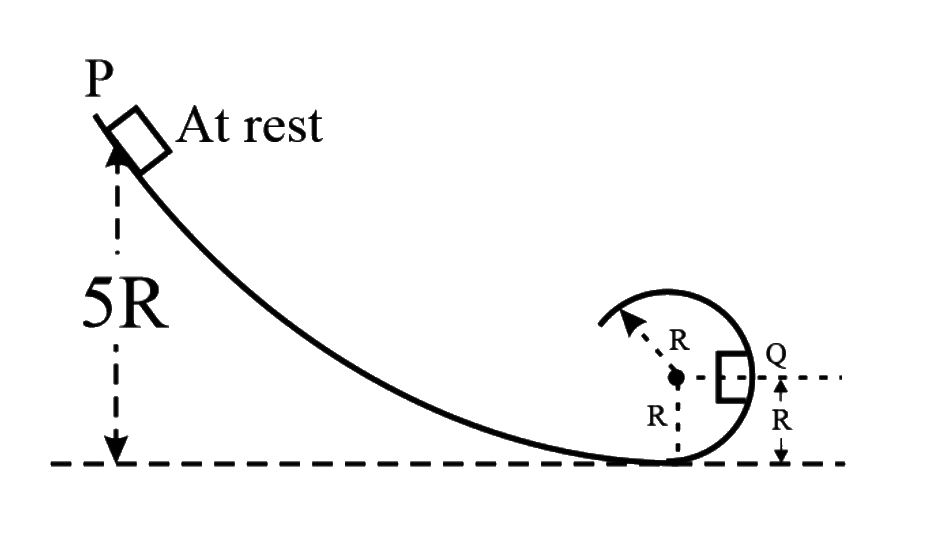
View SolutionA small block of mass m slides along a smooth frictional track as shown in the figure. If it starts from rest at P, what is the resultant force acting on it at Q?
View SolutionA block of mass m is at rest on a rough wedge as shown in figure. What is the force exerted by the wedge on the block?

View SolutionA block of mass m is at rest on a rough wedge as shown in figure. What is the force exerted by the wedge on the block?

View Solution
