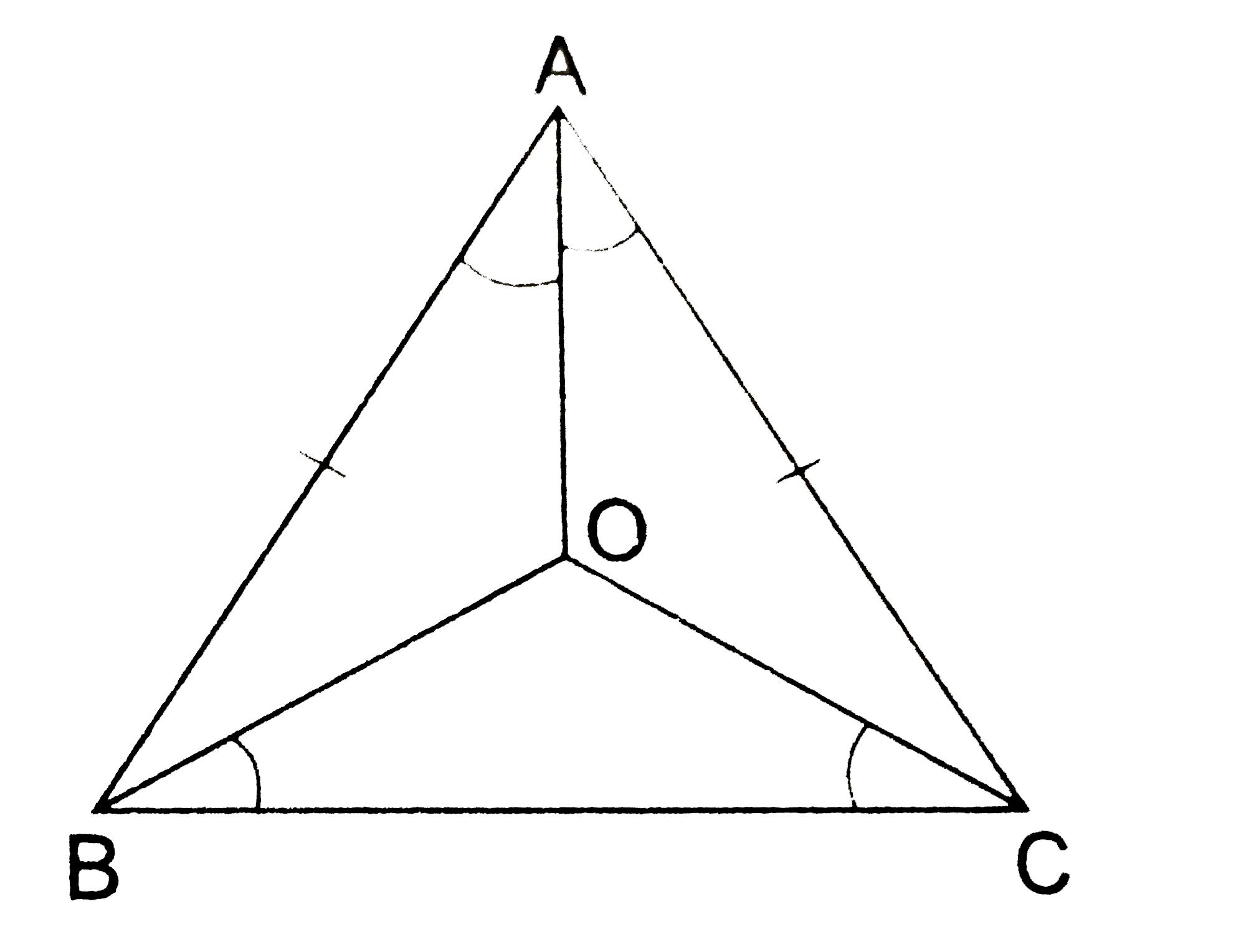
In a ΔABC, the bisectors of ∠Band∠C meet at point O within the triangle. If ∠A=132∘ then the measure of ∠BOC is(in degrees) :
166∘
84∘
156∘
120∘
The correct Answer is:D
To solve the problem, we need to find the measure of angle BOC in triangle ABC, given that angle A = 132 degrees. We will use the property of the incenter of a triangle, which is where the angle bisectors of the triangle meet.
1. Identify the Given Information:
- We have triangle ABC.
- Angle A = 132 degrees.
- O is the point where the angle bisectors of angles B and C meet.
2. Understand the Incenter Property:
- The point O is the incenter of triangle ABC.
- The angle BOC can be calculated using the formula:
Angle BOC=90∘+Angle A2
3. Substitute the Value of Angle A:
- We know angle A = 132 degrees.
- Substitute this value into the formula:
Angle BOC=90∘+132∘2
4. Calculate the Half of Angle A:
- Calculate 132∘2:
132∘2=66∘
5. Add 90 degrees to Half of Angle A:
- Now, add 90 degrees to 66 degrees:
Angle BOC=90∘+66∘=156∘
6. Final Answer:
- Therefore, the measure of angle BOC is:
Angle BOC=156∘
Conclusion:
The measure of angle BOC is 156 degrees.
- We have triangle ABC.
- Angle A = 132 degrees.
- O is the point where the angle bisectors of angles B and C meet.
- The point O is the incenter of triangle ABC.
- The angle BOC can be calculated using the formula:
Angle BOC=90∘+Angle A2
- We know angle A = 132 degrees.
- Substitute this value into the formula:
Angle BOC=90∘+132∘2
- Calculate 132∘2:
132∘2=66∘
- Now, add 90 degrees to 66 degrees:
Angle BOC=90∘+66∘=156∘
- Therefore, the measure of angle BOC is:
Angle BOC=156∘
The measure of angle BOC is 156 degrees.
Similar Questions
Knowledge Check
In a △ABC, the bisectors of ∠B and ∠C meets at point O inside the triangle. If ∠BOC=122∘, then the measure of ∠A
△ABCमें, ∠B तथा ∠C द्विभाजक त्रिभुज के भीतर बिंदु O पर मिलते हैं | यदि ∠BOC=122∘ है, तो ∠A का माप क्या होगा ?A64∘B62∘C72∘D68∘In a ΔABC, the bisectors of ∠Band∠C intersect each other point O. Then∠BOC is ?
A∠BOC=180∘+12∠A.B∠BOC=90∘+12∠A.C∠BOC=180∘−12∠A.D∠BOC=90∘−12∠A.In a △ABC, the bisectors of ∠B and ∠C meets at point O inside the triangle. If ∠BOC=122∘, then the measure of ∠A
△ABCमें, ∠B तथा ∠C द्विभाजक त्रिभुज के भीतर बिंदु O पर मिलते हैं | यदि ∠BOC=122∘ है, तो ∠A का माप क्या होगा ?A62∘B64∘C72∘D68∘
In △ABC,∠A=40∘ if bisector of ∠B and ∠C meets at O then prove that ∠BOC=110∘
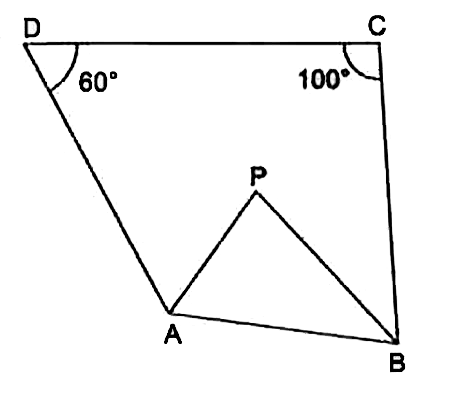
View SolutionIn the adjacent figure, the bisectors of ∠Aand∠B meet in a point P. If ∠C=100∘and∠D=60∘, find the measure of ∠APB.
View SolutionIn △ABC the bisectors of ∠B and ∠C intersect at I if ∠A=70∘ then ∠BIC=…………..
View SolutionThe internal bisectors of ∠Band∠C of ΔABC meet at O. If ∠A=80∘ then ∠BOC is :
View SolutionIn a △ABC,∠A=35∘ and ∠B=65∘,find the measure of ∠C
View Solution