Study the given information carefully and answer the following questions.
There was a football tournament of three teams i.e. A, B and C in which each team played 2 matches. Score pattern of the tournament is:
• A team gets 2 points for scoring a goal against the opponent team.
• A team gets 3 points for scoring a goal against the opponent team from the outside area.
• There is a penalty of 1 point if a team concedes a goal.
• Only three players from each team scored the goals.
A – B Match: B is the winner of this game. Total points scored by B in this match is 4 . Also, team A scored 2 goals and none of the players scored the goal from the outside area.
A – C Match: C scored 0 points in the match. Only one player from team A scored a goal from outside area. A scored 4 points in this match.
B – C Match: B gets 6 points from match. Team C scored 1 goal more than Team B. One player from team B scored a goal from outside area but none from team C.
What is the maximum possible sum of the total number of goals scored by those players of all three teams, who score more than one goal in a tournament?
18
14
12
15
The correct Answer is:D
To solve the problem step-by-step, we need to analyze the information provided about the football tournament involving teams A, B, and C.
Step 1: Analyze the A-B Match
- Winner: B
- Points Scored by B: 4
- Goals Scored by A: 2 (none from outside area)
Since A scored 2 goals, they earn 2 * 2 = 4 points. However, since B won and scored 4 points, we can deduce that B must have scored 3 goals (2 points each) and conceded 1 goal (which incurs a penalty of -1 point):
- Points for B: 3 goals * 2 points = 6 points - 1 point (for conceding 2 goals) = 5 points.
- Thus, B scored 3 goals in total.
Step 2: Analyze the A-C Match
- Points Scored by C: 0
- Points Scored by A: 4
- Goals Scored by A: 2 (1 from outside area)
Since A scored 4 points, and one of the goals was from outside, they earn:
- 1 goal from outside area = 3 points
- 1 goal from inside = 2 points
- Total = 3 + 2 = 5 points - 1 point (for conceding 1 goal) = 4 points.
Thus, A scored 2 goals, and C scored 0 goals.
Step 3: Analyze the B-C Match
- Points Scored by B: 6
- Goals Scored by C: 1 more than B
Let’s denote the goals scored by B as x. Since B scored 6 points:
- If B scored 2 goals from inside and 1 from outside, they would have:
- 1 goal from outside = 3 points
- 2 goals from inside = 2 * 2 = 4 points
- Total = 3 + 4 - 1 (for conceding 1 goal) = 6 points.
Thus, B scored 3 goals, and since C scored 1 more than B, C scored 4 goals.
Step 4: Summarize Goals Scored
- Team A: 2 goals
- Team B: 3 goals
- Team C: 4 goals
Step 5: Identify Players Who Scored More Than One Goal
- Players from Team B and Team C scored more than one goal.
- Team B: 3 goals (1 player can score all)
- Team C: 4 goals (1 player can score all)
Step 6: Calculate the Maximum Possible Sum of Goals
- Maximum goals scored by players who scored more than one goal:
- Team B: 3 goals
- Team C: 4 goals
- Total = 3 (from B) + 4 (from C) = 7
Final Answer
The maximum possible sum of the total number of goals scored by those players of all three teams who scored more than one goal in the tournament is 7.
- Winner: B
- Points Scored by B: 4
- Goals Scored by A: 2 (none from outside area)
Since A scored 2 goals, they earn 2 * 2 = 4 points. However, since B won and scored 4 points, we can deduce that B must have scored 3 goals (2 points each) and conceded 1 goal (which incurs a penalty of -1 point):
- Points for B: 3 goals * 2 points = 6 points - 1 point (for conceding 2 goals) = 5 points.
- Thus, B scored 3 goals in total.
- Points Scored by C: 0
- Points Scored by A: 4
- Goals Scored by A: 2 (1 from outside area)
- 1 goal from outside area = 3 points
- 1 goal from inside = 2 points
- Total = 3 + 2 = 5 points - 1 point (for conceding 1 goal) = 4 points.
- Points Scored by B: 6
- Goals Scored by C: 1 more than B
- If B scored 2 goals from inside and 1 from outside, they would have:
- 1 goal from outside = 3 points
- 2 goals from inside = 2 * 2 = 4 points
- Total = 3 + 4 - 1 (for conceding 1 goal) = 6 points.
- Team A: 2 goals
- Team B: 3 goals
- Team C: 4 goals
- Players from Team B and Team C scored more than one goal.
- Team B: 3 goals (1 player can score all)
- Team C: 4 goals (1 player can score all)
- Maximum goals scored by players who scored more than one goal:
- Team B: 3 goals
- Team C: 4 goals
- Total = 3 (from B) + 4 (from C) = 7
The maximum possible sum of the total number of goals scored by those players of all three teams who scored more than one goal in the tournament is 7.
Similar Questions
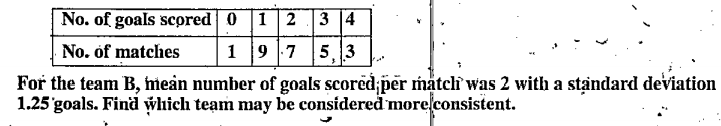
The following is the record of goals scored by team A in a football season.For the team b mean number of goals scored per match was 2 with a standard deviation of 1.25 goals. Find which team may be consider more consistent using coefficient of variation.
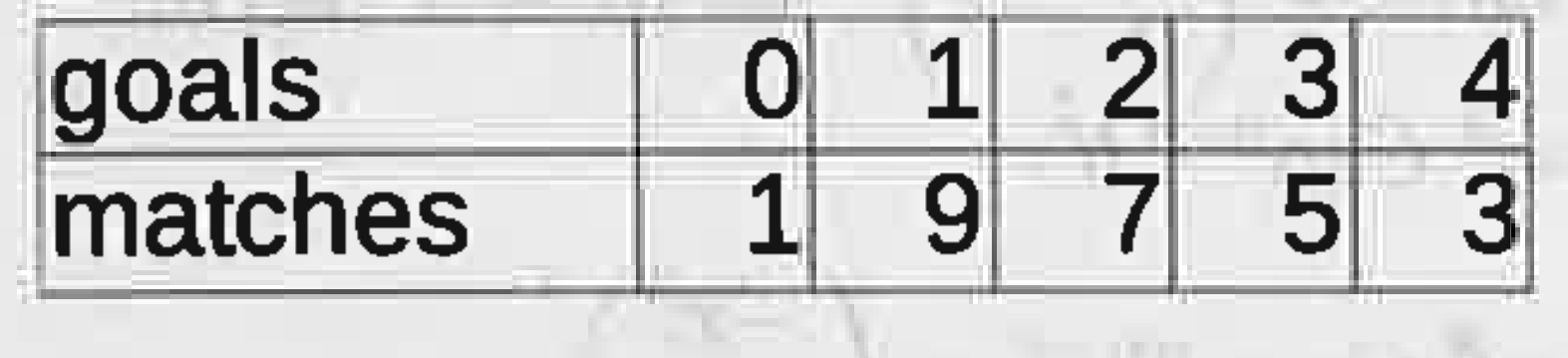
View SolutionThe following is the record of goals scored by team A in a football session

View SolutionThe following is the rècord of goals scored by team '(A)' in a football session.
View SolutionIn a tournament with five teams, each team plays against every other team exctly once. Each game is won by one of the playing teams and the winning team scores one point, while the losing team scores zero. Which of the following is NOT necessarily true?
View SolutionTwenty teams take part in a football tournament. Each team has to play every other team. How many games would be played in the tournament?
View SolutionThe line chart given below represents the runs scored by Kohli and Sharma against 5 teams.
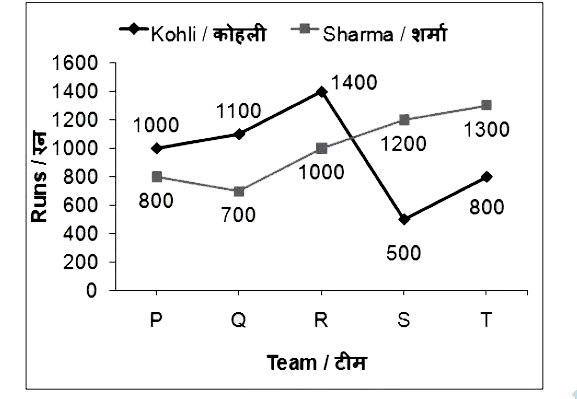
Total runs scored by Sharma against 5 teams is what percent of total runs scored by Kohli against 5 teams?
View SolutionStudy the given information carefully and answer the following questions. There was a football tournament of three teams i.e. A, B and C in which each team played 2 matches. Score pattern of the tournament is: • A team gets 2 points for scoring a goal against the opponent team. • A team gets 3 points for scoring a goal against the opponent team from the outside area. • There is a penalty of 1 point if a team concedes a goal. • Only three players from each team scored the goals. A – B Match: B is the winner of this game. Total points scored by B in this match is 4 . Also, team A scored 2 goals and none of the players scored the goal from the outside area. A – C Match: C scored 0 points in the match. Only one player from team A scored a goal from outside area. A scored 4 points in this match. B – C Match: B gets 6 points from match. Team C scored 1 goal more than Team B. One player from team B scored a goal from outside area but none from team C. The given 3 teams are ranked on the basis of total marks in such a way that the highest scoring team is ranked 1, the second highest scoring team is ranked 2 and the least scoring team is ranked 3. Rank 3 got a total of Rs. 60,000 as prize money. If the ratio of the prize money of the rank 1, rank 2 and rank 3 team is 8 : 5 : 3, which of the following combinations of team and prize money is correct?
View Solution
