A swimmer wishes to cross a 500 m wide river flowing at 5 km/h. His speed with respect to water is 3 km/h. Consider the situation of the previous problem. The man has to reach the other shore at the point directly opposite to his starting point. If the reaches the other shore somewhere else, he has to walk down to this point. Find the minimum distance that he has to walk.Solution in Tamil
The correct Answer is:B, C
Velocity of man, Vm=3kmhr
BD= horizontal distance for resultant velocity R
X- component of resultant
R=5+3cosθ
t=0.53sinθ
Which is same of horizontal component of velocity.
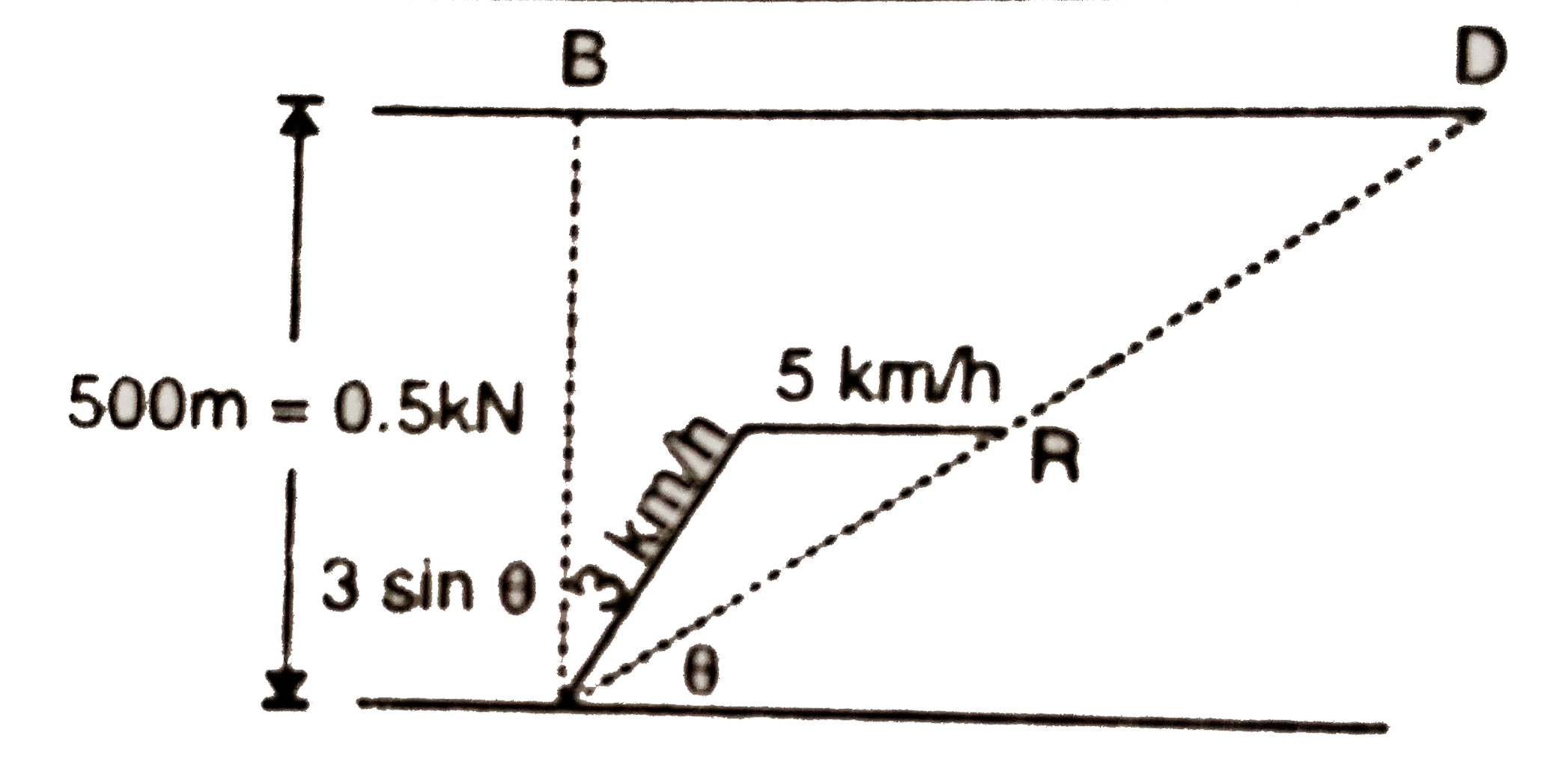
H=BD
=(5−3cosθ)(0.53sinθ)
=5+3cosθ6sinθ
For H to be minimum (dHdθ)=0
>ddθ(5+3cosθ6sinθ)=0
→18(sin2θ+cos2θ)−30cosθ=0
→30cosθ=18
→cosθ=−1830=−35
sinθ=√1−cos2θ0=45
∴H=5+3cosθ6sinθ
=5+3(35))6×45=25−924
=1624=23km.
Topper's Solved these Questions
- REST AND MOTION : KINEMATICSBOOK - HC VERMACHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Objective -2
10 Videos
- PHYSICS AND MATHEMATICSBOOK - HC VERMACHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Exercises
34 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Exercises
86 Videos
Similar Questions
Consider the motion of the tip of the minute hand of a clock. In one hour
View SolutionA vector →A makes an angle of 20∘and→B makes an angle of 110∘ with the X-axis. The magnitude of these vectors are 3 m and 4 m respectively.Find the resultant
View SolutionA wheel is making revolutions about its axis with uniform angular acceleration. Starting from rest, till it reaches 100 rev/sec in 4 seconds. Find the angular acceleration.
View SolutionA man can swim in still water ast a speed of 3 km/h. He wants to cross a river that flows at 2 km/h and reach the point directly oposite to his starting point. A. In which diretionshoeld he try to swim (that is, find the angle his body makes wilth the river flow)? b. How much time will he take to cross the river if the river is 500 m wide?
View SolutionA swimmer wishes to cross a 500 m wide river flowing at 5 km/h. His speed with respect to water is 3 km/h. The man has to reach the other shore at the point directly opposite to his starting point. If the reaches the other shore somewhere else, he has to walk down to this point. Find the minimum distance that he has to walk.
View SolutionA swimmer wishes to cross a 500−m river flowing at 5kmh−1. His speed with respect to water is 3kmh−1. The shortest possible time to cross the river is.
View SolutionA man who swims at a speed of 5km/h wants to cross a 500m wide stream flowing at 4km/h and reach the point which is directly opposite to his starting point. If he reaches a point somewhere else he has to walk back to desitination, his walking speed being 2km/h. Find the minimum time in which he can reah his destination.
View SolutionA man wishes to swim across a river 0.5km. wide if he can swim at the rate of 2 km/h. in still water and the river flows at the rate of 1km/h. The angle (w.r.t. the flow of the river) along which he should swin so as to reach a point exactly oppposite his starting point, should be:-
View Solutionएक तैराक 5 km/h वेग से बह रही 500 m चौड़ी नदी को तैरकर पार करना चाहता है ।
तैराक को पैदल या तैरकर प्रारंभिक बिंदु के ठीक सामने दूसरे किनारे पर पहुँचना है । यदि वह तैरकर इस बिंदु से दूर कही निकलता है , तो उसे पैदल चलकर इस बिंदु तक आना पड़ेगा । उसे कम-से-कम कितनी दुरी पैदल चलना पड़ेगा ?
View SolutionA man can swim in still water at a speed of 6 kmph and he has to cross the river and reach just opposite point on the other bank. If the river is flowing at a speed of 3 kmph, he has to swim in the direction
View SolutionA man can swim in still water at a speed of 6 kmph and he has to cross the river and reach just opposite point on the other bank. If the river is flowing at a speed of 3 kmph, he has to swim in the direction
View SolutionA man can swim in still water ast a speed of 3 km/h. He wants to cross a river that flows at 2 km/h and reach the point directly oposite to his starting point. A. In which diretionshoeld he try to swim (that is, find the angle his body makes wilth the river flow)? b. How much time will he take to cross the river if the river is 500 m wide?
View SolutionA swimmer wishes to cross a 500m river flowing at 5kmh−1. His speed with respect to water is 3kmh−1. The shortest possible time to cross the river is.
View SolutionA man can swim in still water at a speed of 6 kmph and he has to cross the river and reach just opposite is point on the other bank. If the river is flowing at a speed of 3 kmph, he has to swim in the direction
View SolutionA man who swims at a speed of 5km/h wants to cross a 500m wide stream flowing at 4km/h and reach the point which is directly opposite to his starting point. If he reaches a point somewhere else he has to walk back to desitination, his walking speed being 2km/h. Find the minimum time in which he can reah his destination.

View SolutionA man can swim in still water at a speed of 6 kmph and he has to cross the river and reach just opposite point on the other bank. If the river is flowing at a speed of 3 kmph, he has to swim in the direction
View Solution
