बल नियतांक वाली एक स्प्रिंग को दो असमान लम्बाई l1 और l2 में काटा गया है। जहाँ (l1l2=n1n2) है तो प्रत्येक भाग के लिए बल नियतांक ज्ञात कीजिए।
लम्बाई की स्प्रिंग को l1 व l2 लम्बाई के दो भागों में काटा गया है।
स्प्रिंग बल नियताक ∝=1स्प्रिंग की लम्बाई
∴K∝1l ⇒k=Cl (C=नियत)
K1=Cl1,k2=Cl2 तथा k=Cl=Cl1+l2
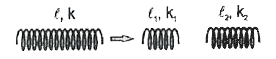
K1K=l1+l2l1 ⇒K1=k[1+l2l1 तथा
K2K=l1+l2l2 ⇒k2=k[1+l1l2]
∴l1l2=n1n2 So,K1=k[1+n2n1]
⇒k1=k[n1+n2n1] तथा k2=k[1+n1n2]
⇒k2=k[n1+n2n2
Similar Questions
If (l1,m1,n1),(l2,m2,n2) are d.c's of two lines whose angle between them is 30∘, then d.c's of a line perpendicular to both these lines are k(m1n2−m2n1,n1l2−n2l1,l1m2−l2m1), k =
View SolutionIf (l1,m1,n1),(l2,m2,n2) are d.c's of two lines then find the value of (l1m2−l2m1)2+(m1n2−n1m2)2+(n1l2−n2l1)2+(l1l2+m1m2+n1n2)2
View SolutionIf the direction cosines of two lines are l1,m1,n1 and l2,m2,n2, then find the direction cosine of a line perpendicular to these lines.
a)l1+l2,m1+m2,n1+n2
b)l1−l2,m1−m2,n1−n2
c)m1n2−m2n1,n1l2−n2l1,l1m2−l2m1
d)l1+2l2,m1+2m2,n1+2n2
View Solutionरेखाओ के समीकरणो
L1=x+3y−5=0
L2=3x−ky−1=0
L3=5x+2y−12=0
पर विचार कीजिये।
स्तम्भ - I से स्तम्भ - II का मिलान कीजिये तथा अपने उत्तर को 4 xx 4 के आव्यूह के रूप में उत्तर पुस्तिका पर अंकित कीजिये।
List - I List - II (A) L1,L2,L3 संगामी है यदि (P) k = - 9 (B) L1,L2,L3 में से कोई एक, शेष दो में से कम से कम एक के समान्तर है, यदि (Q) k=−65(C) L1,L2,L3एक त्रिभुज बनती है, यदि (R) k=56(D) L1,L2,L3 एक त्रिभुज नहीं बनती है , यदि (S)k=5
View SolutionIf A =⎡⎢⎣l1m1n1l2m2n2l3m3n3⎤⎥⎦ then Find A+I
View SolutionIf (l1,m1,n1),(l2,m2,n2) are D.C's of two lines, then (l1m2−l2m1)2+(m1n2−n1m2)2+(n1l2−n2l1)2+(l1l2+m1m2+n1n2)2=
View Solution
If the direction cosines of two lines are (l1,m1,n1) and
(l2,m2,n2) and the angle between them is θ then
l21+m21+n21=1=l22+m22+n22
and costheta=l1l2+m1m2+n1n2
If the angle between the lines is 60∘ then the value
of l1(l1+l2)+m1(m1+m2)+n1(n1+n2) is
View SolutionIf l_(1), m_(1), n_(1) and l_(2), m_(2), n_(2) are the direction cosines of two mutually perpendicular lines show that the Direction Cosines of the line perpendicular to both of them are m_(1)n_(2) - n_(1)m_(2), n_(1)l_(2) - l_(1)n_(2), l_(1)m_(2) - m_(1)l_(2)
View Solution
