For every question, four probable answers bearing (A), (B), (C) and (D) given. Only one out of these is correct. You have to choose the correct answer.
LCM of 42, 70, 98 and 126 is
126
2205
4410
8820
The correct Answer is:C
To find the LCM (Least Common Multiple) of the numbers 42, 70, 98, and 126, we can follow these steps:
Step 1: Prime Factorization
First, we need to find the prime factorization of each number.
- 42:
- 42 = 2 × 3 × 7
- 70:
- 70 = 2 × 5 × 7
- 98:
- 98 = 2 × 7 × 7 = 2 × 7²
- 126:
- 126 = 2 × 3 × 3 × 7 = 2 × 3² × 7
Step 2: Identify the Highest Powers of Each Prime Factor
Next, we identify the highest power of each prime factor from the factorizations:
- For 2, the highest power is 21 (from all numbers).
- For 3, the highest power is 32 (from 126).
- For 5, the highest power is 51 (from 70).
- For 7, the highest power is 72 (from 98).
Step 3: Multiply the Highest Powers Together
Now, we multiply these highest powers together to find the LCM:
LCM=21×32×51×72
Calculating this step-by-step:
1. Calculate 32=9.
2. Calculate 72=49.
3. Now multiply:
- 2×9=18
- 18×5=90
- 90×49=4410
Final Result
Thus, the LCM of 42, 70, 98, and 126 is 4410.
Answer
The correct answer is (C) 4410.
---
First, we need to find the prime factorization of each number.
- 42 = 2 × 3 × 7
- 70:
- 70 = 2 × 5 × 7
- 98:
- 98 = 2 × 7 × 7 = 2 × 7²
- 126:
- 126 = 2 × 3 × 3 × 7 = 2 × 3² × 7
Next, we identify the highest power of each prime factor from the factorizations:
- For 3, the highest power is 32 (from 126).
- For 5, the highest power is 51 (from 70).
- For 7, the highest power is 72 (from 98).
Now, we multiply these highest powers together to find the LCM:
2. Calculate 72=49.
3. Now multiply:
- 2×9=18
- 18×5=90
- 90×49=4410
Thus, the LCM of 42, 70, 98, and 126 is 4410.
The correct answer is (C) 4410.
Similar Questions
For every question, four probable answers bearing (A), (B), (C) and (D) given. Only one out of these is correct. You have to choose the correct answer.
Which of the following is the smallest four digit number?View SolutionFor every question, four probable answers bearing (A), (B), (C) and (D) given. Only one out of these is correct. You have to choose the correct answer.
Convert 4 metres 2604 centimetres into centimetres.View Solution
Knowledge Check
- Question 1 - Select One
For every question, four probable answers bearing (A), (B), (C) and (D) given. Only one out of these is correct. You have to choose the correct answer.
Which one of the following is a prime number?A81B83C85D87 - Question 2 - Select One
For every question, four probable answers bearing (A), (B), (C) and (D) given. Only one out of these is correct. You have to choose the correct answer.
The multiple of 7 between 14 and 77 isA10B9C8D7 - Question 3 - Select One
For every question, four probable answers bearing (A), (B), (C) and (D) given. Only one out of these is correct. You have to choose the correct answer.
The sum total of 975, 983, 923, 913 and 985 to its nearest hundred will beA4500B4600C4700D4800
For every question, four probable answers bearing (A), (B), (C) and (D) given. Only one out of these is correct. You have to choose the correct answer.
What value must be given to • so that the number 6912• is divisible by 25?View SolutionFor every question, four probable answers bearing (A), (B), (C) and (D) given. Only one out of these is correct. You have to choose the correct answer.
Eighty thousand nine hundred and five is represented in number form asView SolutionFor every question, four probable answers bearing (A), (B), (C) and (D) given. Only one out of these is correct. You have to choose the correct answer.
How many days are there in 2 months, 5 weeks and 18 days?View SolutionFor every question, four probable answers bearing (A), (B), (C) and (D) given. Only one out of these is correct. You have to choose the correct answer.
The difference between the place values of two 7s in 27307 isView SolutionFor every question, four probable answers bear in letters (A), (B), (C) and (D) are given. Only one out of these is correct. You have to choose the correct answer.
Profit of company in 2006 will be how much time of the profit in 2002
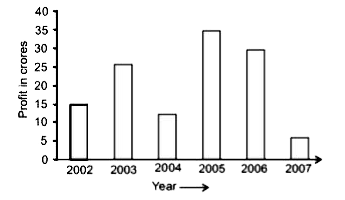 View Solution
View Solution
