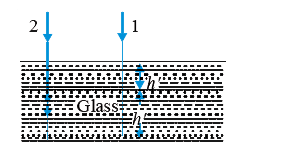
Two beam of lights are incident normally on water (μ=4/3). If the beam 1 passes through a glass (μ=3/2) slab of height h as shown in the figure, the time difference for both the beam for reaching the bottom is:
Zero
h6C
6hC
h6C
The correct Answer is:D
The refractive index of glass is greater than that of water. Therefore the speed of light in glass is less.than that of water. It is given as
v=Cn,where C=3×108m/s
v=speed of light in a medium of R.I.n
:.The time difference for the rays
Δt=t1−t2=hvg−hvw=hC/ng−hCw/nw=hCng−nw
⇒Δt=hC[32−43]=h6C
Hence(D) is correct.
Similar Questions
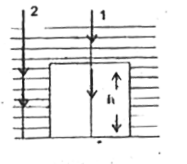
Two beams of light are incident normally on water (R.l. = 43). If the beam 1 passes through a glass (R.l. = 32) slab of height h as shown in the figure, the time difference for both beams for reaching the bottom is
View SolutionTwo parallel beams of light P and Q are incident normally on a prism and the transmitted rays are brought to focus with the help of a convergent lens as shown in Fig. 2.23. If the intensities of the upper and lower beams immediately after transmission from face AC are 4I abd I, respectively, find the resultant intensity at the focus.
View SolutionA beam of light falls on a glass plate (μ=3/2) of thickness 6.0 cm at an angle of 60∘. Find the deflection of the beam on passing through the plate. (in cm).
View SolutionTwo parallel rays are travelling in a medium of refractive index μ1=4/3. One of the rays passes through a parallel glass slab of thickness t and refractive index μ2=3/2. The path difference between the two rays due to the glass slab will be
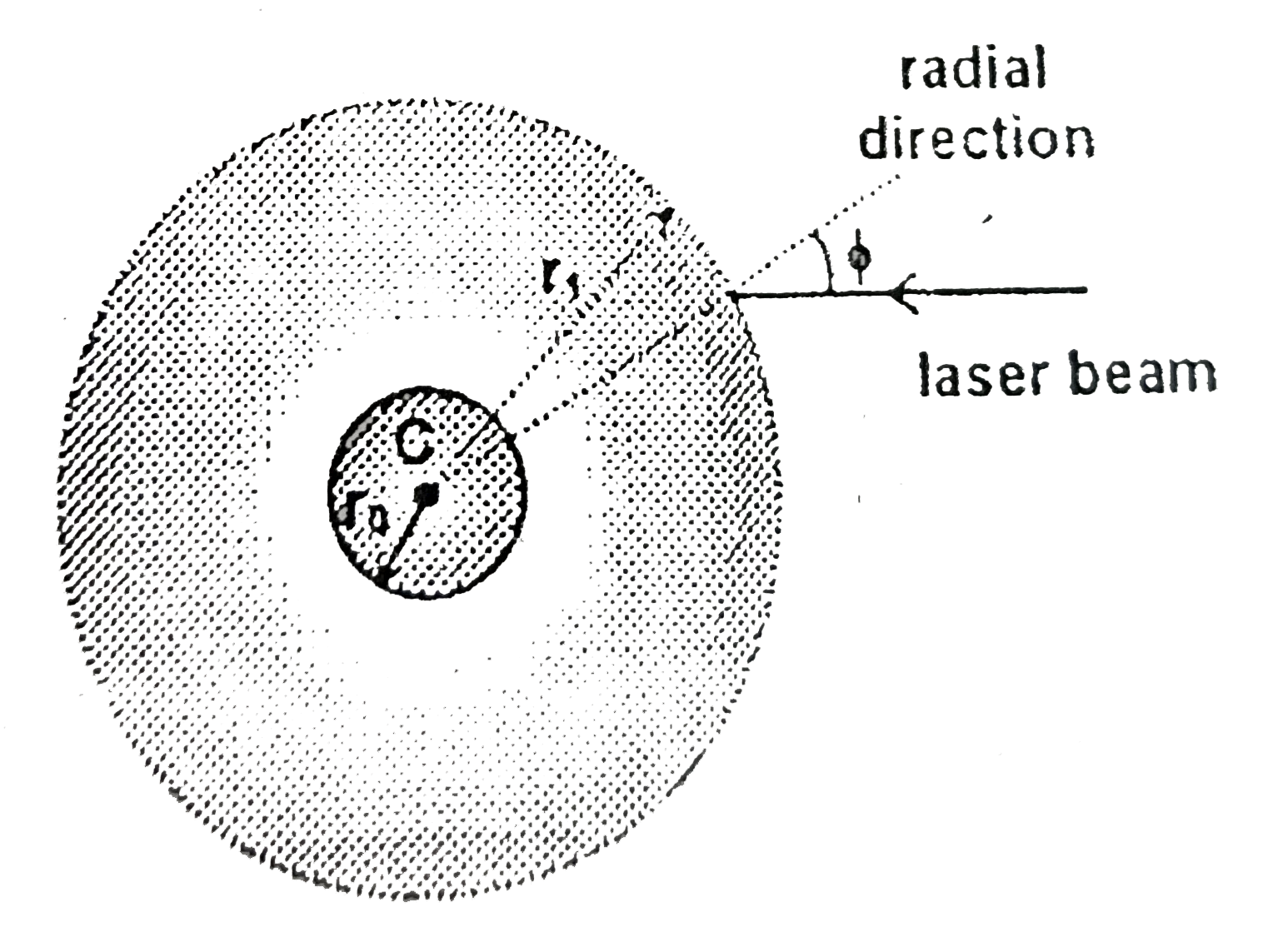
View SolutionA laser beam propagates through a spherically symmetric medium, as shown in the figure. The refractive index varies with the distance to the symmetry centre C by the law μ(r)=μ0rr0, where μ0=1, r0=3cm, r0≤r<∞. The beam's trajectory lies in the plane that includes C. At distance r1=8√2cm the beam makes an angle ϕ=30∘ with →r1 as shown in the figure. Find the minimum distance (in cm) the beam reach relative to the symmetry centre C.
View SolutionA beam of light is incident through the holes on side A and emerges out of the holes on the other face of the box as shown in Figure. Which of the following could be inside the box ?
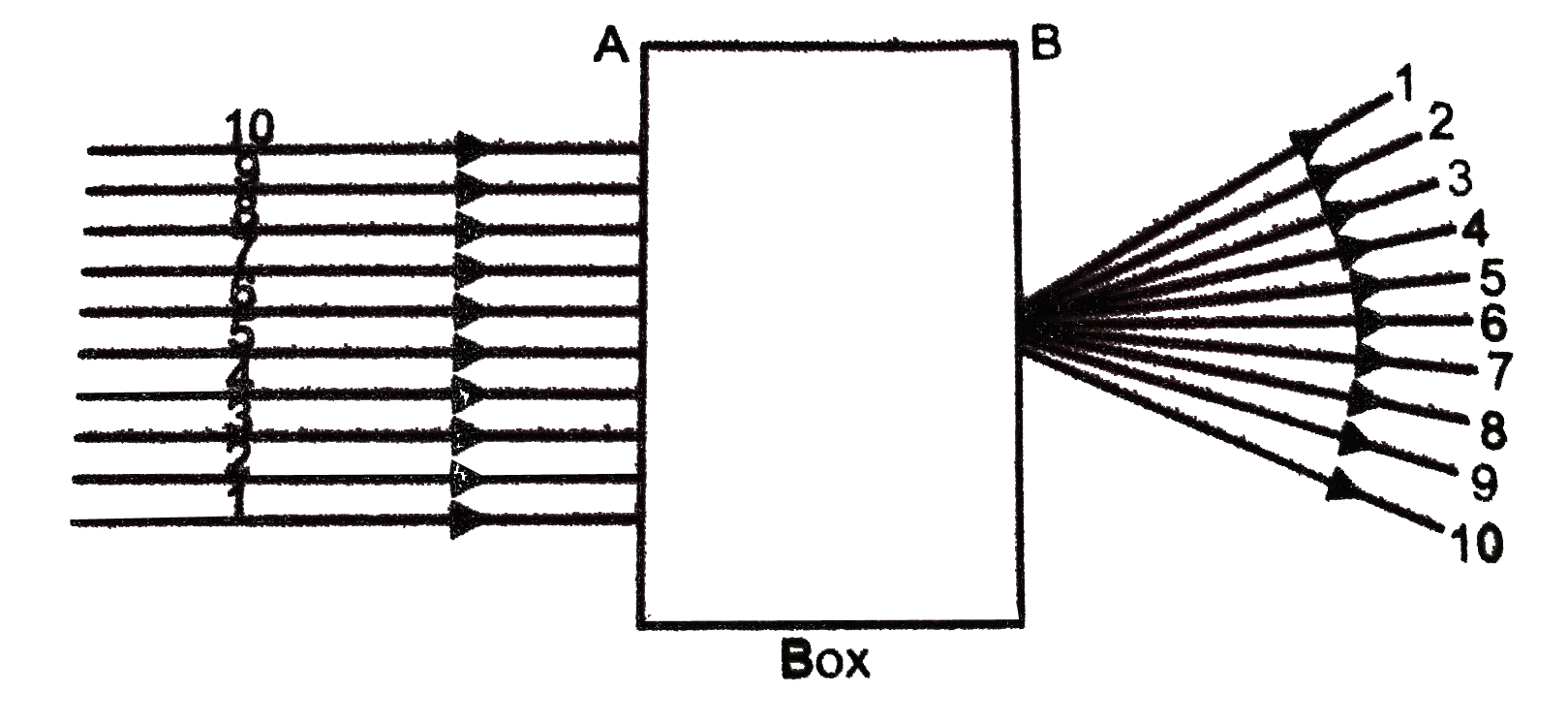
View SolutionA ray of light passes normally through a slab (μ=1.5) of thickness t. If the speed of light in vacuum be C, then time taken by the ray to go across the slab will be
View SolutionLight is incident from glass (μg=32) to water (μw=43). Find the range of the angle of deviation for refracted light.
View SolutionA prism of glass (μ=1.5) is dipped in to water as shown in the figure. If the refractive index of water is 4/3, then the incident ray will be totally reflected if

View SolutionBeams of light are incident through the holes A and B and emerge out of box through the holes C and D respectively as shown in Figure. Which of the following could be inside the box ?
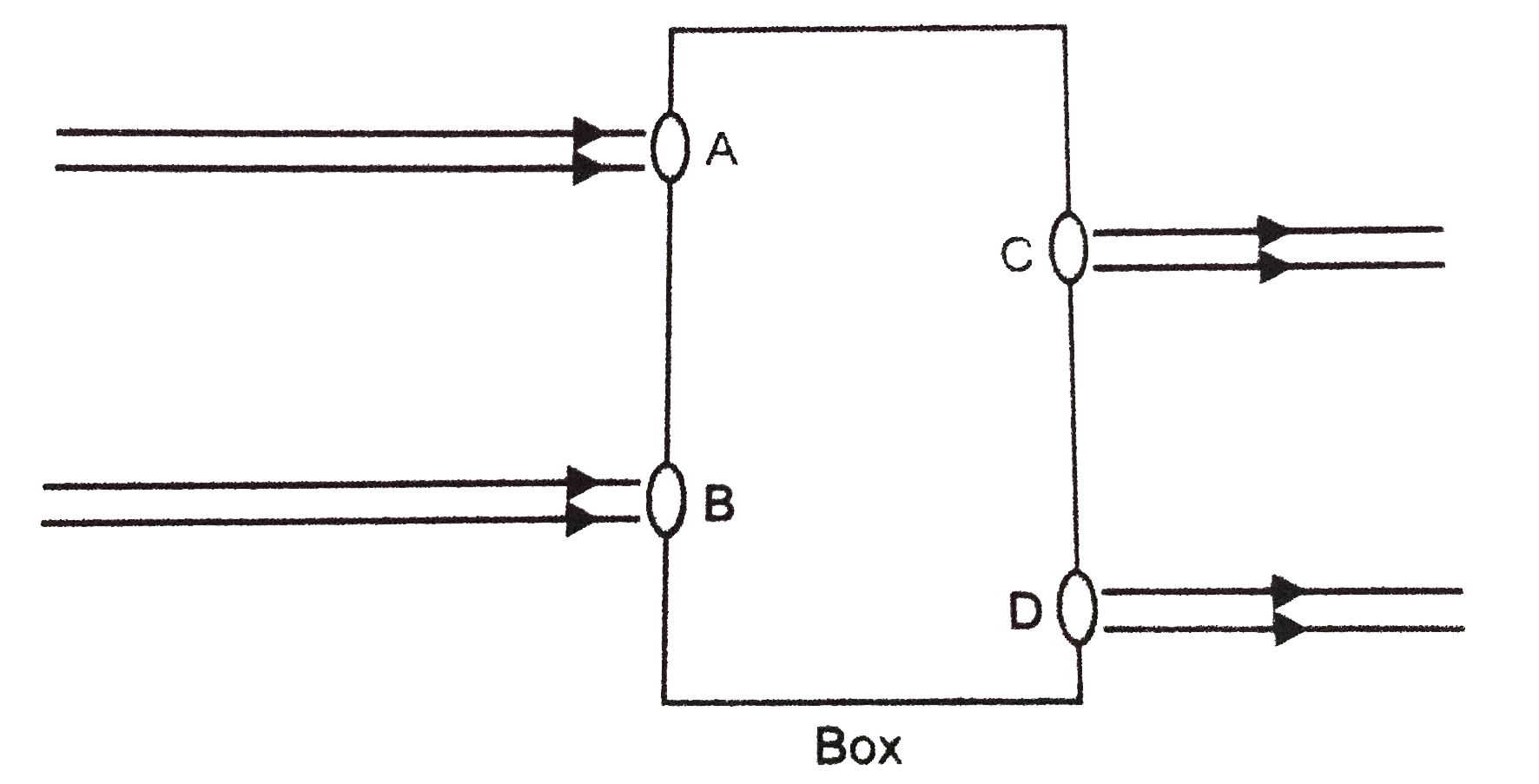
View Solution
