Nuclei of a radioactive element A are being produced at a constant rate α. The element has a decay constant λ. At time t=0, there are N0 nuclei of the element.
(a) Calculate the number N of nuclei of A at time t.
(b) If α=2N0λ, calculate the number of nuclei of A after one half-life of A, and also the limiting value of N as t→∞.
(i)X→A→B Net rate of formulation of A isdNdt=α−λN, ∫dNα−λN=∫dt+Cwhere C is a constant of integration, -1λIn(α−λN)=t+C,At t=0,N=N0,C=−1λIn(α−λN0), or t=1λIn(α−λN0α−λN)orN=(alpha-(alpha-lambdaN_(0))e ^(-mu))/(lambda)(ii)Whenalpha=2N_(0) lambda and t=T_((1)/(2))=(In2)/(lambda), implies N=(2N_(0)lambda-(2N_(0)lambda-lambdaN_(0))e^(-In2)=(3N_(0))/(2),Also,t rarr infty, N=2N_(0)
Similar Questions
Nuclei of a radioactive element X are being produced at a constant rate K and this element decays to a stable nucleus Y with a decay constant λ and half-life T1/3. At the time t=0, there are N0nuclei of the element X.
The number NY of nuclei of Y at time t is .
View SolutionNuclei of a radioactive element X are being produced at a constant rate K and this element decays to a stable nucleus Y with a decay constant λ and half-life T1/2. At the time t=0, there are N0nuclei of the element X.
The number NX of nuclei of X at time t=T1/2 is .
View Solution
Knowledge Check
Nuclei of a radioactive element A are being produced at a constant rate α. The element has a decay constant λ. At time t=0, there are N0 nuclei of the element.
(a) Calculate the number N of nuclei of A at time t.
(b) If α=2N0λ, calculate the number of nuclei of A after one half-life of A, and also the limiting value of N as t→∞.A1λ[α+(α−N0λ)e−λt]B1λ[α−(α−N0λ)e−λt]Cλ[α−(α−N0λ)e−λt]D[α−(N0λ−α)e−λt]
Nuclei of radioactive element A are produced at rate t2 (where t is time) at any time t. The element A has decay constant λ. Let N be the number of nuclei of element A at any time t. At time t=t0,dN/dt is minimum. The number of nuclei of element A at time t=t0 is
View SolutionA rodiactive nucleus is being produced at a constant rate α per second Its decay constant is λ If N0 are the number of nuclie at tieme t=O then, maximum number of nuclei possible are
View SolutionA radioacitve nucleus is being produced at a constant rate α per second. Its decay constant is λ. If N0 are the number of nuclei at time t=0, then maximum number of nuceli possible are .
View SolutionA radioactive substance (A) is produced at a constant rate which decays with a decay constant λ to form stable substance (B).If production of A starts at t=0 . Find (i)The number of nuclei of A and (ii)Number of nuclei of B at any time t.
View SolutionA radioactive sample undergoes decay as per the following gragp. At timet=0, the number of undecayed nuclei is N0. Calculate the number of nuclei left after 1h.
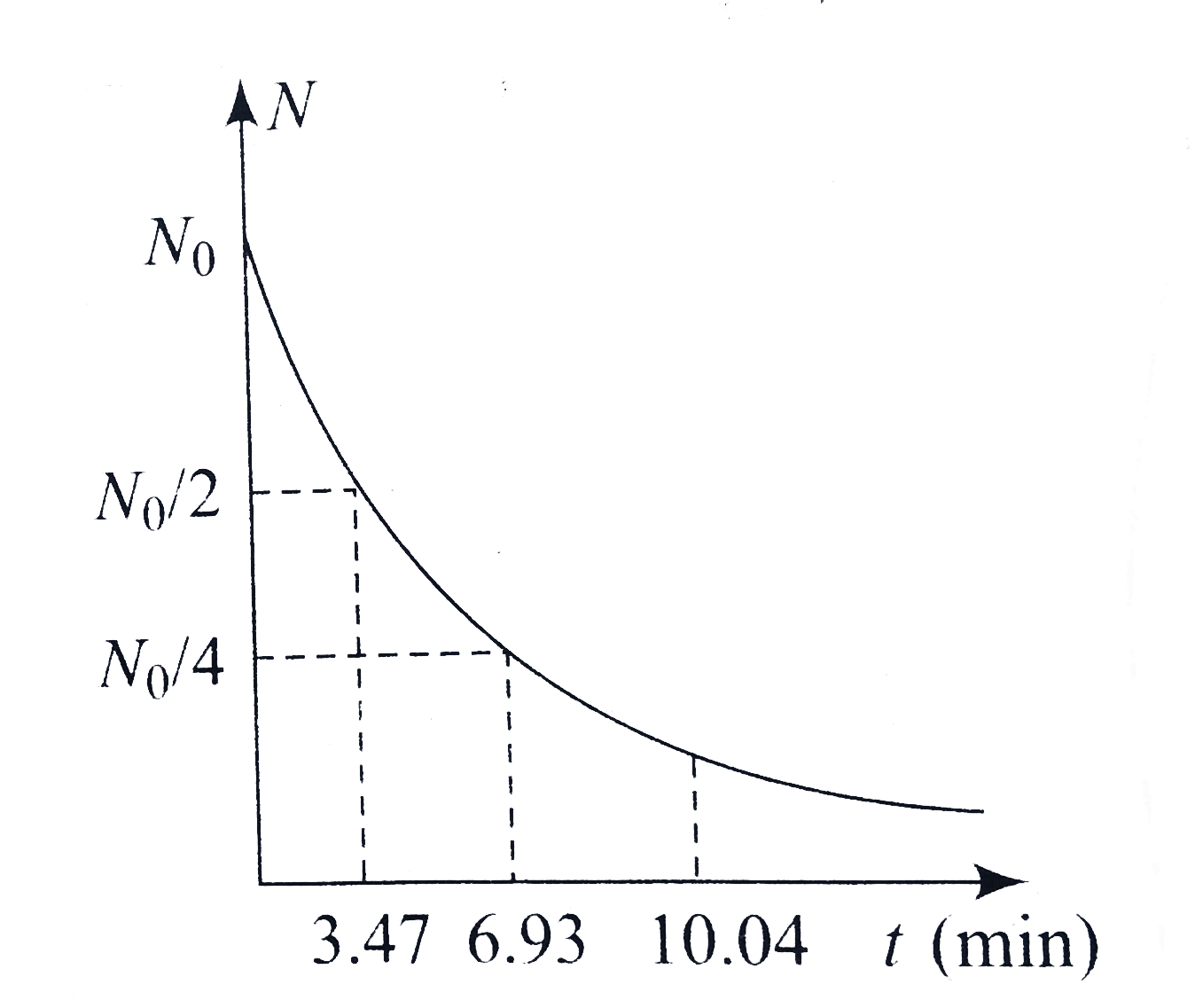 .
.
View SolutionA radioactive sample undergoes decay as per the following gragp. At timet=0, the number of undecayed nuclei is N0. Calculate the number of nuclei left after 1h.
 .
.
View SolutionRadioactive material 'A' has decay constant '8λ' and material 'B' has decay constant 'lamda'. Initial they have same number of nuclei. After what time, the ratio of number of nuclei of material 'A' to that 'B' will be '(1/ e) ?
View Solution
