Maximum pressure difference between inner and outer surface of a thin walled circular tube of radius r and thickness Δr(Δr<<r) so that tube won't break is (Breaking stress of the material is σ0)
σ0rΔr
Δrσ0r
2σ0rΔ
σ0
The correct Answer is:B
To solve the problem of finding the maximum pressure difference between the inner and outer surfaces of a thin-walled circular tube, we can follow these steps:
1. Understanding the Setup:
- We have a thin-walled circular tube with an inner radius r and thickness Δr (where Δr≪r).
- The breaking stress of the material is given as σ0.
2. Identify Forces Acting on the Tube:
- At any cross-section of the tube, there is tension T acting on the material due to the pressure difference between the inner and outer surfaces.
3. Relating Tension to Pressure Difference:
- The net tension acting towards the center of the tube can be expressed in terms of the pressure difference ΔP and the area A of the cross-section.
- The force due to the pressure difference can be given as:
F=ΔP⋅A
4. Area of the Cross-section:
- The area A for the thin-walled tube can be approximated as:
A≈2πrΔr
5. Using the Breaking Stress:
- The breaking stress σ0 is defined as the maximum tension per unit area that the material can withstand:
σ0=TA
- Rearranging gives:
T=σ0⋅A
6. Substituting for Area:
- Substitute the expression for area into the tension equation:
T=σ0⋅(2πrΔr)
7. Relating Tension to Pressure Difference:
- The net force due to the pressure difference can also be expressed in terms of tension:
ΔP⋅A=T
- Substituting for A:
ΔP⋅(2πrΔr)=T
8. Combining the Equations:
- Now, substituting the expression for T:
ΔP⋅(2πrΔr)=σ0⋅(2πrΔr)
9. Solving for Pressure Difference:
- Canceling 2πrΔr from both sides (assuming Δr≠0):
ΔP=σ0
Final Result:
Thus, the maximum pressure difference ΔP that the tube can withstand without breaking is:
ΔP=σ0
- We have a thin-walled circular tube with an inner radius r and thickness Δr (where Δr≪r).
- The breaking stress of the material is given as σ0.
- At any cross-section of the tube, there is tension T acting on the material due to the pressure difference between the inner and outer surfaces.
- The net tension acting towards the center of the tube can be expressed in terms of the pressure difference ΔP and the area A of the cross-section.
- The force due to the pressure difference can be given as:
F=ΔP⋅A
- The area A for the thin-walled tube can be approximated as:
A≈2πrΔr
- The breaking stress σ0 is defined as the maximum tension per unit area that the material can withstand:
σ0=TA
- Rearranging gives:
T=σ0⋅A
- Substitute the expression for area into the tension equation:
T=σ0⋅(2πrΔr)
- The net force due to the pressure difference can also be expressed in terms of tension:
ΔP⋅A=T
- Substituting for A:
ΔP⋅(2πrΔr)=T
- Now, substituting the expression for T:
ΔP⋅(2πrΔr)=σ0⋅(2πrΔr)
- Canceling 2πrΔr from both sides (assuming Δr≠0):
ΔP=σ0
Thus, the maximum pressure difference ΔP that the tube can withstand without breaking is:
ΔP=σ0
Similar Questions
Maximum excess pressure inside a thin-walled steel tube of radius r and thickness △r(<<r), so that the tube would not rupture would be (breaking stress of steel is σmax
View SolutionThe maximum surface charge density of a uniformly charged spherical radius R and wall thickness 't', so that it will not burst apart, is
View SolutionA circular ring of radius R and mass m made of a uniform wire of cross sectional area A is rotated about a stationary vertical axis passing throgh its centre and perpendicular to the plane of the ring. If the breaking stress of the material of the ring is σb, then determine the maximum angular speed ωmax at which the ring may be rotated without failure.
View SolutionA pipe of circular cross-section of inner radius r and outer radius 2r is bent into a semi circle as shown in the diagram. A fluid of density ρ=103kg/m3 is flowing through it. The breaking stress of the material of the pipe is 3×107n/m2. The maximum velocity with which the fluid can flow in the pipe is k×100m/s. find value of k.

View SolutionWhen a viscous liquid flows , adjacent layers oppose their relative motion by applying a viscous force given by
F=−ηAdvdz
where , ete = coefficient of viscosity ,
A = surface area of adjacent layers in contact ,
dvdz = velocity gradient
Now , a viscous liquid having coefficient of viscosity η is flowing through a fixed tube of length l and radius R under a pressure difference P between the two ends of the tube . Now consider a cylindrical vloume of liquid of radius r . Due to steady flow , net force on the liquid in cylindrical vloume should be zero .
−η2πrldvdr=Pπr2
−∫0v,dv=P2ηl∫Rτrdr(∵ layer in contact with the tube is stationary )
v=v0(1−r2R2), where v0=PR24nl
∴ Q=πPR48stal

This is called Poisecuille's equation .
The volume of the liquid flowing per sec across the cross - section of the tube is .
View SolutionWhen a viscous liquid flows , adjacent layers oppose their relative motion by applying a viscous force given by
F=−ηAdvdz
where , eta = coefficient of viscosity ,
A = surface area of adjacent layers in contact ,
dvdz = velocity gradient
Now , a viscous liquid having coefficient of viscosity η is flowing through a fixed tube of length l and radius R under a pressure difference P between the two ends of the tube . Now consider a cylindrical vloume of liquid of radius r . Due to steady flow , net force on the liquid in cylindrical volume should be zero .
−η2πrldvdr=Pπr2
−∫0v,dv=P2ηl∫Rτrdr(∵ layer in contact with the tube is stationary )
v=v0(1−r2R2) , where v0=PR24nl
∴ Q=πPR48stal
This is called Poisecuille's equation .
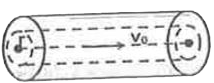
The velocity of flow of liquid at r = R/2 is
View SolutionA cylindrical tube of length l has inner radius a while outer radius b. What is the resistance of the tube between (a) its ends (b) inner and outer surfaces ? (The resistivitiy of its material is p)
View SolutionA cylinderical tube of length L has inner radius a and outer radius b. What is the resistance of the tube between its inner and outer surface ? Given that the resistivity of its material is ρ.
View SolutionThe weight of a body at earth's surface is W. At a depth half way to the centre of earth it will weight
View SolutionA sphere of density d, specific heat s and radius r is hung by a thermally insulating thread in an enclosure which is kept at a lower temperature than the sphere. The temperature of the sphere starts to drop at a rate which depends upon the temperature difference between the sphere and the enclosure. If the temperature difference is ΔT and surrounding temperature is T0 then rate of fall in temperature will be
[Given that ΔT<<T0]
View Solution
