The sum of two forces is 18 N and resultant whose direciton is at right angles to the smaller force is 12 N. the magnitude of the two forces are

A

6N & 10N

B

8N & 8N

C

4N & 12N

D

2N & 14N

Video Solution

Text Solution

Verified by Experts

The correct Answer is:1

$F_{1} + F_{2} = 16$ ...(i)
$8 = \sqrt{F_{1}^{2} + F_{2}^{2} + 2 F_{1} F_{2} cos θ}$
$\Rightarrow F_{1}^{2} + F_{2}^{2} + 2 F_{1} F_{2} cos θ = 64$ …(ii)

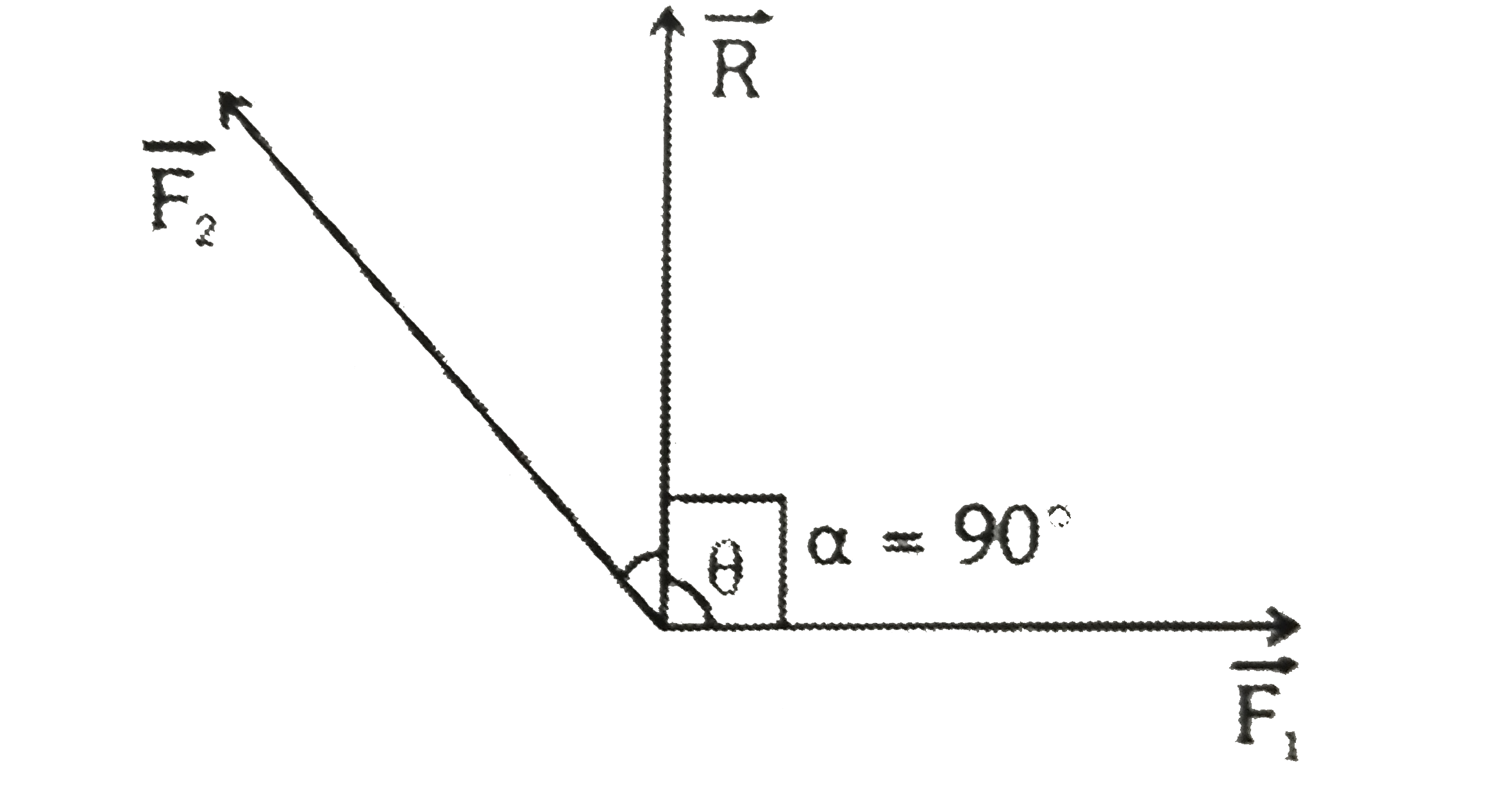
$tan α = \frac{F_{2} sin θ}{F_{1} + F_{2} cos θ} = {tan 90}^{\circ} = \infty$
$F_{2} cos θ = - F_{1}$ ...(iii)
Solving (i), (ii) and (iii) $\Rightarrow F_{1} = 6 N, F_{2} = 10 N$

|

Updated on:21/07/2023

Class 11 PHYSICS BASIC MATHEMATICS USED IN PHYSICS &VECTORS

Topper's Solved these Questions

Similar Questions

ALLEN-BASIC MATHEMATICS USED IN PHYSICS &VECTORS -EXERCISE-III CHECK YOUR UNDERSTANDING

Home
Ask Doubt
Profile