The velocity of body is given as v=20+0.1t2. The body is undergoing
uniform acceleration
uniform retardation
non-uniform acceleration
zero acceleration
The correct Answer is:C
v=20+0.1t2
a=dvdt=0.1×2t=0.2t
as acceleration depends on time and it is increaesing with time, hence it is non uniform acceleration.
Topper's Solved these Questions
- MOTION IN A PALNEBOOK - ALLENCHAPTER - MOTION IN A PALNEEXERCISE - EXERCISE-3
40 Videos
- MOTION IN A PALNEBOOK - ALLENCHAPTER - MOTION IN A PALNEEXERCISE - SOLVED EXAMPLE
28 Videos
- MOTION IN A PALNEBOOK - ALLENCHAPTER - MOTION IN A PALNEEXERCISE - EXERCISE-1
99 Videos
- KINEMATICS-2DBOOK - ALLENCHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-2)
46 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (JA)
4 Videos
Similar Questions
The velocity of a body depends on time according to the equative v=20+0.1t2. The body is undergoing
View SolutionThe velocity of a body is given by v=At2+Bt+C. If v and t are expressed in SI, what are the units of A,B and C?
View SolutionThe velocity-time graph of a body is given in. The maximum acceleration in ms−1 is .
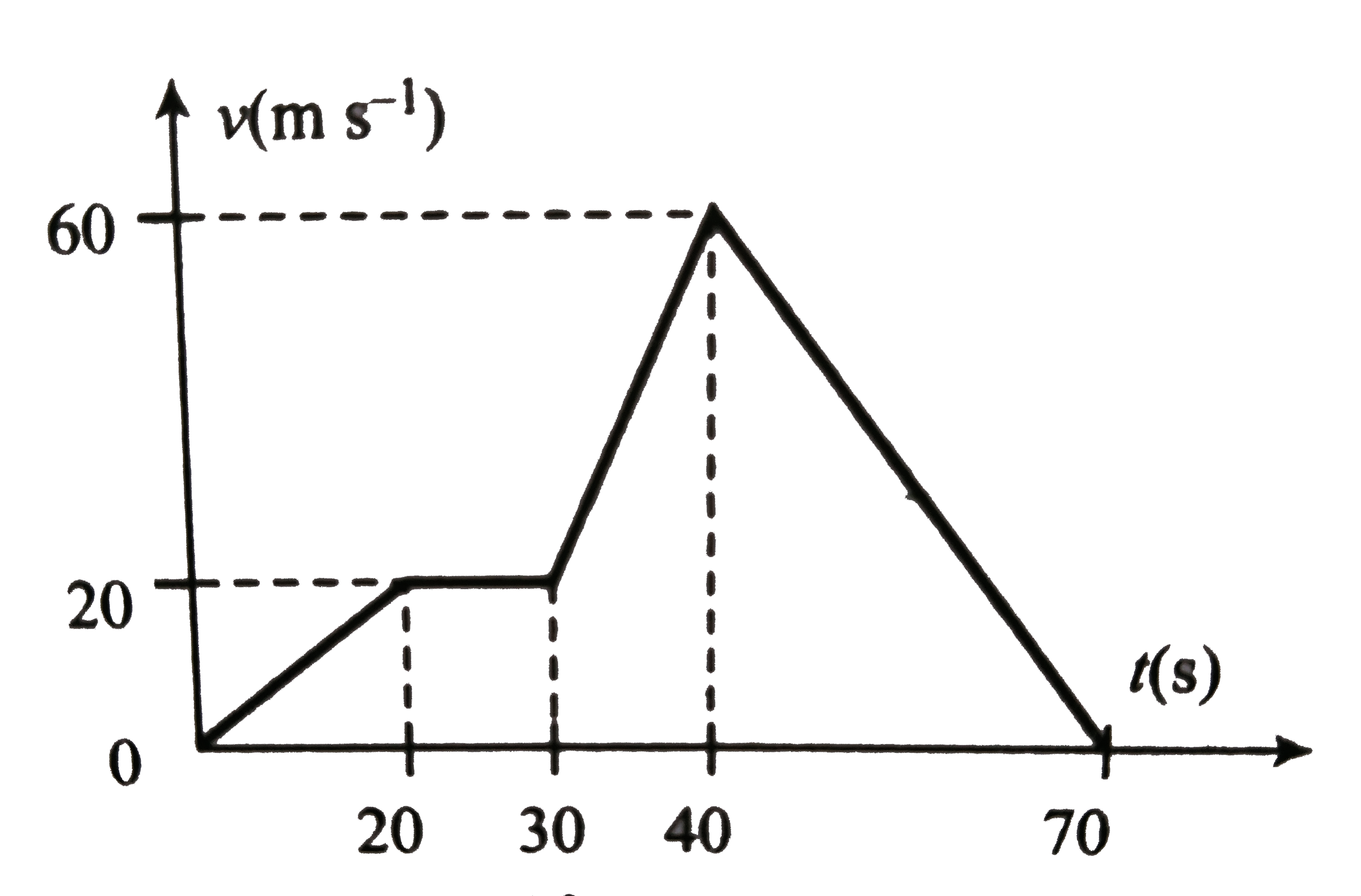 .
.
View SolutionThe displacement of a body is given by s=12gt2 where g is acceleration due to gravity. The velocity of the body at any time t is
View SolutionThe velocity of a body depends on time according to the equation v=t210+20. The body is undergoing
View SolutionThe velocity of a body of mass 2kg as a function of t is given by υ(t)=2tˆi+t2ˆj Find the momentum and force acting on it at time t=2s.
View SolutionThe velocity of a body of mass 2kg as a function of t is given by υ(t)=2tˆi+t2ˆj Find the momentum and force acting on it at time t=2s.
View SolutionThe displacement -time (x– t) graph of a body is given as
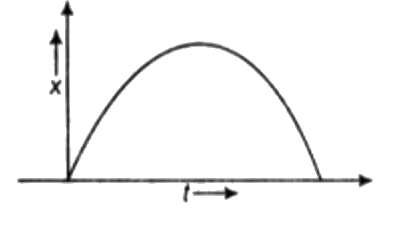
The corresponding velocity -time (v – t ) graph will be
View SolutionThe displacement-time (x - t) graph of a body is given as
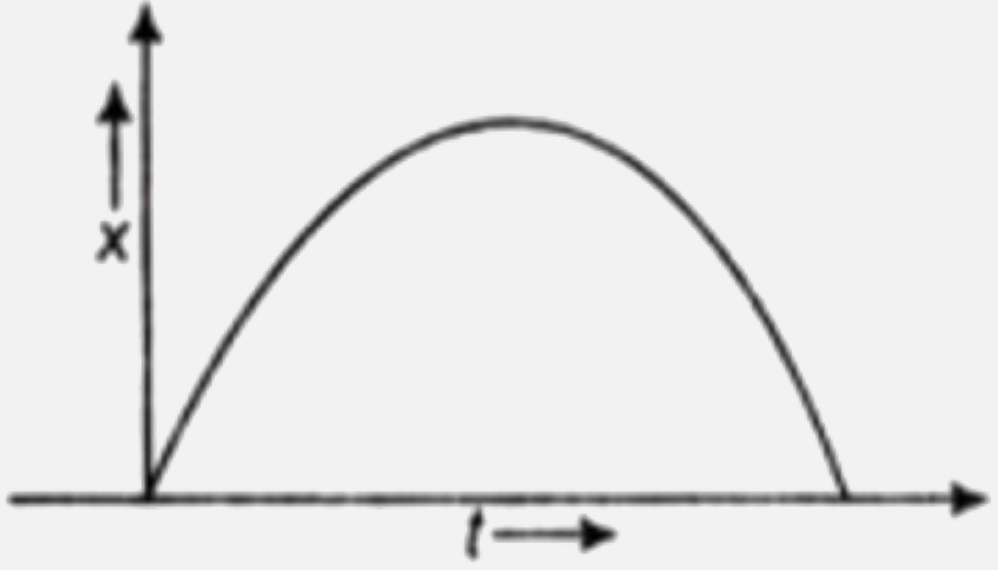
The corresponding velocity -time (v - t) graph will be
View SolutionThe angular momentum of a body is given by L=5t2+2t+1kgm2s.The torque acting on the body at t=1s is
View Solution
