In a quadrilateral PQRS,→PQ=→a,→QR=→b,→SP=→a−→b,M is the midpoint of →QRandX is a point on SM such that SX=45SM. Prove that P,XandR are collinear.Solution in Punjabi
PX=15PR
PX=35PR
PX=25PR
none of these
The correct Answer is:B
If we take point P as the origin, the position vectors of Q and S are a and b-a respectively.
In ΔPQR, we have
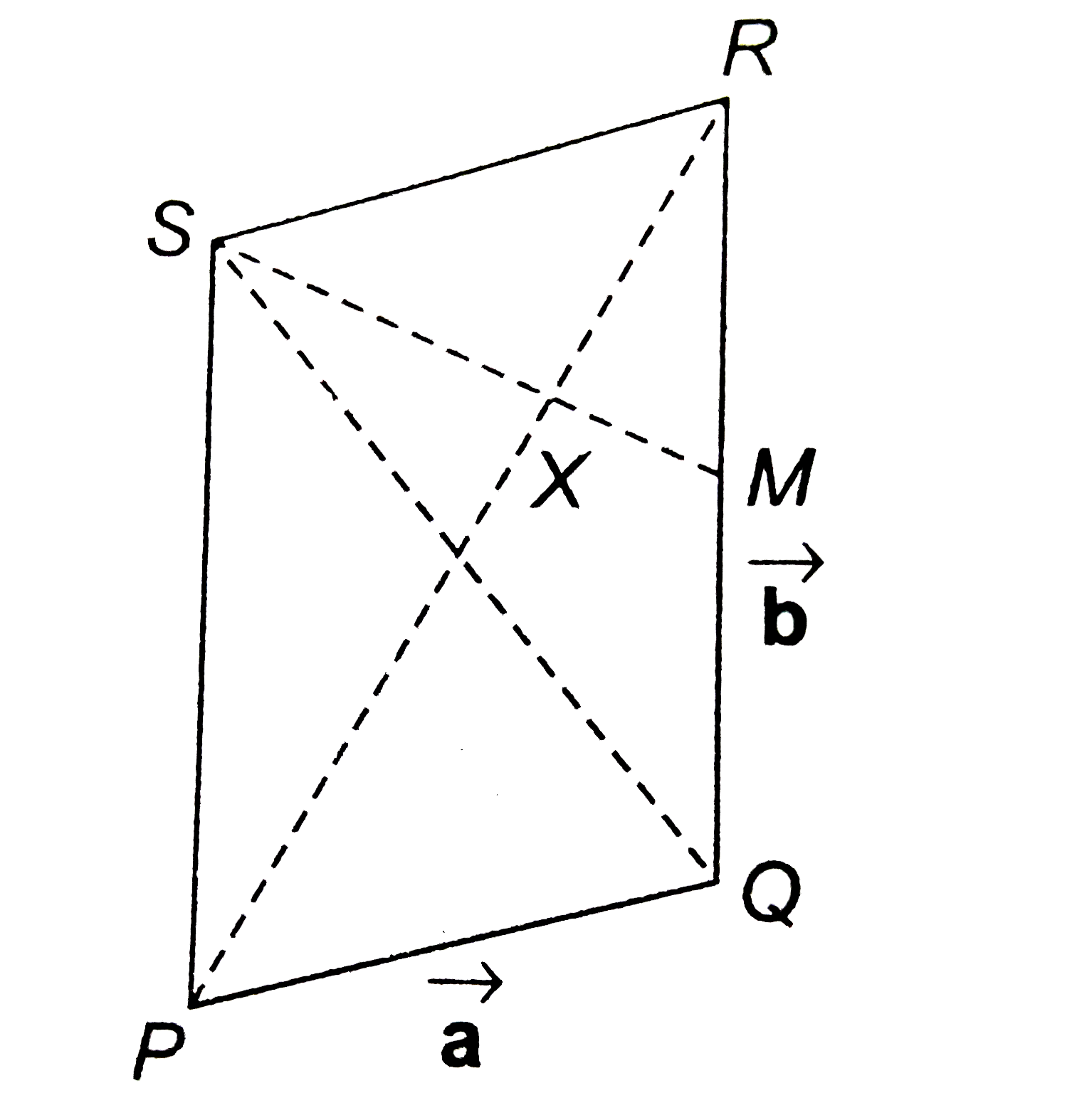
PR=PQ+QR⇒PR=a+b
∴Position vector of R=a+b
⇒PV or M=a+(a+b)2=(a+12b)
Now, SX=45SM
⇒XM=SM−SX=SM−45SM=15SM
∴SX:XM=4:1
⇒PV of X=4(a+12b)+1(b−a)4+1
=3a+2b5⇒PX=35(a+b)
⇒PX=35PR
Topper's Solved these Questions
- VECTOR ALGEBRABOOK - ARIHANT MATHSCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise For Session 1
7 Videos
- VECTOR ALGEBRABOOK - ARIHANT MATHSCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise For Session 2
17 Videos
- TRIGONOMETRIC FUNCTIONS AND IDENTITIESBOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
18 Videos
Similar Questions
Classify the following measure as scalar and vector: 10Kg
View SolutionIf a=2ˆi−ˆj+2ˆkandb=−ˆi+ˆj−ˆk, then find a+b. Also, find a unit vector along a+b.
View SolutionLet αandβ be non-zero real numbers such that 2(cosβ−cosα)+cosαcosβ=1. Then which of the following is/are true ?
View SolutionSuppose that vec p, vec q and vec r are three non-coplanar vectors in R^3. Let the components of a vector vec s along vec p, vec q and vec r be 4, 3 and 5, respectively. If the components of this vector vec s along (-vec p+vec q +vec r), (vec p-vec q+vec r) and (-vec p-vec q+vec r) are x, y and z, respectively, then the value of 2vec x+vec y+vec z is
View Solution→p,→q,and→r are three mutually perpendicular vectors of the same magnitude. If vector →x satisfies the equation →p×((→x−→q)×→p)+→q×((→x−→r)×→q)+→r×((→x−→p)×→r)=0,then→x is given by a.12(→p+→q−2→r) b. 12(→p+→q+→r) c. 13(→p+→q+→r) d. 13(2→p+→q−→r)
View SolutionThe projection of point P(→p) on the plane →r.→n=q is (→s) , then a. →s=(q−→p.→n)→n|→n|2 b. →s=p+(q−→p.→n)→n|→n|2 c. →s=p−(→p.→n)→n|→n|2 d. →s=p−(q−→p.→n)→n|→n|2
View SolutionIf vec p xxvec q = vec m xxvec n and vec p xxvec m = vec q xxvec n show that vec p-vec n is parallel to vec q-vec m where vec p! = Vec n and vec q! = Vec m
View SolutionIf vec p=3vec a-5vec b;vec q=2vec a+vec bvec r=vec a+4vec b ; vec s=-vec a+vec b are four vectors such that sin(vec p^^vec q)=1 and sin(vec r^^vec s)=1 then cos(vec a^^vec b) is
View SolutionIf vec p + vec q + vec r = xvec s and vec q + vec r + vec s = yvec p and are vec p, vec q, vec r non coplaner vectors then | vec p + vec q + vec r + vec r + vec s | =
View SolutionThree vectors vec(P) , vec(Q) and vec( R) are such that |vec(P)| , |vec(Q )|, |vec(R )| = sqrt(2) |vec(P)| and vec(P) + vec(Q) + vec(R ) = 0 . The angle between vec(P) and vec(Q) , vec(Q) and vec(R ) and vec(P) and vec(R ) are
View SolutionColumn- IColumn II(Operation of nonzero vectors→Pand→Q)(Possivle angle between→Pand→Q)(A)∣∣→P×→Q∣∣=0(P) 90∘(B)∣∣→P×→Q∣∣=√3→P.→Q(Q) 180∘(C)→P+→Q=→RandP+Q=R(R) 60∘(D)∣∣→P+→Q∣∣=∣∣→P−→Q∣∣(S) 0∘(T) 30∘
View SolutionSuppose that →p,→qand→r are three non-coplanar vectors in R. Let the components of a vector →s along →p,→qand→r be 4, 3 and 5 respectively. If the components of this vector →s along(−→p+→q+→r),(→p−→q+→r)and(−→p−→q+→r) are x,y and z respectively, then the value of 2x+y+z is
View SolutionThe projection of point P(→p) on the plane →r.→n=q is (→s) , then a. →s=(q−→p.→n)→n|→n|2 b. →s=p+(q−→p.→n)→n|→n|2 c. →s=p−(→p.→n)→n|→n|2 d. →s=p−(q−→p.→n)→n|→n|2
View SolutionIf P,Q and R are three collinear points such that →PQ=→a and →QR = →b. Find the vector →PR .
View SolutionIf vec P+ vec Q = vec R and |vec P| = |vec Q| = | vec R| , then angle between vec P and vec Q is
View SolutionIf →P+→Q=→Rand→P−→Q=→S, then R2+S2 is equal to
View Solution
