P is a point on the side BC of ΔABC and Q is a point such that PQ is the resultant of AP,PB and PC. Then, ABQC is aSolution in Punjabi
square
rectangle
parallelogram
trapezium
The correct Answer is:C
AP+PB+PC=PQ or AP+PB=PQ+CP
⇒AB=CQ
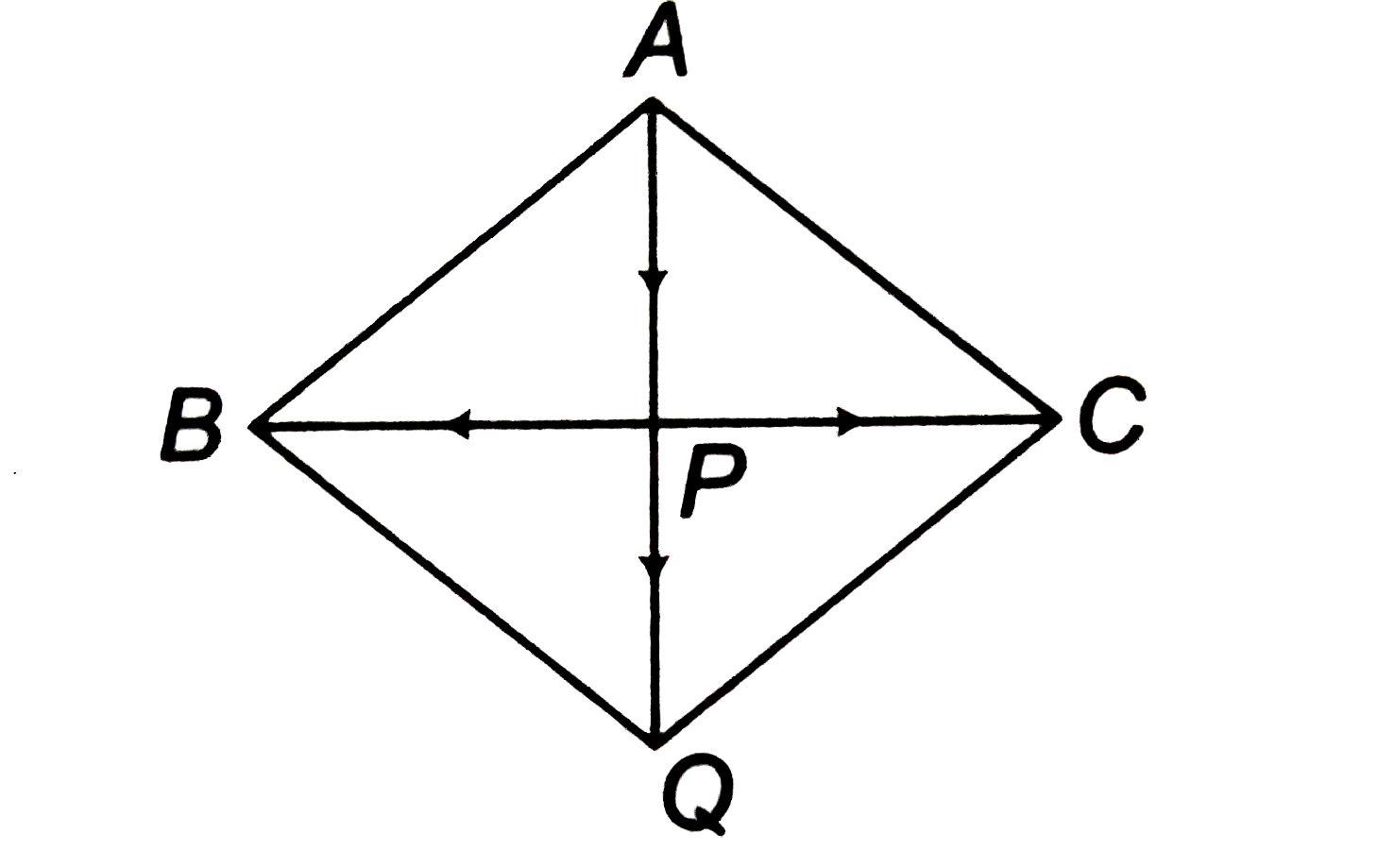
Hence, it is a parallelogram.
Topper's Solved these Questions
- VECTOR ALGEBRABOOK - ARIHANT MATHSCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise For Session 1
7 Videos
- VECTOR ALGEBRABOOK - ARIHANT MATHSCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise For Session 2
17 Videos
- TRIGONOMETRIC FUNCTIONS AND IDENTITIESBOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
18 Videos
Similar Questions
Classify the following measure as scalar and vector: 10Kg
View SolutionIf a=2ˆi−ˆj+2ˆkandb=−ˆi+ˆj−ˆk, then find a+b. Also, find a unit vector along a+b.
View SolutionLet αandβ be non-zero real numbers such that 2(cosβ−cosα)+cosαcosβ=1. Then which of the following is/are true ?
View SolutionIN any △ABC, a point p is on the side BC. If −−→PQis the resultant of the vectors −−→AP,−−→PB and −−→PC the prove that ABQC is a parallelogram and hence Q is a fixed point.
View SolutionΔABC में P और Q क्रमशः AB तथा AC पर दो बिन्दु इस प्रकार हो कि PQ∣∣BC और APPB=23 हो , तो AQQC का मान ज्ञात करें।
View SolutionP is a point on the side BC off the ΔABC and Q is a point such that PQ is the resultant of AP,PB and PC. Then, ABQC is a
View SolutionD is any point on the side BC of ΔABC. P and Q are centroids of ΔABD and ΔADC respectively. Prove that PQ∣∣BC.
View SolutionD is any point on the side BC of the ΔABC. P and Q are the centroids of ΔABD and ΔACD. Prove that PQ∣∣BC.
View SolutionABC का त्रिभुज है और P, BC पर कोई बिंदु है | यदि −−→AP,−−→PB,−−→PC का परिणामी −−→PQ हो तो दिखाइए ABQC एक समान्तर चतुर्भुज है और Q एक स्थिर बिंदु है |
View Solutionत्रिभुज ABC की भुजा BC में P कोई बिंदु है। यदि विषम −−→AP.−−→PB,−−→PC का परिणामी −−→PQ हो तो दर्शाइए कि ABQC एक समांतरचतुर्भुज है और इसलिए P की सभी स्थितियां के लिए Q एक स्थिर बिंदु है।
View SolutionIn ΔABC, P and Q are points on sides AB and AC respectively. For each of the following cases, state whether PQ∣∣BC or not :
AP=8cm,PB=8cm,AQ=7cm,AC=15cm
View SolutionABC is any triangle and P is a point on the side BC. If Q is a point such that ¯¯¯¯¯¯PQ=¯¯¯¯¯¯AP+¯¯¯¯¯¯PB+¯¯¯¯¯¯PC, then
View SolutionDeltaABC P is a point in the interior of X, Y and Z. The points on the sides PA, PB and PC respectively are such that XY||AB And XZ||AC Prove that YZ||BC
View SolutionDeltaABC P is a point in the interior of X, Y and Z are the points PQ, PB and PC respectively in such a way that XY||AB And XZ||AC | Prove that YZ||BC .
View SolutionProve by vector method that in any triangle ABC, the point P being on the side −−→BC, if −−→PQ is the resultant of the vectors −−→AP, −−→PB and −−→PC, then ABQC is a parallelogram.
View SolutionP is a point on the side BC off the ΔABC and Q is a point such that PQ is the resultant of AP,PB and PC. Then, ABQC is a
View Solution
