Question

If the normal at one end of the latus rectum of
the ellipse $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$
passes through one end of the monor axis, then prove that
eccentricity is constant.

Explanation

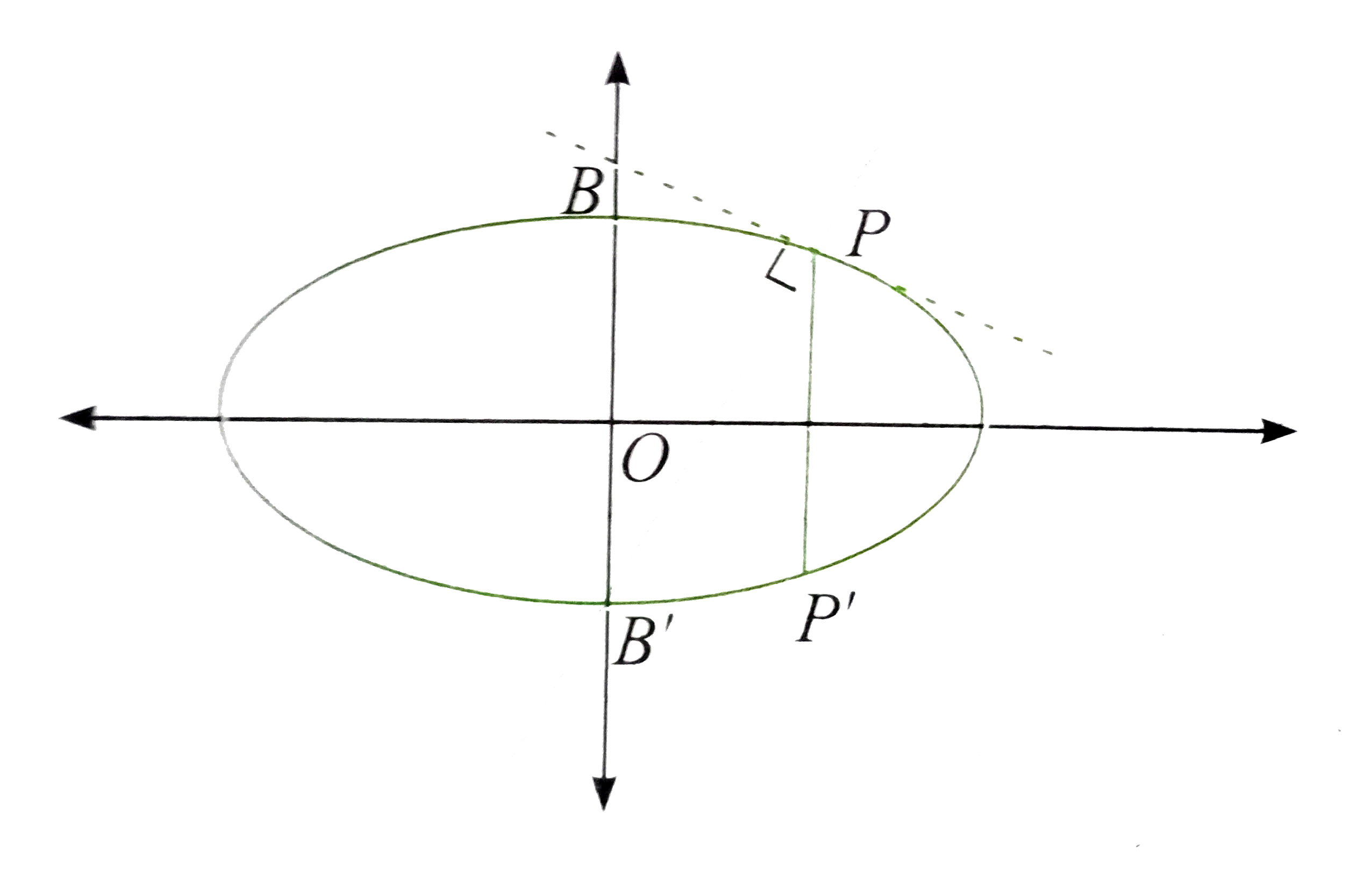
The equation of the normal at the extermity P of latus reactum is `x-ey=ae^(3)`
This passes through the extermity of the minor i.e.,B'(0,-b).
Then, `0+eb-ae^(3)=0`
`or ba^(2)" " or b^(2)=a^(2)e^(4)`
or `a^(2)(1-e^(2))=a^(2)e^(4)" " or e^(4)+e^(2)-1=0`