For a given point A(0,0), ABCD is a rhombus of side 10 units where slope of AB is and slope of AD is . The sum of abscissa and ordinate of point C (where C lies in first quadrant) is Solution in Bengali
The correct Answer is:
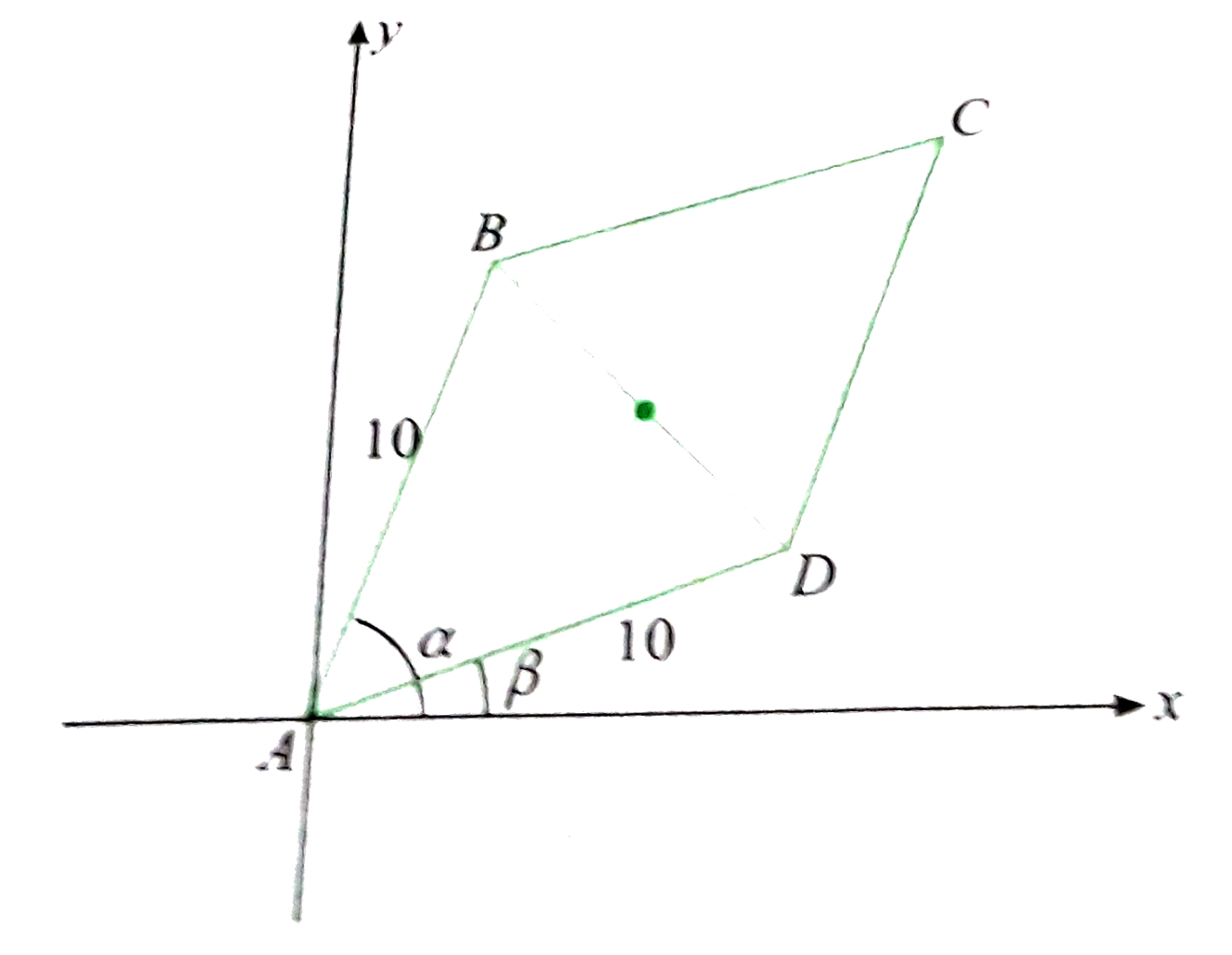
In the figure,
Coordinates of point B
Also,
coordinates of Point D
Diagonals of rhombus bisect. So, midpoint of BD is which is midpoint of AC. therefore, coordinates of C are .
Topper's Solved these Questions
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.5
5 Videos
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.6
9 Videos
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.3
10 Videos
- COORDINATE SYSTEMBOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type
2 Videos
- CROSS PRODUCTS BOOK - CENGAGE PUBLICATIONCHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.2
13 Videos
Similar Questions
Convert the polar coordinates to its equivalent Cartesian coordinates(2,π2).
View SolutionFind the locus of a point whose distance from (a, 0) is equal to its distance from the y-axis.
View SolutionIf point P(3,2) divides the line segment AB internally in the ratio of 3:2 and point Q(−2,3) divides AB externally in the ratio 4:3 then find the coordinates of points A and B.
View SolutionCoordinates of points on curve 5x2−6xy+5y2−4=0 which are nearest to origin are
View SolutionLet →a and →b be two vectors of equal magnitude 5 units. Let →p,→q be vectors such that →p=→a−→b and →q=→a+→b. If |→p×→q|=2{λ−(→a.→b)2}12, then value of λ is
View SolutionPoint A and B are in the first quadrant,point O is the origin. If the slope of OA is 1,slope of OB is 7 and OA=OB,Then slope of AB is: a. -1/5 b. -1/4 c. -1/3 d. -1/2
View SolutionThe slope of the tangent to the curve y=√4−x2 at the point where the ordinate and the abscissa are equal is −1 (b) 1 (c) 0 (d) none of these
View SolutionPoint A and B are in the first quadrant; point O is the origin. If the slope of OA is 1, the slope of OB is 7, and OA=OB, then the slope of AB is −15 (b) −14 (c) −13 (d) −12
View SolutionFor a given point A(0,0),ABCD is a rhombus of side 10 units where slope of AB is (4)/(3) and slope of AD is (3)/(4). The sum of abscissa and ordinate of point C (where C lies in first quadrant) is
View SolutionA straight line has slope (3)/(4) and it passes through the point A(-2,-5). Find the co- ordinates of a point B on this line where bar(AB)=10 unit.
View SolutionFind the equation of the curve passing through the point (0,0) and whose slope of the normal at a point (x, y) is equal to the sum of the ordinate and the product of the abscissa and ordinate of that point.
View SolutionThe slope of normal at any point P of a curve (lying in the first quadrant) is reciprocal of twice the product of the abscissa and the ordinate of point P. Then, the equation of the curve is (where, c is an arbitrary constant)
View SolutionThe slope of the tangent to the curve y=√4−x2 at the point where the ordinate and the abscissa are equal is (a)−1 (b) 1 (c) 0 (d) none of these
View SolutionFor a given point A(0,0), ABCD is a rhombus of side 10 units where slope of AB is 43and slope of AD is 34. The sum of abscissa and ordinate of point C (where C lies in first quadrant) is
View SolutionPoint A and B are in the first quadrant,point O is the origin. If the slope of OA is 1,slope of OB is 7 and OA=OB,Then slope of AB is: a. -1/5 b. -1/4 c. -1/3 d. -1/2
View SolutionThe slope of the tangent to the curve y=√4−x2 at the point where the ordinate and the abscissa are equal is (a) −1 (b) 1 (c) 0 (d) none of these
View Solution
