A line through point A(1,3) and parallel to the line x-y+1 = 0 meets the line 2x-3y + 9 = 0 at point P. Find distance AP without finding point P.Solution in Bengali
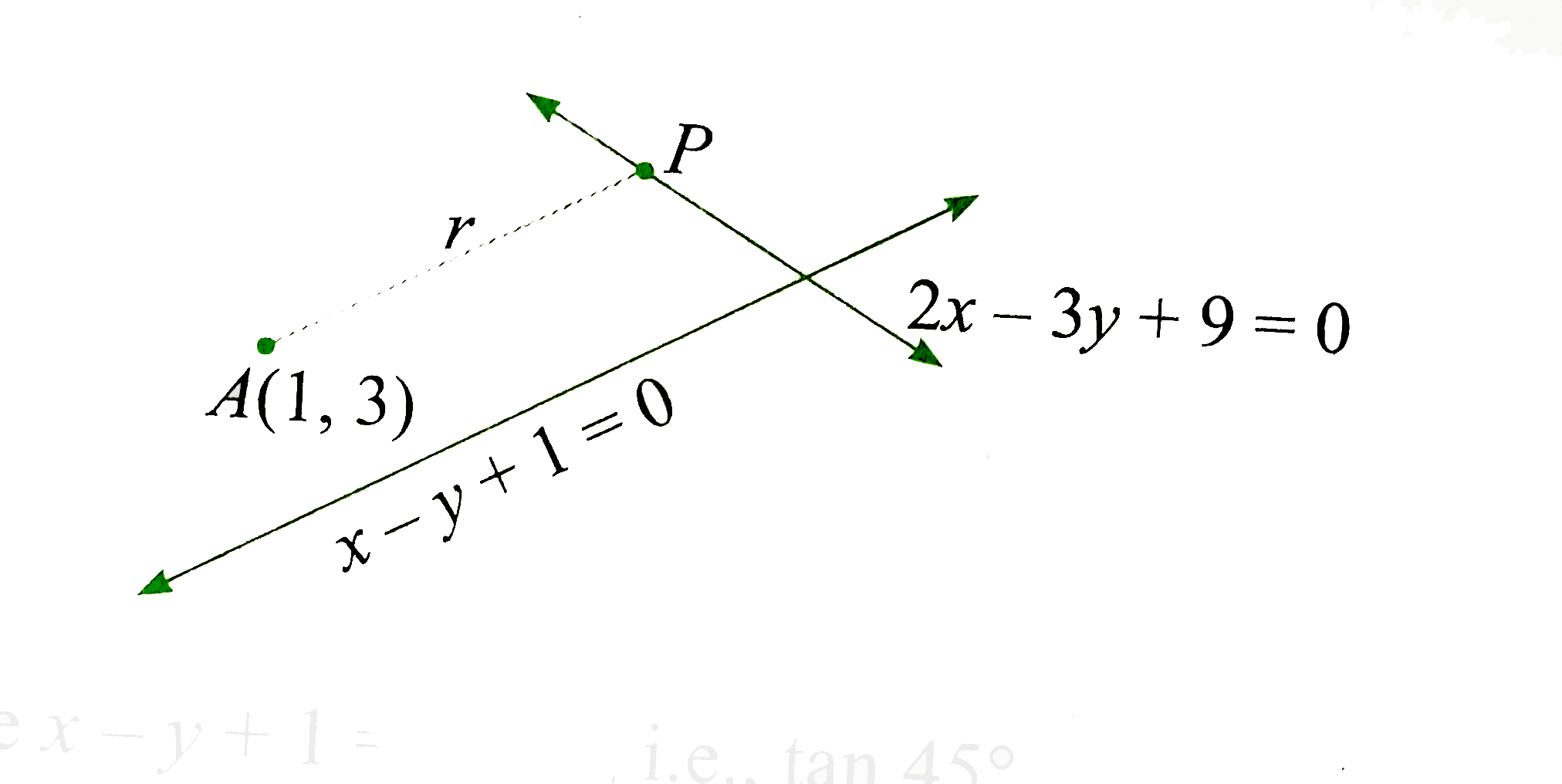
Slope of line x-y + 1 = 0 is 1, i.e., tan 45∘.
So, the equation of line through A(1,3) and having slope I is
x−1cos45∘=y−3sin45∘
or x−11/√2=y−31/√2r(say)
This line meets the given line 2x-3y + 9 = 0 at point P.
Let AP= r. Than P=(1+r√2,3+r√2).
But point P lies on the line 2x-3y + 9 = 0.
∴2(1+r√2)−3(3+r√2)+9=0
⇒r√2=2
⇒r=2√2
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXAMPLE
12 Videos
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - CONCEPT APPLICATION EXERCISE 2.1
23 Videos
- STRAIGHT LINEBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )
1 Videos
Similar Questions
Show that the lines 4x+y−9=0,x−2y+3=0,5x−y−6=0 make equal intercepts on any line of slope 2.
View SolutionFind the equation of the right bisector of the line segment joining the points (3,4) and (-1,2).
View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
View SolutionThe distance of a point (2,3) from the line 2x-3y+9=0 measured along a line x-y+1=0 is
View SolutionEquation of a line which passes through point A(2,3) and makes an angle of 45 with x axis.If this line meet the line x+y+1=0 at point P then distance AP is
View SolutionDistance of point (1,3) from the line 2x-3y+9=0 along x-y+1=0
View SolutionA line through point A(1,3) and parallel to the line x-y+1 = 0 meets the line 2x-3y + 9 = 0 at point P. Find distance AP without finding point P.
View SolutionFind the equation of the pair of straight lines passing through the point (1,3) and perpendicular to the lines 2x-3y+1=0 and 5x+y-3=0.
View SolutionDistance of point (1,3) from the line 2x−3y+9=0 along x−y+1=0
View SolutionA line through point A(1,3) and parallel to the line x-y+1 = 0 meets the line 2x-3y + 9 = 0 at point P. Find distance AP without finding point P.
View SolutionFind the distance of the point (2,3) from the line 2x−3y+9=0 measured along a line x−y+1=0.
View SolutionFind the equation of the line through the point of intersection of, the lines x−3y+1=0 and 2x+5y−9−0 and whose distance from the origin is √5
View SolutionWithout finding point of intersection obtain the equation of line passes from point of intersection of lines 5x+y+4=0and2x+3y−1=0. which is parallel to the line 4x−2y−1=0.
View SolutionFind the line passing through the point of intersection of the lines x−3y+1=0,2x+5y=9 and
(i) parallel to y−2x=0
(ii) perpendicular to 2x+y−5=0
View SolutionThe distance of the point (2,3) from the line 2x−3y+9=0 measured along a line x−y+1=0 is
View Solution
