What is the Euler’s formula for a Polyhedron?
The correct Answer is:B
Step-by-Step Solution:
1. Understanding Euler's Formula:
Euler's formula for polyhedra relates the number of faces (F), vertices (V), and edges (E) of a polyhedron. The formula is given by:
F+V−E=2
2. Identifying Components:
- Faces (F): These are the flat surfaces of the polyhedron.
- Vertices (V): These are the corners or points where the edges meet.
- Edges (E): These are the line segments where two faces meet.
3. Example with a Cube:
Let's take a cube as an example to illustrate Euler's formula.
- A cube has:
- Faces (F): 6 (top, bottom, left, right, front, back)
- Vertices (V): 8 (corners of the cube)
- Edges (E): 12 (the line segments connecting the vertices)
4. Substituting Values into the Formula:
Now, we will substitute the values of F, V, and E into Euler's formula:
F+V−E=2
Substituting the values:
6+8−12
5. Calculating the Left Side:
- First, add the number of faces and vertices:
6+8=14
- Then, subtract the number of edges:
14−12=2
6. Conclusion:
Since the left side equals 2, we can confirm that Euler's formula holds true for a cube:
F+V−E=2(True)
Euler's formula for polyhedra relates the number of faces (F), vertices (V), and edges (E) of a polyhedron. The formula is given by:
F+V−E=2
- Faces (F): These are the flat surfaces of the polyhedron.
- Vertices (V): These are the corners or points where the edges meet.
- Edges (E): These are the line segments where two faces meet.
Let's take a cube as an example to illustrate Euler's formula.
- A cube has:
- Faces (F): 6 (top, bottom, left, right, front, back)
- Vertices (V): 8 (corners of the cube)
- Edges (E): 12 (the line segments connecting the vertices)
Now, we will substitute the values of F, V, and E into Euler's formula:
F+V−E=2
Substituting the values:
6+8−12
- First, add the number of faces and vertices:
6+8=14
- Then, subtract the number of edges:
14−12=2
Since the left side equals 2, we can confirm that Euler's formula holds true for a cube:
F+V−E=2(True)
Similar Questions
What is the formula of diborane ?
View SolutionWhat is the empirical formula for a compound that contains 22%S and 78%F? (S=32,F=19)
View SolutionWhat is the formula of hypo?
View SolutionWhat is a molecular formula?
View SolutionWhat is a chemical formula?
View Solution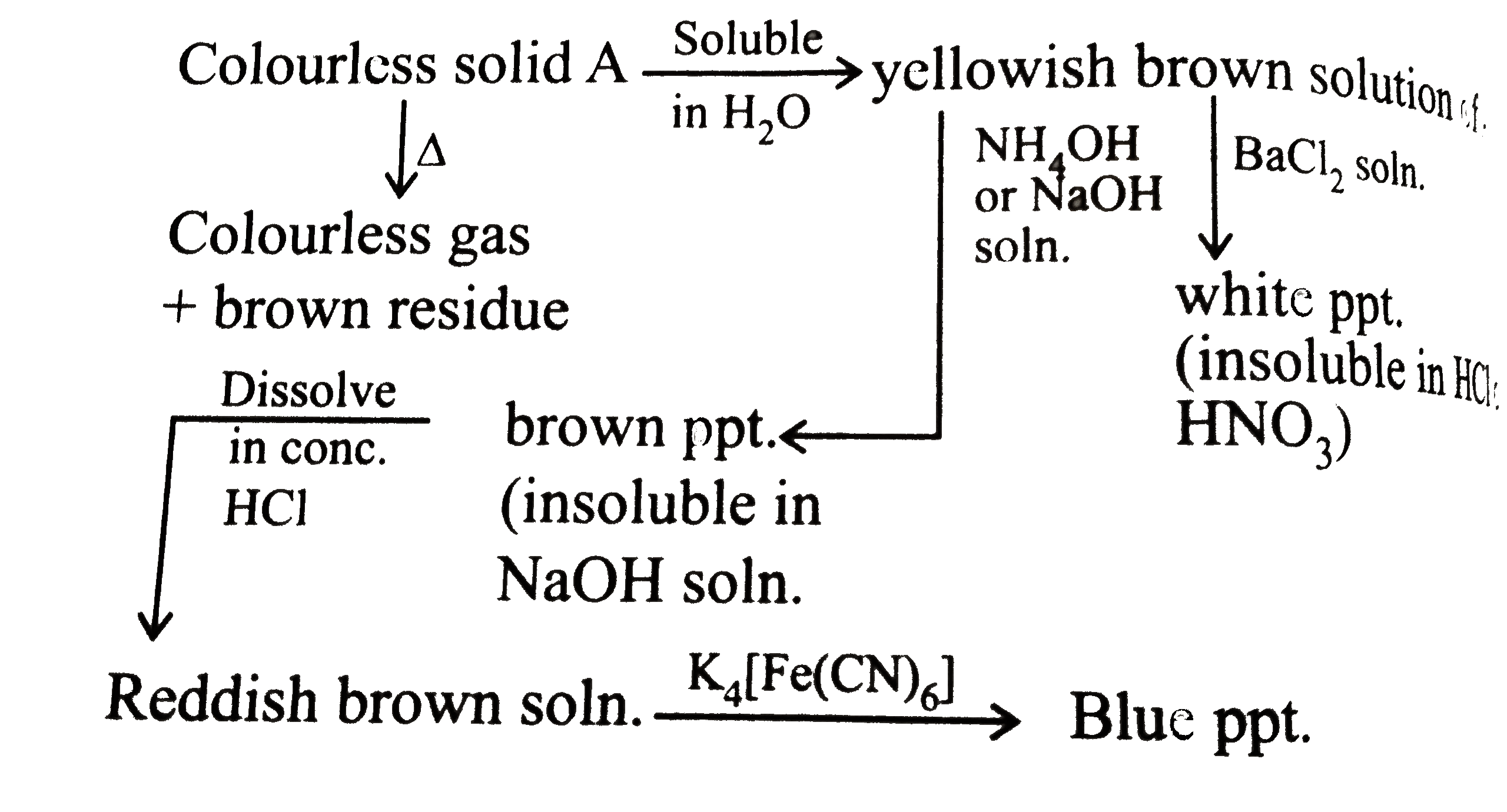
What is the formula of brown ppt?
View SolutionWhat is the formula of glycerol?
View SolutionWhat is the general formula of an amino acid?
View SolutionWhen 3.2 g S is vaporized at 450∘Cand723 mm pressure, the vapours occupy a volume of 780 mL What is the molecular formula of S vapours ?
View SolutionWhat is the general formula of silicones?
View Solution
