Suppose that →p,→qand→r are three non- coplaner in R3 ,Let the components of a vector→s along →p,→qand→r be 4,3, and 5, respectively , if the components this vector →s along (−→p+→q+→r),(→p−→q+→r)and(−→p−→q+→r) are x, y and z , respectively , then the value of 2x+y+z is Solution in Tamil
The correct Answer is:(9)
Here s=4P+3q+5r
and s=(−P+q+r)x+(p−q+r)y+(−P−q+r)z……(1)
∴4p+3q+5r=p(−x+y−z)+q(x−y+z)+r(x+y+z)
On comparing both sides we get
−x+y−z=4,x−y=3 and x+y+z=6
On solving above equations we get
x=4,y=92,=−72
∴2x+y+z=8+92−72=9
Similar Questions
If →p,→q and →r are perpendicular to →q+→r,→r+→p and →p+→q respectively and if |→p+→q|=6,|→q+→r|=4√3 and |→r+→p|=4 then |→p+→q+→r| is
View Solution→P,→Q,→R,→S are vector of equal magnitude. If →P+→Q−→R=0 angle between →P and →Q is θ1 . If →P+→Q−→S=0 angle between →P and →S is θ2 . The ratio of θ1 to θ2 is
View SolutionSuppose that →p,→qand→r are three non- coplaner in R3 ,Let the components of a vector→s along →p,→qand→r be 4,3, and 5, respectively , if the components this vector →s along (−→p+→q+→r),(→p−→q+→r)and(−→p−→q+→r) are x, y and z , respectively , then the value of 2x+y+z is
View SolutionIf →P+→Q=→Rand P=Q=R√2, then find the angles between the pairs of vectors (→P+→Q),(→Q,→R)and(→R,→P)respectively
View SolutionSuppose that vec p,vecqand vecr are three non- coplaner in R^(3) ,Let the components of a vector vecs along vecp , vec q and vecr be 4,3, and 5, respectively , if the components this vector vec s along (-vecp+vec q +vecr),(vecp-vecq+vecr) and (-vecp-vecq+vecr) are x, y and z , respectively , then the value of 2x+y+z is
View SolutionThree vectors →P,→Q and →R are shown in the figure. Let S be any point on the vector →R. The distance between the points P and S is b∣∣→R∣∣. The general relation among vectors→P,→Q and →S is:
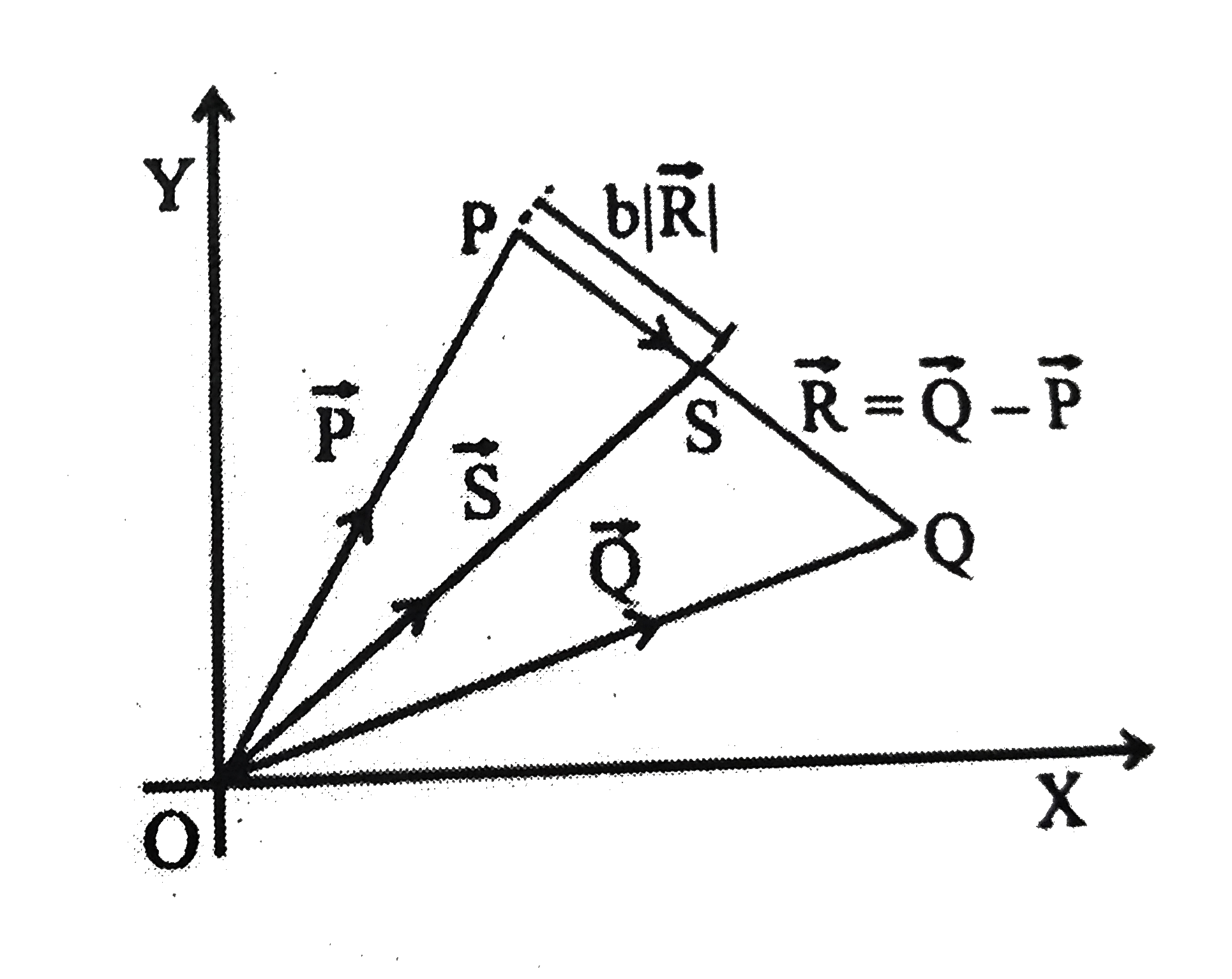
View SolutionSuppose that vec p,vecqand vecr are three non- coplaner in R^(3) ,Let the components of a vector vecs along vecp , vec q and vecr be 4,3, and 5, respectively , if the components this vector vec s along (-vecp+vec q +vecr),(vecp-vecq+vecr) and (-vecp-vecq+vecr) are x, y and z , respectively , then the value of 2x+y+z is
View SolutionThree vectors →P,→Q and →R are shown in the figure. Let S be any point on the vector →R. The distance between the points P and S is b∣∣→R∣∣. The general relation among vectors→P,→Q and →S is:

View Solution
