Question

Find the area bounded by the curve $x^2=4y$ and the line $x=4y-2$.

Options

A. `(7)/(8)`
B. `(9)/(8)`
C. `(5)/(4)`
D. `(3)/(4)`

Answer

B

Explanation

Given equation of curve is `x^(2)=4y,` which represent a parabola with vertex (0, 0) and it open upward.

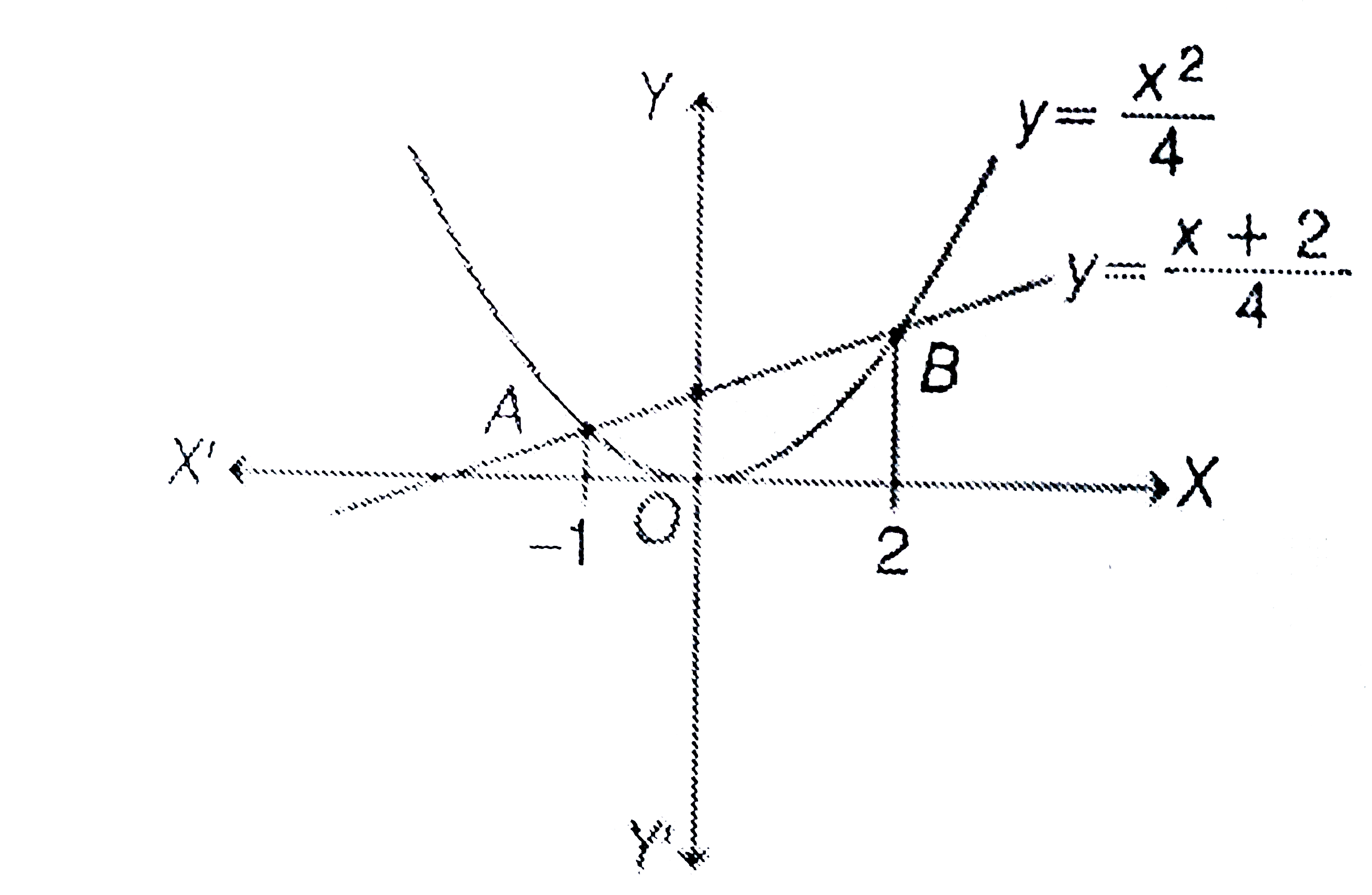
Now, let us find the points of intersection of `x^(2)=4y` and `4y=x+2`.
For this consider, `x^(2) =x+2`
`rArr x^(2)-x-2=0`
`rArr (x-2)(x+1)=0`
`rArr x= -1," then " y=(1)/(4)`
and when `x=2, " then " y=1`
Thus, the points of intersection are `A(-1,(1)/(4)) and B(2,1).`
Now, required area = area of shaded region
`=int_(-1)^(2){y("line")-y("parabola")}dx`
`=int_(-1)^(2)((x+2)/(4)-(x^(2))/(4))dx=(1)/(4)[(x^(2))/(2)+2x-(x^(3))/(3)]_(-1)^(2)`
`=(1)/(4)[(2+4-(8)/(3))-((1)/(2)-2+(1)/(3))]`
`=(1)/(4)[8-(1)/(2)-3]=(1)/(4)[5-(1)/(2)]=(9)/(8)` sq units.