Question

The area (in sq. units) of the region bounded by the parabola $y=x^2+2\text{and the lines}y=x+1,x=0\text{and}x=3$, is

Options

A. `(15)/(2)`
B. `(17)/(4)`
C. `(21)/(2)`
D. `(15)/(4)`

Answer

A

Explanation

Given equation of parabola is ` y= x^(2)+2, ` and the line is ` y=x+1 `

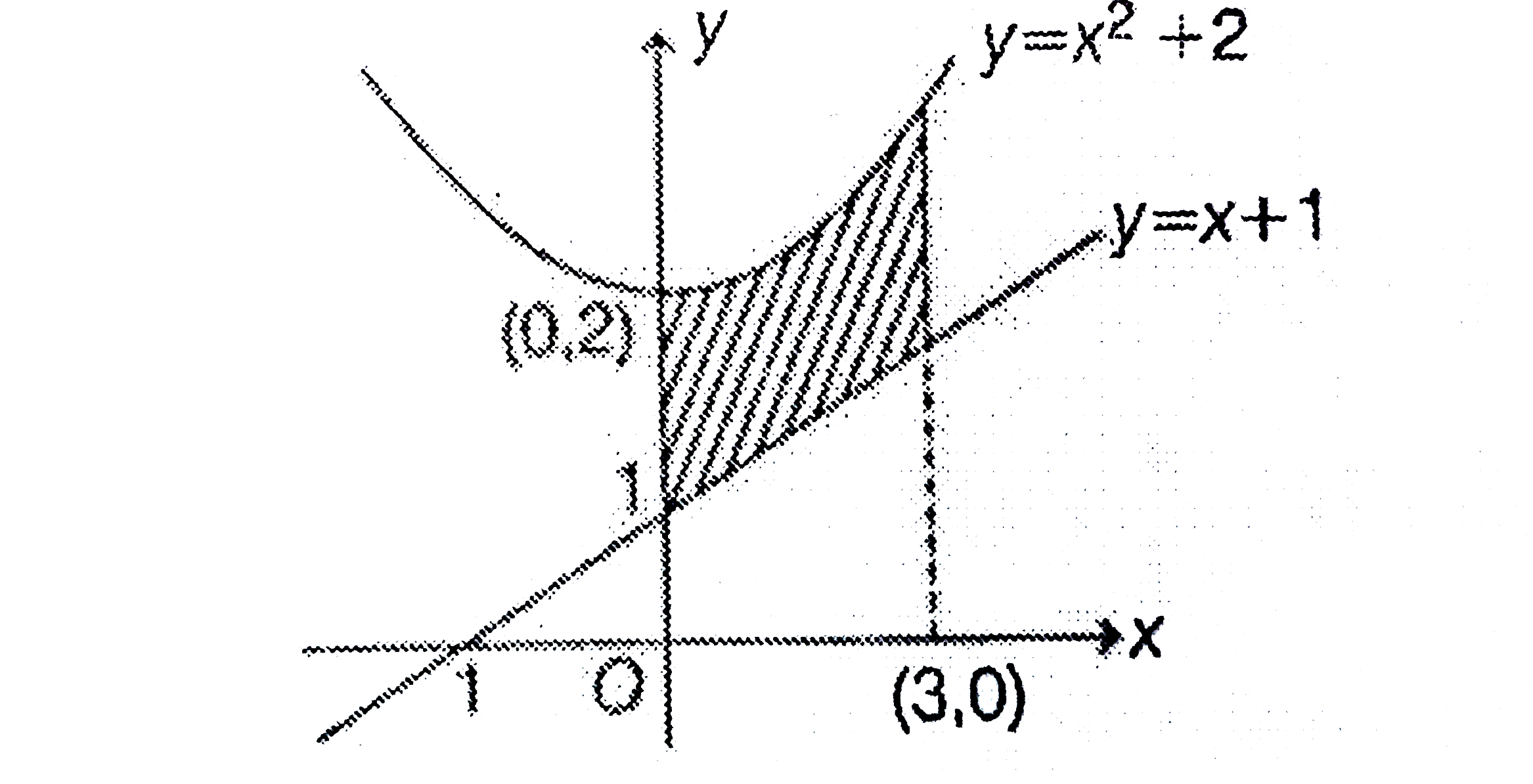
The required area = area of shaded region
`= int_(0)^(3)((x^(2)+2)-(x+1))dx = int_(0)^(3)(x^(2)-x+1)dx `
`=[(x^(3))/(3)-(x^(2))/(2)+x]_(0)^(3) =((27)/(3)-(9)/(2)+3)-0`
`=9-(9)/(2)+3=12-(9)/(2)=(15)/(2)` sq units