Twenty metres of wire is available for fencing off a flower-bed in the form of a circular sector. Then the maximum area (in sq.m) of the flower-bed is: (a) 25 (2) 30 (3) 12.5 (4) 10Solution in Tamil
12.5
10
25
30
The correct Answer is:C
Total length =2r+rθ=20
⇒θ=20−2rr
Now, are of flower-bed,
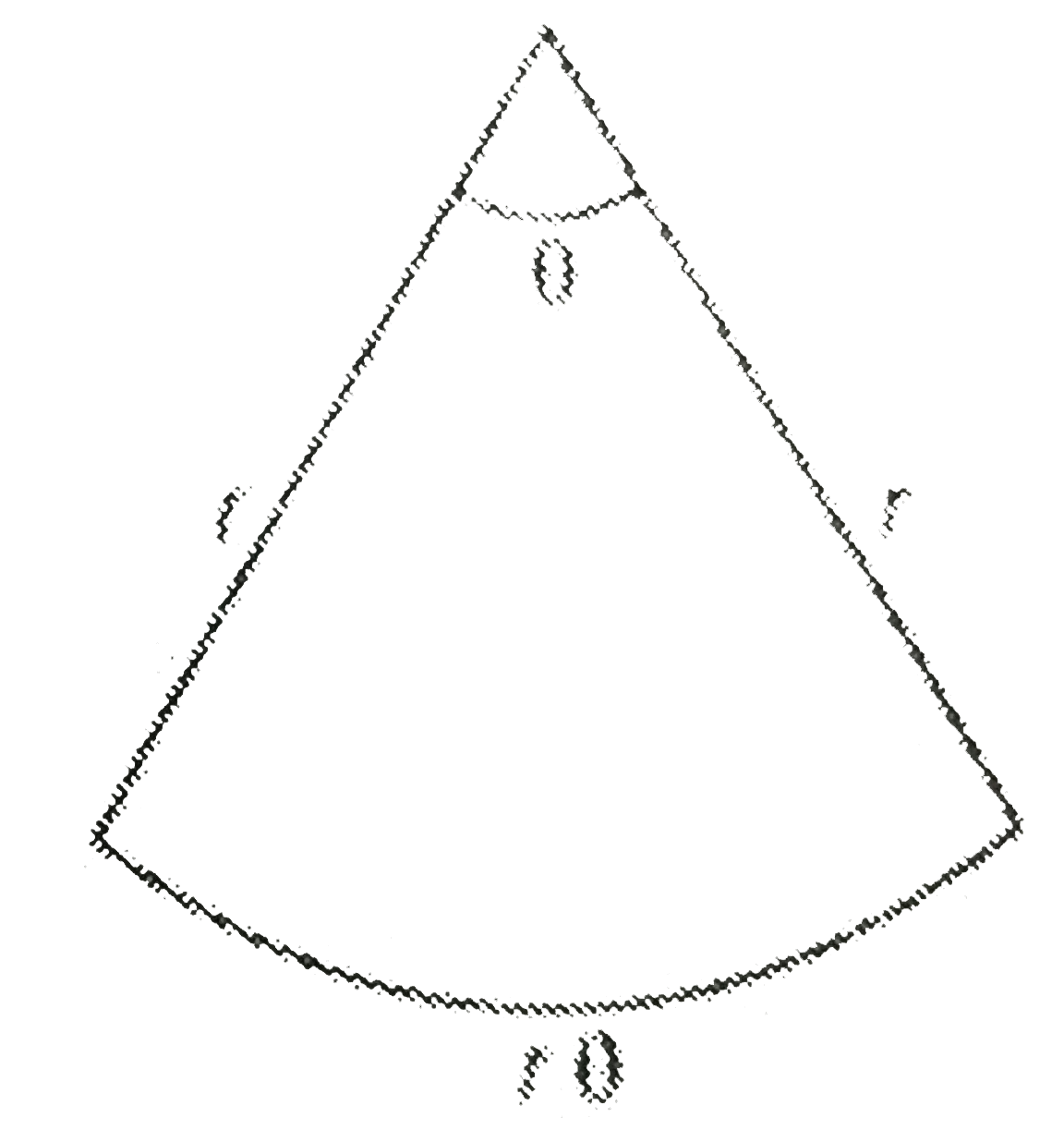
A=12r2θ
⇒A12r2(20−2rr)
⇒A=10r−r2
∴dAdr=10−2r
For maximum or minima, put, dAdr=0
⇒10−2r=0⇒r=5
∴Amax=12(5)2[20−2(5)5]
=12×25×2=25 sq. m
Similar Questions
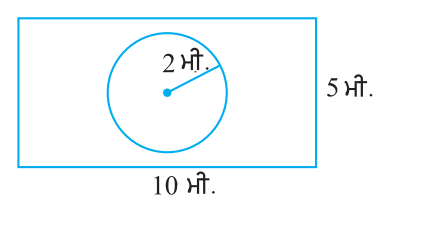
ਜਿਵੇਂ ਚਿਤਰ ਵਿਚ ਵਿਖਾਇਆ ਗਿਆ ਹੈ, ਇੱਕ ਆਇਤਕਾਰ ਪਾਰਕ ਦੇ ਕੇਂਦਰ ਵਿੱਚ ਇਕ ਚੱਕਰਾਕਾਰ ਫੁੱਲਾਂ ਦੀ ਕਿਆਰੀ ਹੈ। ਪਤਾ ਕਰੋ:- ਫੁੱਲਾਂ ਦੀ ਕਿਆਰੀ ਨੂੰ ਛੱਡ ਕੇ, ਪਾਰਕ ਦੇ ਬਾਕੀ ਭਾਗ ਦਾ ਖੇਤਰਫਲ
 02:39View Solution
02:39View Solutionएक फूलों की क्यारी, जो एक वृत्त के त्रिज्य खंड के रूप में है, की घेराबंदी करने के लिये बीस मीटर तार उपलब्ध है। तो फूलों की क्यारी का अधिकतम क्षेत्रफल ( वर्ग मी. में ) है
05:25View SolutionTwenty metres of wire is available for fencing off a flower-bed in the form of a circular sector. Then the maximum area (in sq.m) of the flower-bed is: 25 (2) 30 (3) 12.5 (4) 10
04:05View SolutionA wire of length 20m is available to fence off a flower bed in the form of a sector of a circle. What must be the radius of the circle, if we wish to have a flower bed with the greatest possible area?
06:16View SolutionTwenty metres are available to fence a flower bed in the form of a circular sector. If the flower bed should have the greatest possible surface area, the radius of the circle must be
03:38View SolutionTwenty two meters are available to fence a flower bed in the form of a circular sector. If the flower bed should have the greatest possible surface area, the radius of the circle must be:
04:22View Solutionसंलग्न आकृति एक आयताकार पार्क के मध्य में एक वृत्ताकार फूलों की क्यारी को दर्शाती है। ज्ञात कीजिए :
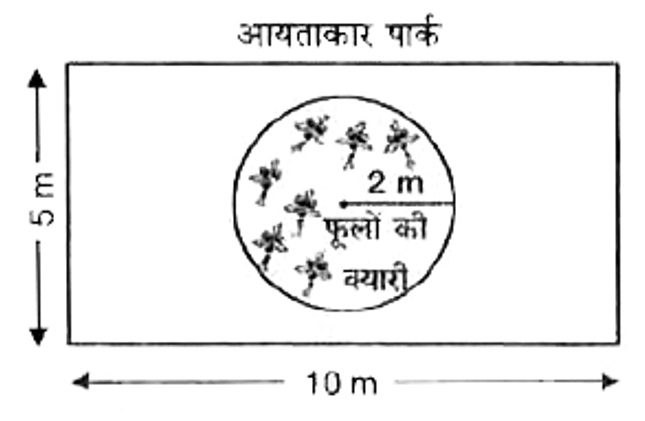
फूलों की क्यारी का क्षेत्रफल02:17View SolutionTwenty metres of wire is available for fencing off a flower-bed in the form of a circular sector. Then the maximum area (in sq.m) of the flower-bed is: (a) 25 (2) 30 (3) 12.5 (4) 10
05:09View Solution
