Let T be the line passing through the points P(−2, 7) and Q(2, −5) . Let F1 be the set of all pairs of circles (S1, S2) such that T is tangent to S1 at P and tangent to S2 at Q , and also such that S1 and S2 touch each other at a point, say, M . Let E1 be the set representing the locus of M as the pair (S1, S2) varies in F1 . Let the set of all straight lines segments joining a pair of distinct points of E1 and passing through the point R(1, 1) be F2 . Let E2 be the set of the mid-points of the line segments in the set F2 . Then, which of the following statement(s) is (are) TRUE? The point (−2, 7) lies in E1 (b) The point (45,75) does NOT lie in E2 (c) The point (12, 1) lies in E2 (d) The point (0,32) does NOT lie in E1Solution in Tamil
The point (−2,7) lies in E1
The point (4/5,7/5) does NOT lie in E2
The point (1/2,1) lie in E2
The point (0,3/2) does NOT lie in E1
The correct Answer is:2,4
According to the question , we have the following figure :
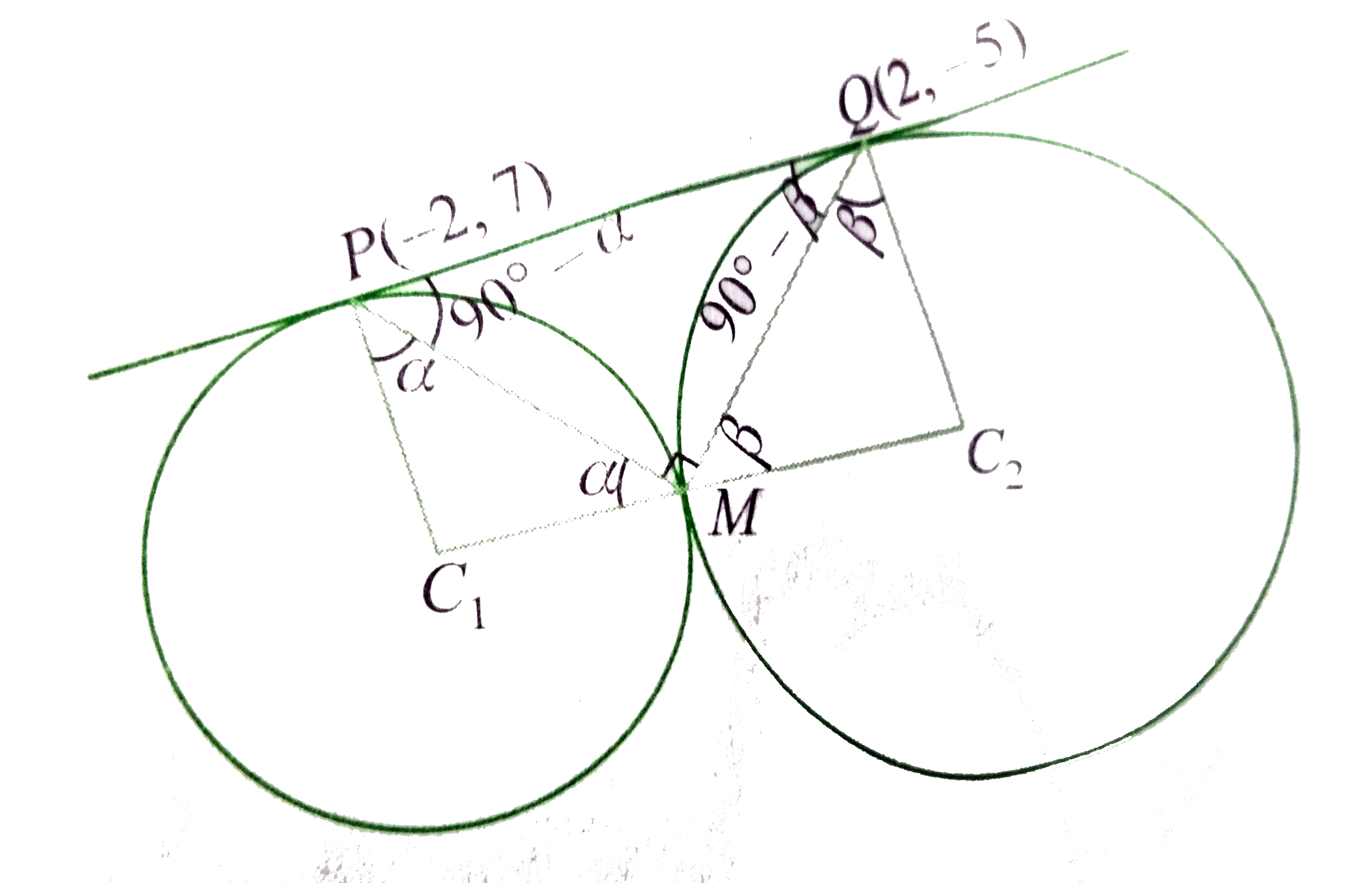
Clearly, ∠PMQ=π2.
Hence, locus of M is
(x+2)(x−2)+(y−7)(y+5)=0
or E1≡x2+y2−2y−39=0 (i)
Clearly, E2 is the locus of mid-points of chords of E1 passing through (1,1).
Let the mid-pioint of chord of E1 be (h,k) .
Its equation is
h+k−(1+k)−39=h2+k2−2k−39
or h2+k2−2k−h+1=0
Hence, E2≡x2+y2−x−2y+1=0 (ii) ltbrlt Operation (A) is incorrect, although, it satisfies eq. (i) otherwise the line T would touch the second circle at two points.
Als, (4/5,7/5) satisfies eq. (ii) but again in this case one end of the chord would be (−2,7) which is not included in E1.Therefore, (4/5,7/5) does not lie in E2
(12,1) does not satisfy eq. (ii), therefore, it does not lie in E2.
(0,3/2) does not satisfy eq. (i), so it does not lie inE1.
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - MATRIX MATCH TYPE6videos
CIRCLE
- BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - JEE Main Previous Year10videos
CIRCLE
- BOOK - CENGAGECHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension11videos
BINOMIAL THEOREM
- BOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank16videos
CIRCLES
Similar Questions
Find the derivative of y=ln(cosx2).
09:58View SolutionIf P and Q are the points of intersection of the circles x2+y2+3x+7y+2p=0 and x2+y2+2x+2y−p2=0 then there is a circle passing through P,Q and (1,1) for
03:23View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is01:51View SolutionIf ax+by=10 is the chord of minimum length of the circle (x−10)2+(y−20)2=729 and the chord passes through (5,15) then the value of (4a+2b) is
03:13View SolutionLet T be the line passing through the points P(−2,7) and Q(2,−5) . Let F1 be the set of all pairs of circles (S1,S2) such that T is tangent to S1 at P and tangent to S2 at Q , and also such that S1 and S2 touch each other at a point, say, M . Let E1 be the set representing the locus of M as the pair (S1,S2) varies in F1 . Let the set of all straight lines segments joining a pair of distinct points of E1 and passing through the point R(1,1) be F2 . Let E2 be the set of the mid-points of the line segments in the set F2 . Then, which of the following statement(s) is (are) TRUE? The point (−2,7) lies in E1 (b) The point (45,75) does NOT lie in E2 (c) The point (12,1) lies in E2 (d) The point (0,32) does NOT lie in E1
03:38View SolutionAmong two interfering sources, let S1 be ahead of the phase by 90∘ relative to S2 . If an observation point P is such that PS1−PS2=1.5λ , the phase difference between the waves from S1andS2 reaching P is
04:25View SolutionLet Sn=cos(nπ10),n=1,2,3,…… then the value of s1s2……s10s1+s2+……..+s10 is equal to
02:49View SolutionLet the sum of n, 2n, 3n terms of an A.P. be S1,S2andS3 , respectively, show that S3=3(S2−S1)
05:43View SolutionLet the sum of n, 2n, 3n terms of an A.P. be S1,S2andS3 , respectively, show that S3=3(S2−S1)
13:51View SolutionLet the sum of n, 2n, 3n terms of an A.P. be S1,S2andS3 , respectively, show that S3=3(S2−S1)
05:05View SolutionLet the sum of n, 2n, 3n terms of an A.P. be S1,S2and S3, respectively, show that S3=3(S2−S1).
04:55View SolutionIf S1,S2andS3 be respectively the sum of n, 2n and 3n terms of a G.P., prove that S1(S2+S3)=(S1)2+(S2)2
02:58View SolutionLet T be the line passing through the points P(−2, 7) and Q(2, −5) . Let F1 be the set of all pairs of circles (S1, S2) such that T is tangent to S1 at P and tangent to S2 at Q , and also such that S1 and S2 touch each other at a point, say, M . Let E1 be the set representing the locus of M as the pair (S1, S2) varies in F1 . Let the set of all straight lines segments joining a pair of distinct points of E1 and passing through the point R(1, 1) be F2 . Let E2 be the set of the mid-points of the line segments in the set F2 . Then, which of the following statement(s) is (are) TRUE? The point (−2, 7) lies in E1 (b) The point (45,75) does NOT lie in E2 (c) The point (12, 1) lies in E2 (d) The point (0,32) does NOT lie in E1
07:31View SolutionIf S1,S2,S3 be respectively the sums of n,2n,3n terms of a G.P., then prove that (S1)2+(S2)2=S1(S2+S3) .
02:37View SolutionLet the sum of n, 2n, 3n terms of an A.P. be S1, S2 and S3, respectively, show that S3=3(S2−S1)
03:22View Solution
