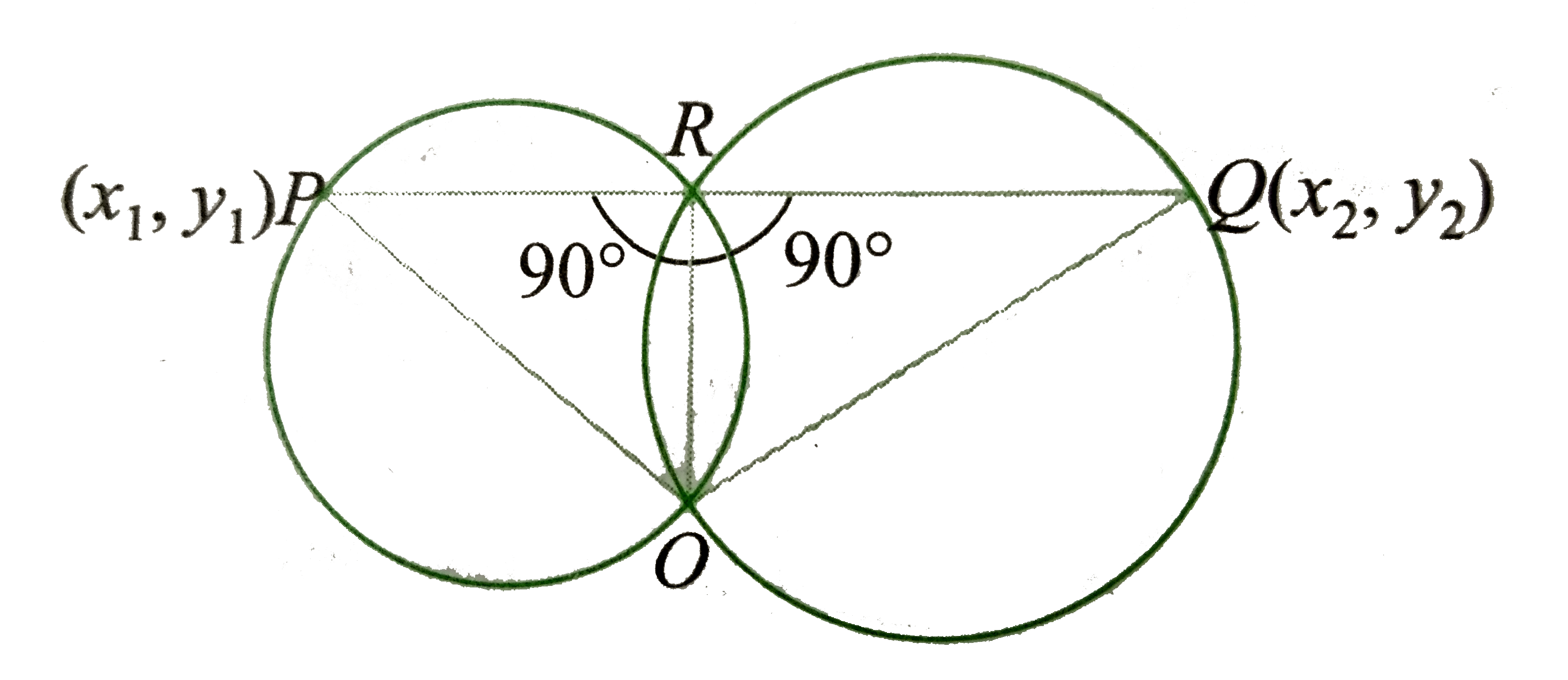
The coordinates of two points PandQ are (x1,y1)and(x2,y2)andO is the origin. If the circles are described on OPandOQ as diameters, then the length of their common chord is (a)|x1y2+x2y1|PQ (b) |x1y2−x2y1|PQ |x1x2+y1y2|PQ (d) |x1x2−y1y2|PQSolution in Tamil
|x1y2+x2y1|PQ
|x1y2−x2y1|PQ
|x1x2−y2y1|PQ
|x1x2+y2y1|PQ
The correct Answer is:2
OR=2×area of ΔOPQPQ
=2×∣∣12(x1y2−x2y1)∣∣PQ=|x1y2−x2y1|PQ
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Multiple)25videos
CIRCLE
- BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Comprehension)46videos
CIRCLE
- BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.65videos
CIRCLE
- BOOK - CENGAGECHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension11videos
BINOMIAL THEOREM
- BOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank16videos
CIRCLES
Similar Questions
about to only mathematics
03:06View SolutionEach side of a square is of length 6 units and the centre of the square Is ( - 1, 2). One of its diagonals is parallel to x+y=0. Find the co-ordinates of the vertices of the square.
02:27View SolutionIf the circle x2+y2+2x+3y+1=0 cuts x2+y2+4x+3y+2=0 at AandB , then find the equation of the circle on AB as diameter.
05:02View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is01:47View SolutionIf ax+by=10 is the chord of minimum length of the circle (x−10)2+(y−20)2=729 and the chord passes through (5,15) then the value of (4a+2b) is
09:11View SolutionThe coordinates of two points PandQ are (x1,y1)and(x2,y2)andO is the origin. If the circles are described on OPandOQ as diameters, then the length of their common chord is |x1y2+x2y1|PQ (b) |x1y2−x2y1|PQ |x1x2+y1y2|PQ (d) |x1x2−y1y2|PQ
04:08View SolutionIf the line segment joining the points P(x1,y1)and Q(x2,y2) subtends an angle α at the origin O, prove that : OP.OQ cosα=x1x2+y1y2.
04:04View SolutionIf θ is the angle which the straight line joining the points (x1,y1)and (x2,y2) subtends at the origin, prove that tanθ=x2y1−x1y2x1x2+y1y2 andcosθ=x1x2+y1y2√x12+y12x22+y22
04:24View SolutionIf 'P$Q', means 'P is father of Q ','P#Q' means 'P is mother of Q', 'P**Q' means 'P is sister of Q ', then how is D related to N in N#A$B*D ?
02:06View SolutionIf the pthandqth terms of a G.P. are qandp respectively, show that (p+q)th term is (qppq)1p−q .
03:05View SolutionIf pandq are positive, then prove that the coefficients of xpandxq in the expansion of (1+x)p+q will be equal.
04:00View SolutionIf O is the origin and if the coordinates of any two points Q1 and Q2 are (x1,y1) and (x2,y2), respectively, prove that OQ1.OQ2cos∠Q1OQ2=x1x2+y1y2.
02:33View SolutionThe coordinates of two points PandQ are (x1,y1)and(x2,y2)andO is the origin. If the circles are described on OPandOQ as diameters, then the length of their common chord is (a)|x1y2+x2y1|PQ (b) |x1y2−x2y1|PQ |x1x2+y1y2|PQ (d) |x1x2−y1y2|PQ
07:23View SolutionIn Figure, AP and BQ are perpendiculars to the line segment AB and AP=BQ . Prove that O is the mid-point of line segment AB and PQ
05:54View SolutionPQ and RS are two parallel chords of a circle and lines RP and SQ intersect each other at O as shown in Figure. Prove that OP=OQ
03:17View SolutionIf the line segment joining the points P(x1,y1)and Q(x2,y2) subtends an angle α at the origin O, prove that : OP.OQ cosα=x1x2+y1y2.
03:06View Solution
