Question

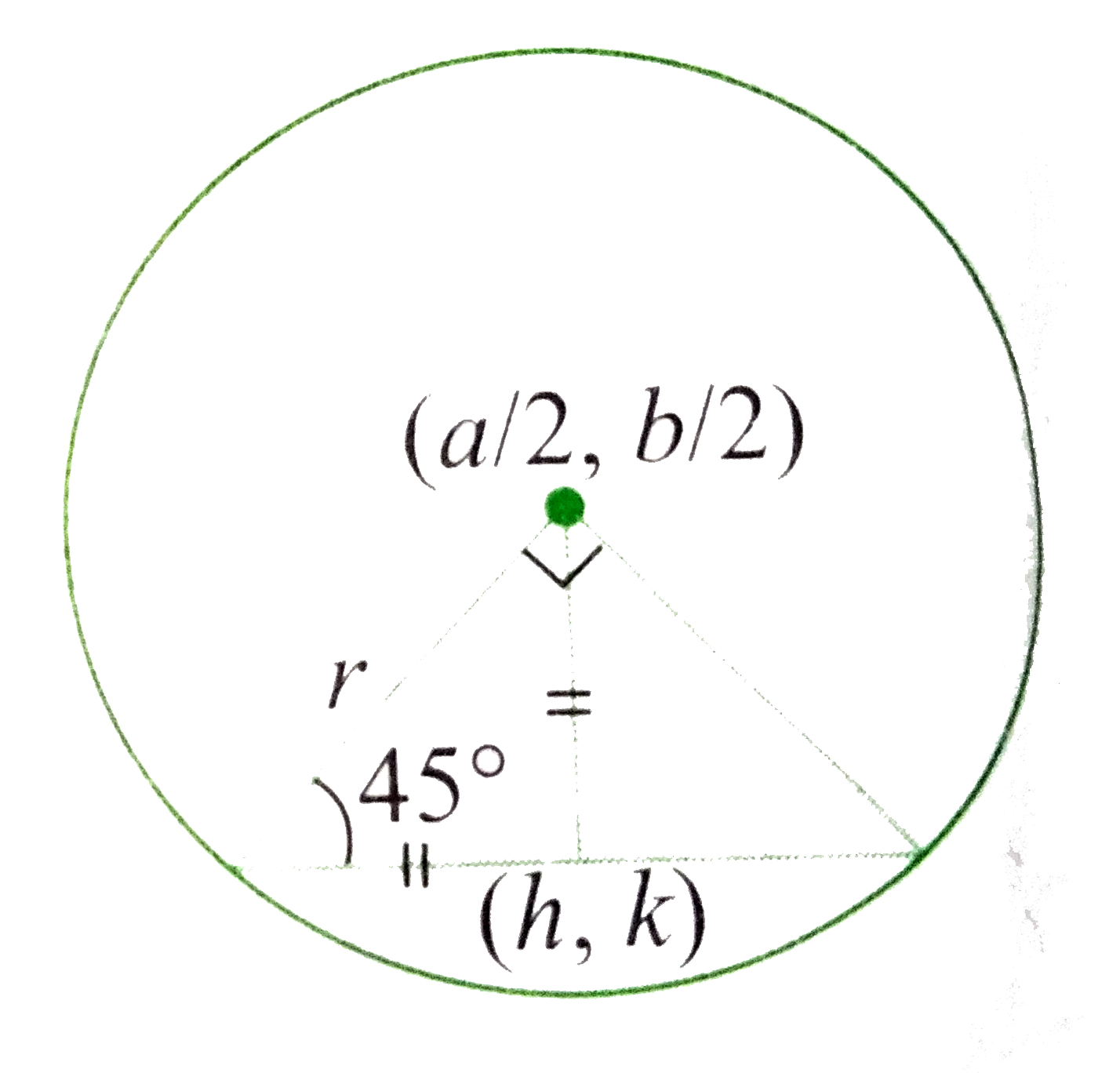
The locus of the midpoints of the chords of the circle $x^2+y^2-ax-by=0$
which subtend a right angle at $(\frac{a}{2},\frac{b}{2})$
is
(a) $ax+by=0$

(b) $ax+by=a^2=b^2$

(c) $x^2+y^2-ax-by+\frac{a^2+b^2}{8}=0$

(d) $x^2+y^2-ax-by-\frac{a^2+b^2}{8}=0$

Options

A. `ax+by=0`
B. `ax+by=a^(2)=b^(2)`
C. `x^(2)+y^(2)-ax-by+(a^(2)+b^(2))/(8)=0`
D. `x^(2)+y^(2)-ax-by-(a^(2)+b^(2))/(8)=0`

Answer

3

Explanation

`r=sqrt((a^(2))/(4)+(b^(2))/(4))=(sqrt(a^(2)+b^(2)))/(2)`
`sin 45^(@)=(sqrt((h-(a)/(2))^(2)+(h-(b)/(2))^(2)))/(sqrt(a^(2)+b^(2))//2)`
or `(1)/(2) =4[(((2h-a)^(2))/(4)+((2k-b)^(2))/(4))/(a^(2)+b^(2))]`
Simplify to get locus `x^(2)+y^(2)-ax-by-(a^(2)+b^(2))/(8)=0`