Find the equation of the tangent at the endpoints of the diameter of circle which is inclined at an angle with the positive x-axis.Solution in Tamil
Diameter makes an angle with x-axis.
So, the slope of diameter is
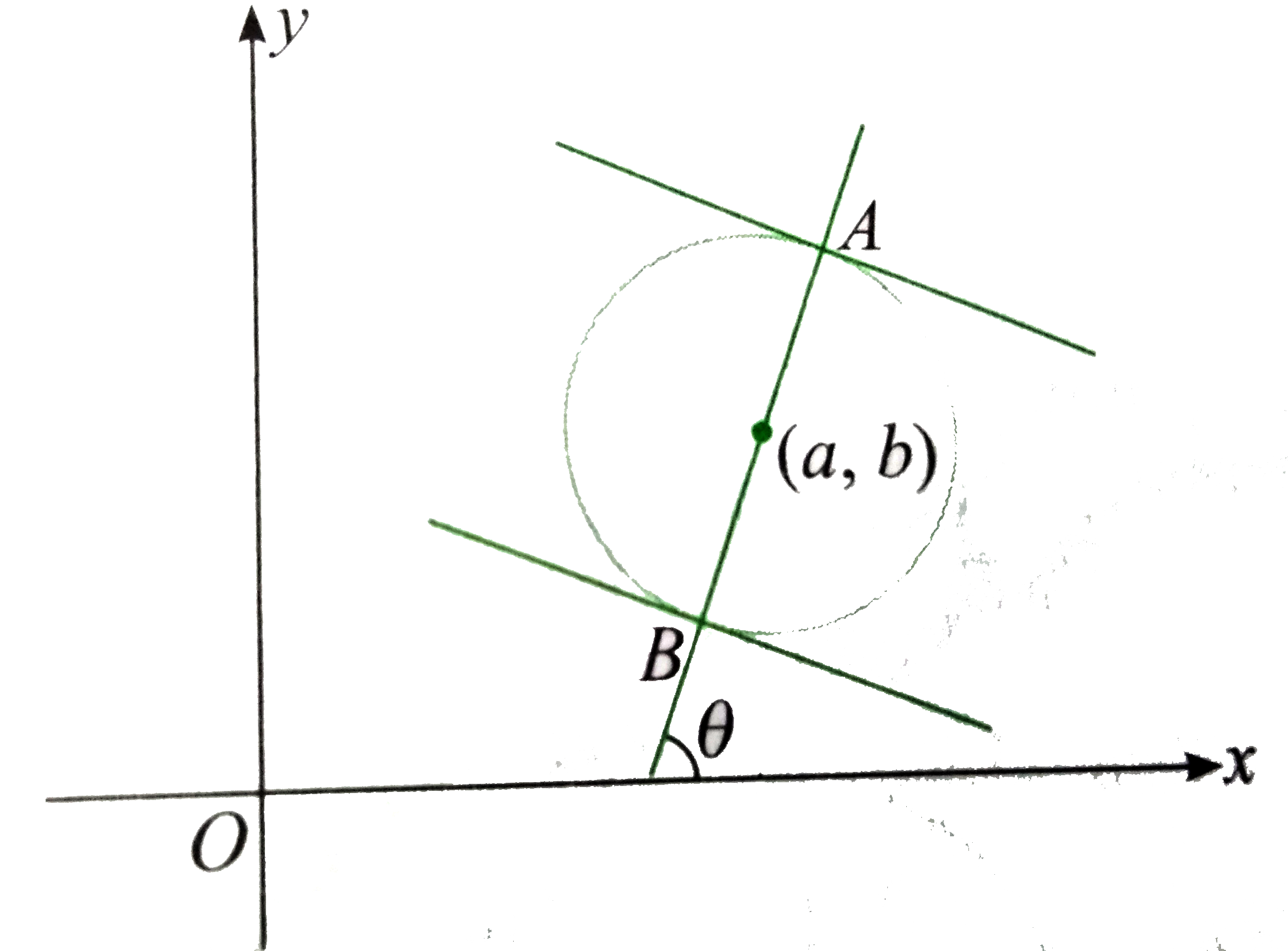
Let this diameter meet the circle at points A and B.
Thus, the coordinates of A and B are given by
Therefore, equations of tangents at points A and B are given by
or
or
Topper's Solved these Questions
Similar Questions
If a circle whose center is (1,−3) touches the line 3x−4y−5=0 , then find its radius.
View SolutionFind the radius of the circle (x−5)(x−1)+(y−7)(y−4)=0 .
View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is
View SolutionIf ax+by=10 is the chord of minimum length of the circle (x−10)2+(y−20)2=729 and the chord passes through (5,15) then the value of (4a+2b) is
View SolutionFind the equation of the tangent at the endpoints of the diameter of circle (x−a)2+(y−b)2=r2 which is inclined at an angle θ with the positive x-axis.
View SolutionFind the equation of the tangents to the circle x^(2)+y^(2)=4 which make an angle of 60^(@) with the x -axis
View SolutionFind the equation of the tangent to the circle x^(2)+y^(2)=25 which is inclined at 60^(@) angle with x -axis
View SolutionThe points of contact of tangents to the circle x2+y2=25 which are inclined at an angle of 30∘ to the x-axis are
View SolutionEquation of the tangent to the circle x2+y2=3, which is inclined at 60∘ with the x-axis is
View SolutionThe equation of the tangent to the circle x2+y2=16 which are inclined at an angle of 60∘ to the x-axis is
View SolutionFind the equation of the tangent to the parabola y2=8x which is inclined at an angl 45∘ with the x-axis.
View SolutionEquation of the tangent to the circle x2+y2=3, which is inclined at 60∘ with the x-axis is
View SolutionFind the equation of the tangent at the endpoints of the diameter of circle (x−a)2+(y−b)2=r2 which is inclined at an angle θ with the positive x-axis.
View SolutionA tangent is drawn to parabola y2=8x which makes angle θ with positive direction of x-axis. The equation of tangent is
View SolutionFind the equation of the tangent at the endpoints of the diameter of circle (x−a)2+(y−b)2=r2 which is inclined at an angle θ with the positive x-axis.
View SolutionThe equations of the tangents to the circle x2+y2=36, which are inclined at an angle of 45∘ to the X-axis are
View Solution
