Find the area bounded by
(i) y=loge|x|andy=0
(ii) y=|loge|x||andy=0Solution in Tamil
(i) y=loge|x|andy=0
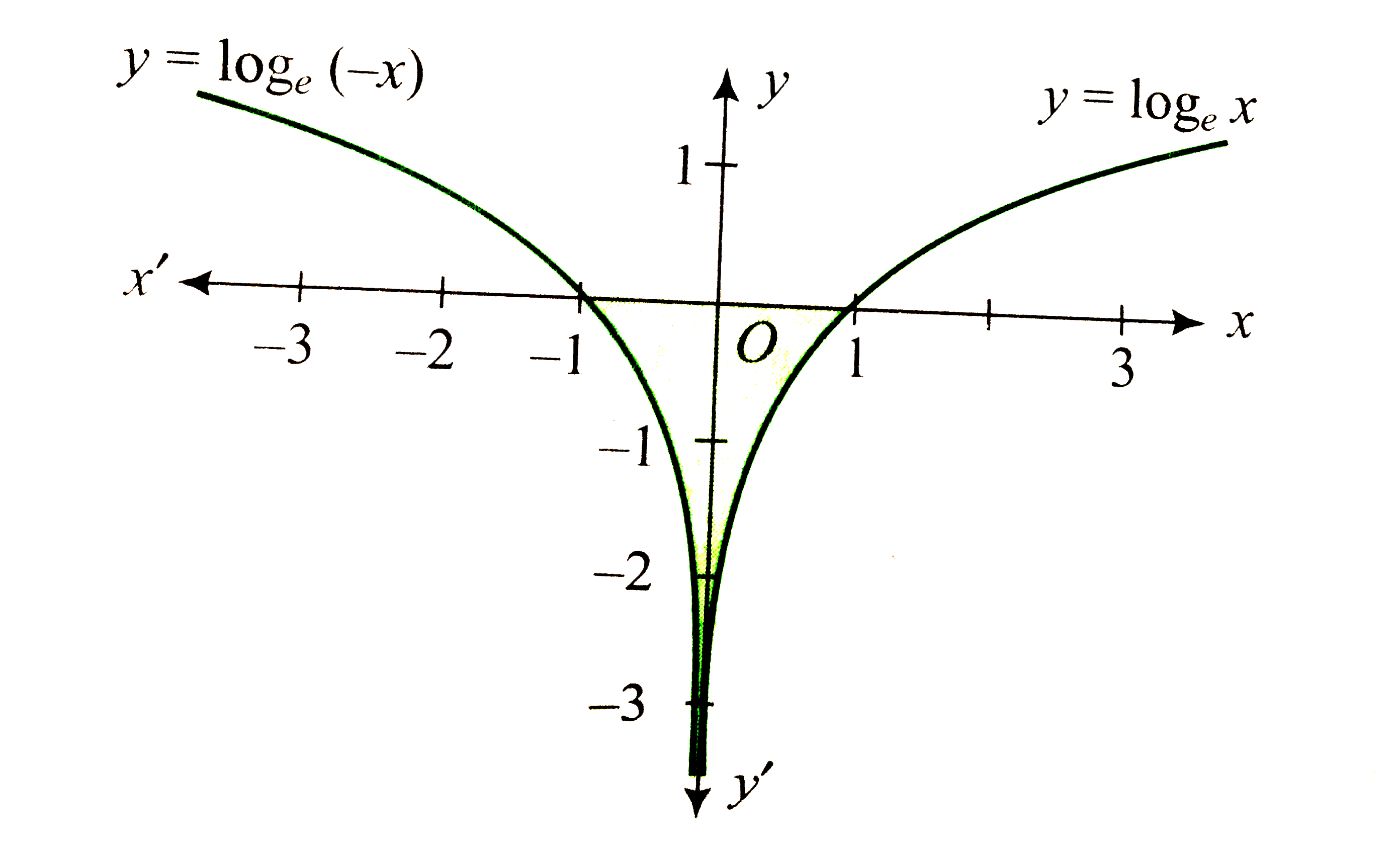
From the figure, required area = area of the shaded region = 1 + 1 =2 sq. units (as we know that area bounded by y=logex,x=0andy=0 is 1 sq. units)
(ii) y=|loge|x||andy=0
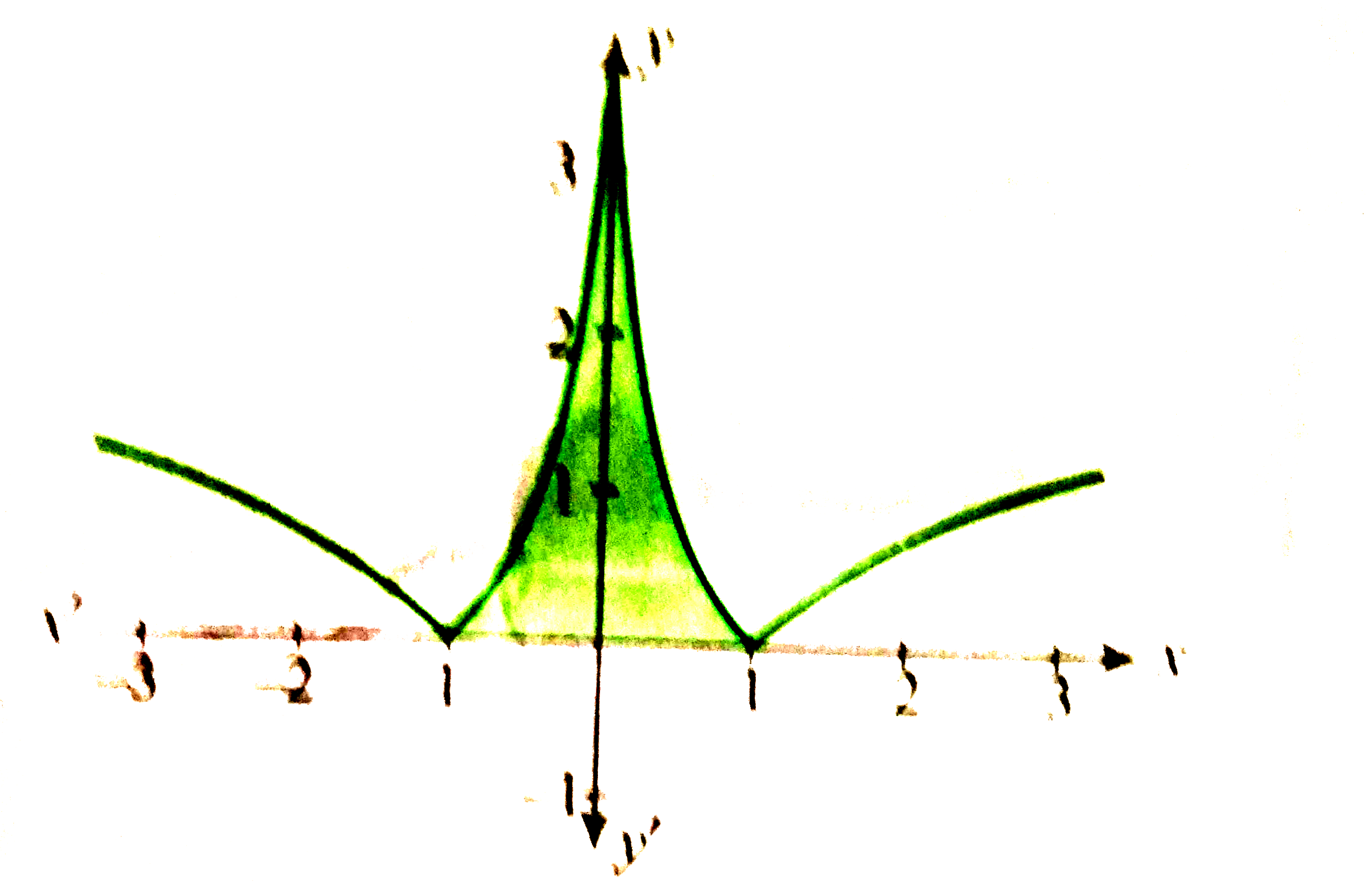
From the figure,
Required area = Area of the shaded region
= 1 + 1 =2 sq. units.
Topper's Solved these Questions
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise 9.1
9 Videos
- AREABOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise 9.2
14 Videos
- APPLICATIONS OF DERIVATIVESBOOK - CENGAGECHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Subjective Type
2 Videos
- AREA UNDER CURVESBOOK - CENGAGECHAPTER - AREA UNDER CURVESEXERCISE - Question Bank
10 Videos
Similar Questions
Find the area of the smaller part of the circle x2+y2=a2 cut off by the line x=a√2.
View SolutionFind the area lying in the first quadrant and bounded by the curve y=x3 and the line y=4x.
View SolutionProve that for λ>1, the equation xlogx+x=λ has least one solution in [1,λ].
View SolutionThe area of the region (s) enclosed by the curves y=x2 and y=√|x| is
View SolutionIf xandy are real numbers such that 2log(2y−3x)=logx+logy ,then find xy.
View SolutionFind the area bounded by a . y=(log)e|x|andy=0 b y=∣∣(log)3∣∣x∣∣andy=0
View SolutionCompute the area of the region bounded by the curves y=ex(log)exandy=logxex
View SolutionFind the area bounded by y=logex,y=−logex,y=loge(−x),andy=−loge(−x).
View SolutionThe area bounded by the curve y=ln(x) and the lines y=0,y=ln(3) and x=0 is equal to
View SolutionFind the area bounded by
(i) y=loge|x|andy=0
(ii) y=|loge|x||andy=0
View SolutionIf y=x3logex, then find y''andy'''.
View SolutionIf x log xdydx+y=logx2 and y(e)=0 , then
y(e2)=
View SolutionIf xlogxdydx+y=logx2andy(e)=0, then y(e2)=
View SolutionStatement-I : Area bounded by y =ex ,y=0 and x=0 is 1 square units
Statement -II: Area bounded by y=logex,x=0andy=0 is 1 square units
View Solutionवक्रों y=ex,y=logex तथा रेखाओं x = 1, x = 2 द्वारा परिबद्ध क्षेत्र का क्षेत्रफल है :
View SolutionArea of the region bounded by y=|x|andy=2 is
View Solution
