Question

PQ is a vertical tower having P as the foot. A,B,C
are three points in the horizontal plane through P. The angles of elevation
of Q from A,B,C are equal and each is equal to ${\displaystyle \theta }$
. The sides of the triangle ABC are a,b,c, and area of the triangle ABC
is del${\displaystyle }$
. Then prove that the height of the tower is (abc) ${\displaystyle \frac{\tan \theta }{4}\stackrel{.}{}}$

Explanation

Let the height of the tower PQ be h.

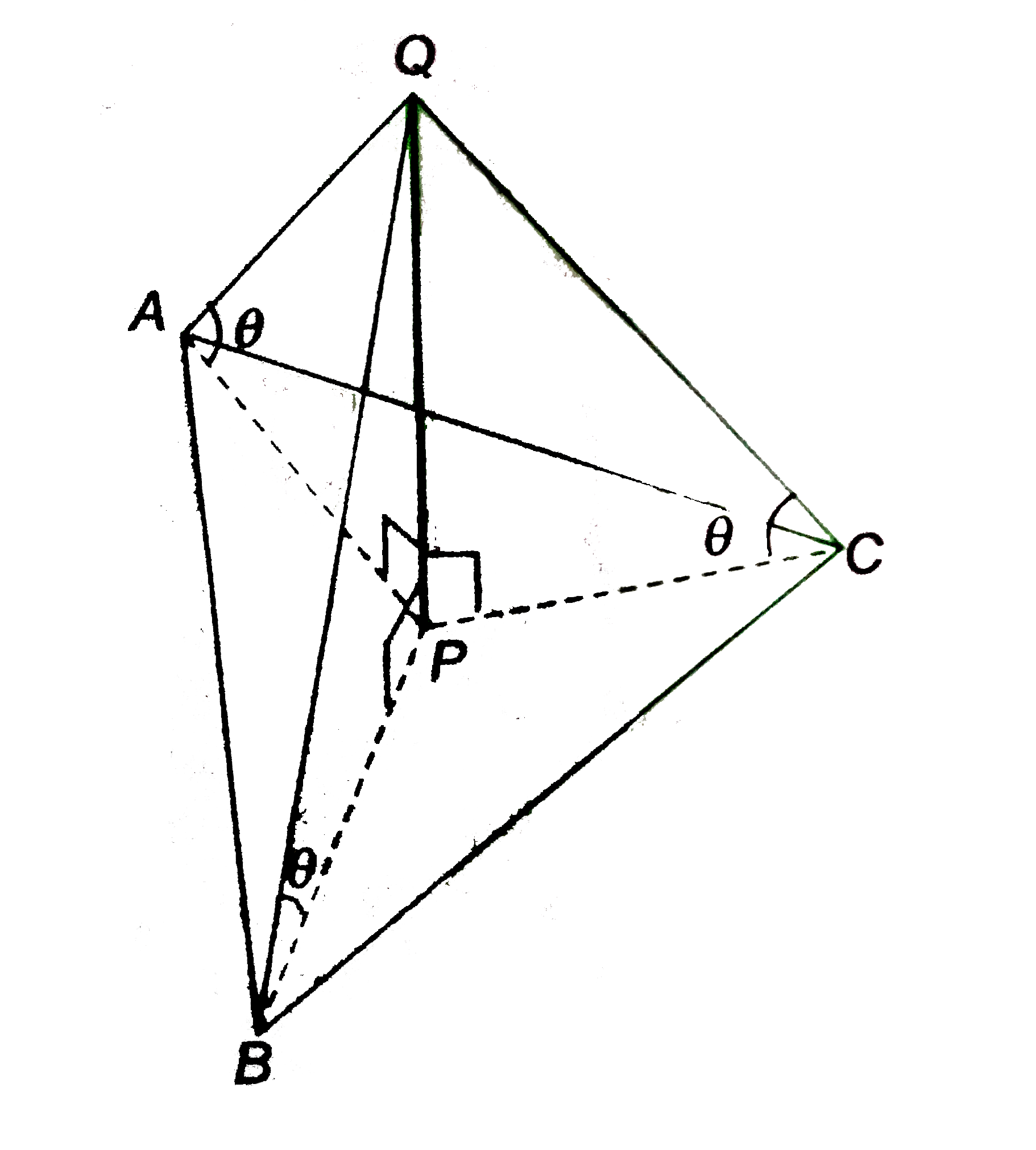
AP = BP =CP =`h cot theta`
Therefore , P is the circumcentre of `DeltaABC`
`therefore AP=BP =CP = R = (abc)/(4Delta)`
`therefore h= (abc)/(4Delta)tantheta`