For a normal distribution if the mean is M, mode is M0 and median is Md, then Solution in Tamil
M>Md>M0
M<Md<M0
M=MdM0
M=Md=M0
The correct Answer is:D
It is obvious.
Topper's Solved these Questions
- STATISTICSBOOK - CENGAGECHAPTER - STATISTICSEXERCISE - JEE Previous Year
10 Videos
- STATISTICSBOOK - CENGAGECHAPTER - STATISTICSEXERCISE - Exercise 11.2
6 Videos
- SOLUTIONS AND PROPERTIES OF TRIANGLE BOOK - CENGAGECHAPTER - SOLUTIONS AND PROPERTIES OF TRIANGLE EXERCISE - Comprehension Type
6 Videos
- STRAIGHT LINEBOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
Similar Questions
If the mean deviation of number 1,1+d, 1+2d,…., 1+100d from their mean is 255, then d is equal to
View SolutionIn an experiment with 15 observation on x, the following results were available. ∑x2=2830,∑x=170 One observation 20 was found to be wrong and was replaced by the correct value 30. Then the corrected variance is
View SolutionLet ABC be a triangle in which the line joining the circumecentre and incentre is parallel to base BC of the triangle. Then answer the following questions :
Then range of ∠A is
View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionFor a frequency distribution, mean, median and mode are connected by the relation (a) Mode = 3 Mean – 2 median (b) Mode = 2 Median – 3 Mean (c) Mode = 3 Median – 2 Mean (d) Mode = 3 Median + 2 Mean
View SolutionThe relationship between mean, median and mode for a moderately skewed distribution is (a) Mode = 2 Median – 3 Mean (b) Mode = Median – 2 Mean (c) Mode = 2 Median – Mean (d) Mode = 3 Median – 2 mean
View SolutionFor a normal distribution if the mean is M, mode is M0 and median is Md, then
View SolutionThe mean and the median of a distribution are 45.9 and 46 respectively. The mode will be
View SolutionThe relation between mean, median, and mode for a frequency distribution is
View SolutionFor a normal distribution if the mean is M, mode is M0 and median is Md, then
View SolutionFor a frequency distribution, mean, median and mode are connected by the relation (a) Mode = 3 Mean – 2 median (b) Mode = 2 Median – 3 Mean (c) Mode = 3 Median – 2 Mean (d) Mode = 3 Median + 2 Mean
View SolutionThe relationship between mean, median and mode for a moderately skewed distribution is (a) Mode = 2 Median – 3 Mean (b) Mode = Median – 2 Mean (c) Mode = 2 Median – Mean (d) Mode = 3 Median – 2 mean
View SolutionWrite the relationship between mean, median and mode of a normal distribution.
View SolutionCalculate the mean, the median and the mode of the following distribution:
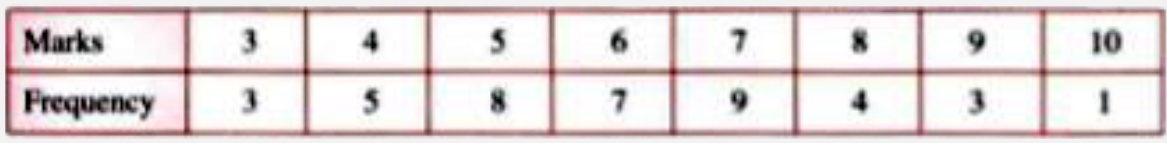
View SolutionFor a frequency distribution, mean, median and mode are connected by the relation . . . . .
View Solution
